The Axially Symmetric Wind Magnetosphere

Extract

A central question in magnetospheric theory of pulsars is: how does a rapidly rotating magnetized neutron star manage to short-circuit its huge unipolar induction voltage? This problem is best studied in an idealized geometry like the parallel rotator. The theory must at least include basic plasma physics with relativistic particle inertia. Even the simplest model will require a global self-consistent solution of both Maxwell's equations and relativistic MHD-equations of motion. Assuming time independence, axial symmetry and charge separation, we reduce this problem to three coupled, quasilinear second order partial differential equations for three dimensionless scalar quantities Φ, f and Γ (see Schmalz et al., 1980): 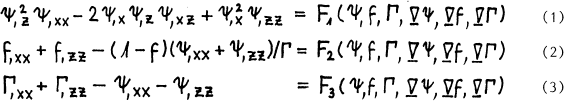Here x and z are cylindrical coordinates measured in units of the light cylinder radius. Φ is the stream function which is constant on stream lines, f = x · vφ/C is connected with the angular momentum (vφ = toroidal velocity), and Γ = 2∊γ = 2∊/(1 - v²/c²)^1/2 where ∊ = mc²/(eB_oa²ω) is a dimensionless parameter. Taking electrons (mass m, charge e) and typical neutron star parameters (radius a = 10 km, angular velocity ω = 30/s, polar field strength B_o = 10⁸ T), we have ∊ ≈ −10⁻¹².