Dead or “undead”? The curious and untidy history of Volta’s concept of “contact potential”

Argument

Much of the long controversy concerning the workings of electric batteries revolved around the concept of the contact potential (especially between different types of metals), originated by Alessandro Volta in the late eighteenth century. Although Volta’s original theory of batteries has been thoroughly rejected and most discussions in today’s electrochemistry hardly ever mention the contact potential, the concept has made repeated comebacks through the years, and has by no means completely disappeared. In this paper, I describe four salient foci of its revivals: dry piles, thermocouples, quadrant electrometers, and vacuum phenomena. I also show how the contact potential has maintained its presence in some cogent modern scientific literature. Why has the death of the Voltaic contact potential been such an untidy affair? I suggest that this is because the concept has displayed significant meaning and utility in various experimental and theoretical contexts, but has never been successfully given a simple, unified account. Considering that situation, I also suggest that it would make sense to preserve and develop it as a multifarious concept.

Volta’s concept of contact potential and its demise

When Alessandro Volta invented the “pile” (battery) at the turn of the nineteenth century (see figure 1), his understanding of its mechanism was based on the concept of the contact potential: the contact between two different metals (zinc and silver in his original apparatus) created a “tension” that drove the electrical fluid in one direction, the wet layers (paper soaked in salt water) between metallic pairs merely serving the role of conducting the electricity (Volta 1800). (Or, if one believed in the existence of two electrical fluids, positive and negative, the tension drove the two electrical fluids in opposite directions.) Volta’s view, the so-called “contact theory” of battery action, was soon challenged by the “chemical theory.” According to the latter view, the flow of electricity was generated from the chemical reaction between one of the metals (zinc in Volta’s original design) and the electrolyte layer, and the other metal (silver) merely served to conduct the electricity (see, e.g., Wollaston 1801). These opposing theories of how batteries work were the source of a long-running contention throughout the nineteenth century. ¹

Figure 1. Alessandro Volta’s “pile,” the original battery, from Volta (1800). “Z” indicates a plate of zinc, and “A” silver.

Today the study of batteries belongs squarely in the realm of electrochemistry, and within electrochemistry the Voltaic contact potential is hardly ever mentioned. A century ago, Irving Langmuir was already lamenting this situation, noting that “As a general rule, since Ostwald’s and Nernst’s work (1889–1893) on the potentials of electrolytic cells, electrochemists have denied the very existence of contact potentials as an intrinsic property of metals, whereas physicists have been divided in their opinions” ([1916] 1961, 175). In a recent survey of the treatment of electrolysis in general chemistry textbooks for secondary schools and lower-level university courses (Chang et al. 2020), we found only one textbook that discusses the contact potential (Levine 2002, 413), and even that one does not actually employ the concept in an explanation of electrochemical cells. More advanced texts in electrochemistry are not much better in this regard. Even in the exhaustive treatment given in the classic textbook of electrochemistry by John Bockris and Amulya Reddy, the discussions of contact potentials and work functions are brief, and they are certainly not noted as main factors behind the power of batteries (Bockris and Reddy 1970, e.g., 647, 942). One great exception here is the modern textbook by Vladimir Sergeevich Bagotsky, which contains a full chapter on “Electron Work Functions and Volta Potentials” (Bagotsky 2006, ch. 9). Whether this may be an indication of a different kind of tradition in Soviet and Russian electrochemistry, I cannot tell.

The history of how we got to this point is not simple at all. The contact potential seems to have been discredited in the course of the ascendancy of the chemical theory, yet it has made repeated “comebacks,” which extend even into the twentieth century, and has never disappeared entirely. In this paper, I will describe four salient foci of the survival and revival of the contact potential: (1) dry piles, namely Voltaic piles without wet elements; (2) thermocouples, which generate a flow of electricity in a bimetallic loop due to a difference of contact potentials at different temperatures; (3) William Thomson’s investigation of contact potentials by means of the sensitive electrometers that he invented; and (4) vacuum phenomena, including the photoelectric effect and thermionic emissions. After describing these foci, I will conclude with some reflections on why the “death” of the Voltaic contact potential has been such an untidy and protracted affair.

The Voltaic tradition

Volta’s own path to the invention of the “pile” has been treated extensively by historians of science over the years (see, e.g., Heilbron 1978; Pancaldi 2003; Pera 1992), so I will not try to cover that ground again here. What is particularly worth emphasizing and remembering is the fact that Volta was not working with electric currents and circuits as we now understand them, which only came into existence as a result of his own invention and its later development and re-interpretation. As John Heilbron says, Volta’s pile was “the last great discovery with the instruments, concepts, and methods of the eighteenth-century electricians” — that is to say, Volta was engaged primarily in operations of charging up objects, releasing the accumulated charge, and observing the effects of that discharge (1979, 491).

Volta’s thinking was driven by the impressions left from a classic experiment that he had performed long before he invented the pile, which continued to impress generations of researchers throughout the nineteenth and into the twentieth century. What some later commentators called the “Volta effect” was a very simple phenomenon: Volta put pieces of two different metals into contact with each other, and then separated them. After separation, he found that electric charge had accumulated on the metallic pieces, with opposite signs from each other. Volta had no trouble detecting this effect with an electrometer, but it was a feeble effect. The Voltaic pile was a way of multiplying the Volta effect, by literally making a pile of bimetallic cells.

The key to Volta’s understanding of these phenomena was his concept of “tension,” which corresponds roughly to the modern concept of potential, or “voltage” (rightly named after Volta himself). This was the original foundation of the notion of contact potential, which is the main subject of our discussion here. Volta’s tension was a rather hydraulic notion, as Heilbron (1978, 43–48) explains. The material conception of electrical fluid easily led to the notion of the quantity (Q) of electricity, or electric charge, that a body holds. If more electrical fluid is stuffed into the same space, it must become more compressed. This “degree of compression” is what Cavendish and Volta called “tension” (T). We thus have a relation Q = CT, for a given body, where C is the capacity of the body for holding the electrical fluid, which depends on its size and other properties. In other words, tension was an indication of how strongly a body was charged up, and that mode of thinking applied to batteries as well.

This concept of tension was quite analogous to the concept of temperature under the caloric theory of heat. As the caloric fluid was seen to move from a place of higher temperature to a place of lower temperature, the electrical fluid was seen to be pushed from a body of higher tension to one of lower tension. Between two bodies at the same tension there would be no movement of the electrical fluid.

For those seeking to understand batteries in Volta’s tradition of electrical science, the most important instrument was the electrometer, which was said to measure tension. Old-fashioned electrometers work by being charged up through contact with the charged body being examined. An electrometer has two light moveable parts hanging down together (e.g., thin gold-leaves, or pieces of straw, as in one type invented by Volta), which diverge from mutual repulsion when the whole instrument is charged up. But how does such detection of charge measure tension or potential?

None of this makes sense without the old idea of electrical “capacity” (C), as described above. Consider the situation in which a charged body is put into contact with the electrometer. The charge will distribute itself over the body and the electrometer, until equilibrium is reached (i.e., until the body and the electrometer are at the same tension T). The electrometer will hold the amount of charge proportional to this tension, and that amount of charge will determine how far the moveable parts diverge from each other.

In Volta’s tradition, the default move in investigating the details of what was happening in a battery and a circuit was to take an electrometer to various parts of the setup to see whether they were electrostatically charged, and how strongly. This impulse was present for a long time, and the talk of whether electrodes are positively or negatively charged pops up even in the midst of many discussions not framed primarily in Volta’s terms. Recognizing this point of view helps us make sense of the motivation behind other work, such as Jean André de Luc’s (1810) now-forgotten “dissection” of the Voltaic pile, in which he endeavored to ascertain which parts of the pile were charged and with how much positive or negative electricity (see Pancaldi 2011, 269).

Focal point #1: Dry piles

Having outlined the initial establishment of Volta’s way of understanding the workings of batteries, I now want to discuss the focal points around which it underwent further developments, beyond Volta’s own experiments and conceptions, for many years to follow. These points also served as anchors for the survival and revival of the Voltaic contact potential as a concept and as an experimental reality.

The iconic apparatus for the followers of the contact theory, especially in the context of the disputes against the chemical theory of the battery, was actually not the Voltaic pile itself, but the “dry pile”—namely any variety of a Voltaic pile made with dry materials between the bimetallic cells, instead of Volta’s electrolyte-soaked wet layers. The Voltaic pile was readily interpretable according to the chemical theory, but there was no easy chemical explanation of how the dry pile was able to generate electricity. The invention of the dry pile is an event somewhat difficult to pinpoint, as it seems to have happened quite naturally by accident, and on various occasions, when Voltaic piles were left to dry and it was noticed that some electrical activity still remained. As Willem Hackmann (2001, 105, 113) reports, the first person on record as having constructed a dry pile deliberately is Johann Wilhelm Ritter, who in 1802 published a description of a dry pile consisting of 600 cells of zinc, copper and leather. Ritter’s dry pile could produce the same degree of electricity as an ordinary pile, but the charging took much longer—up to 20 minutes for the same level of charging that a wet pile could achieve through a momentary contact (see Ostwald [1896] 1980, 347/G360 ² ). Many different designs for dry piles were proposed by various researchers, and A. F. Dyckhoff in 1804 even made a pile that had nothing between the bimetallic cells except air. Volta himself had predicted that it might be possible to make a pile consisting entirely of solids, if “a solid conductor without any power of excitation” could be discovered (ibid., 138/G141), and in 1803 he reported an observation of a dried-up pile that was still active (see Hackmann 2001, 113).

Dry piles of various configurations were a subject of great experimental and theoretical dispute, made famous in the 1810s by De Luc and Giuseppe Zamboni (see Hackmann 2001; Pancaldi 2011; Ostwald 1980 [1896], 346–353/G359–G366; Partington 1964, 16–17). They did not seem obviously chemical: no one could generate chemical effects from them, and the metals in dry piles did not seem to suffer any chemical changes, either. This delighted De Luc, for whom it was important to separate out the chemical and the electrical effects of the pile, as Giuliano Pancaldi points out (2011, 267, 272). Zamboni reported that, even after twenty-four years of operation, the metals in his dry pile showed no sign of tarnish (Hackmann 2001, 114). Summing up what was known by 1814, George John Singer noted: “It therefore appears indispensably necessary to the chemical power of the Voltaic apparatus, that a liquid be interposed between each pair of its plates, whilst for the pure electrical effects, the only condition appears to be the association of the two metals; and the connexion of the different pairs, by some conductor that does not interfere with the electro-motive power” (1814, 462; also quoted in Hackmann 2001, 112).

In Volta’s terms, dry piles generate a high tension but a very small amount of electric charge in motion. It therefore makes sense that they can be felt (literally) but cannot generate a sufficient amount of chemical change to be noticeable. That is to say, the effects generated by dry piles were very much like those of the old frictional-electricity generators; concerning the latter, no one claimed any relevance of chemistry in how electric charge was generated.

Dry piles served as a very clear demonstration of the reality of Voltaic contact potential at the level of phenomena, but their principle of operation remained obscure. After much debate and experimental work, agreement was reached that the operation of the dry pile did require the presence of moisture in the allegedly dry layers, most likely absorbed from the air, even if the experimenters had dried the materials with the utmost care. Ritter had already noted the role of moisture: “The cardboard, leather, linen, etc. import efficiency to the pile only to the extent that they are damp. But the experiment again shows how very small is the proportion of moisture necessary for the pile to perform efficiently” (Ritter, quoted in Ostwald [1896] 1980, 348/G360). However, contrary to the wishes of the advocates of the chemical theory of batteries, there was never a conclusive agreement on whether the role of the moisture was to make the dry layers conductive or to generate electricity by facilitating chemical reactions.

Dry piles are real, and they have never disappeared entirely. The Clarendon Laboratory of the Department of Physics at the University of Oxford boasts a two-dry-pile arrangement that has been ringing a bell nearly continuously since 1840! The departmental website estimates that “so far the bells have been rung of the order of 10 billion times” (Department of Physics 2022). ³ A. J. Croft, who reported on this remarkable instrument nearly forty years ago now, noted: “what the piles are made of is not known with certainty” (Croft 1984, 193). He also reported that “a considerable number” of dry piles inspired by this instrument were made for military purposes during the Second World War by the Oxford physicist A. Elliott. The bell-ringing arrangement was a fairly common setup, going ultimately back to De Luc and B. M. Foster around 1810 (see Pancaldi 2011, 273–4).

There are still dry-pile enthusiasts today, who will eagerly tell you how to build one in YouTube videos. ⁴ The basic principle can be shown in very simple and quick experiments. For example, putting one’s dry thumb in between two metallic plates generates a healthy (open-circuit) voltage, around 0.5V depending on the circumstance, when copper and zinc are used. This is not such an oddity, considering that the very first dry pile on record (made by Ritter) was made with layers of leather, as noted above. Historians of medicine may also remember the once-fashionable “galvanic tractor,” a tweezer-shaped bi-metallic instrument applied to the skin to administer electricity. This was an invention by the American physician Elisha Perkins (1741–1799), who gained great notoriety for it at the end of the eighteenth century (see Miller 1935). All indications point to the conclusion that dry piles do work, and that they were a source of at least some discomfort to the chemical theorists of the battery.

Focal point #2: Thermocouples

The dry pile was invented almost immediately after Volta’s original invention, and it has led a shadowy existence of under-appreciation for more than 200 years now. The thermocouple, on the other hand, arrived on the scene twenty years later. It generates a flow of electricity in a loop made with two pieces of different metals, whose two junctions are put at different temperatures. This provides a very clear and simple demonstration of the bimetallic contact potential. The phenomenon of thermoelectricity makes perfect sense if one assumes that the contact potential between two metals has different magnitudes at different temperatures. Hackmann (2001, 115) even argues that thermocouples helped push dry piles off the center stage of the debate about the nature of the Voltaic pile: “By the 1830s the dry pile had effectively dropped out of this debate. This was partly because a new startling phenomenon, Seebeck’s ‘thermoelectricity’ and the thermopile, overshadowed it” (the thermopile being an arrangement that connects multiple thermocouples in series to get a stronger voltage).

The invention of the thermocouple, in 1821, was the work of Thomas Johann Seebeck, who initially saw his discovery as the direct conversion of a thermal gradient into magnetic action, as Keld Nielsen (1989; 1991) explains in wonderful detail. In Seebeck’s own words: “two metals alone, connected to form a circuit, might become magnetic without the participation of a moist conductor” (quoted in Nielsen 1991, 378). His interpretation was soon superseded by that proposed by Hans Christian Ørsted, André-Marie Ampère and others, who recognized an electric current flowing due to bimetallic junctions at different temperatures, turning the magnetic needle as a secondary effect caused by the current. But the point that remained from Seebeck’s interpretation is the most important one for the science of batteries: the action happens “without the participation of a moist conductor.”

Now, there are those who will say that the thermocouple is not a battery, because a battery is a device that converts chemical energy into electrical energy. That is the consensus among modern chemists, but as a historiographical framing device this modern understanding is question-begging. If I am trying to tell the complex story of how this modern understanding came to be the dominant view, I should not start by presuming its conclusion. Nahum Kipnis gives some indication that some battery-scientists did consider thermoelectricity as part of their remit. Gustav Theodor Fechner is said to have expressed his dissatisfaction in 1838 about the fact that “[Voltaic] contact phenomena, as well as thermoelectricity, remained unexplained in the chemical theory” (quoted in Kipnis 2001, 138). Antoine-César Becquerel is quoted as saying in 1842 that “thousands of experiments have proven that there are no electrical effects of contact unless there is a chemical or calorific action, or any derangement of the natural position of molecular equilibrium” (quoted in Kipnis 2001, 140).

Regardless of the disputes concerning its mechanism, the thermocouple did have a very promising start in its role as a battery. Within about five years of its invention, it was used by Georg Simon Ohm in the experiments that led to the discovery of Ohm’s law (for a general and detailed discussion of Ohm’s work, see Ostwald 1980 [1896] 368–408/G382–G420). In his first experimental attempt to establish the law of conduction, published in 1825 in both Schweigger’s Journal and Poggendorff’s Annalen, Ohm presented a law indicating a logarithmic dependence between the length of conductor and the “loss of force” (der Verlust an Kraft); it is not the Ohm’s law that we are all familiar with today (Ohm 1825, 111, 115; see also Ostwald 1980 [1896], 371/G384). One of the difficulties Ohm experienced and discussed was the fact that the battery he used, an ordinary Voltaic pile in the trough form, employing copper, zinc and dilute sulphuric acid, had a very fickle and unsteady output. In response, Johann Christian Poggendorff made the following suggestion, which he put in as a note to the version of Ohm’s paper published in his journal: “It is to be wished that the author could find leisure to undertake this and similar determination of laws with the help of the so-called thermo-electric cell. The effects with thermo-electric cells are much steadier than those for the so-called wet cells. These actions can therefore be measured very accurately” (quoted in Ostwald 1980 [1896], 373, G386). ⁵ Here Poggendorff was clearly taking the thermocouple as an electric cell (battery). It is not too idle to speculate how much more importance as a battery the thermocouple might have had, if John Frederic Daniell had not gone on to invent a steady wet cell within about ten years of the time of Ohm’s work.

Ohm (1826) duly followed Poggendorff’s suggestion, and rigged up the now-iconic instrument combining a torsion-balance galvanometer as the current-measuring device (as before), and a bismuth–copper thermocouple as the power source. As Poggendorff had hoped, this setup yielded much steadier results, which formed the empirical basis of the form of Ohm’s law that became accepted and remains to this day: I = V/(R_i+R_e), where R_i is the internal resistance of the battery and R_e is the external resistance in the rest of the circuit. It is difficult to think of a more significant use of a simple scientific instrument, than a decisive contribution to the discovery and confirmation of Ohm’s law. And Ohm’s contribution, enabled by the use of the thermocouple as a battery, was theoretically even more significant than people now recognize. What he did was not just a matter of finding an empirical regularity holding between well-defined theoretical quantities. The more fundamental part of his work was articulating voltage, current and resistance as well-defined quantitative concepts in the first place. As Ostwald put it:

These concepts were not worked out to the stage of quantitative definition. It was left for Ohm to prove that it is possible not only to talk about these things but also to use them in calculations. Ohm conceived the electroscopic force or the voltage as a driving force for the movement of electricity. He introduced the concept of intensity of current as the quantity of electricity flowing per unit of time. He also conceived the resistance as something independent of the voltage. In a given conductor the resistance determines, besides [together with] the voltage, the strength of the current. In this way Ohm found a form in which the phenomena of the galvanic circuit can be ‘described’ in a very simple manner. (1980 [1896], 393/G405)

The thermocouple was a perfect instantiation of the idea of a contact potential between two different metals, and it provides the most conclusive imaginable argument against an exclusively chemical conception of the battery, even better than the dry pile. It is curious that the contact theorists do not seem to have made much use of the thermocouple as ammunition in their attack on the chemical theorists, though both Seebeck and Ohm have been identified as being on the contact side of the contact–chemical divide. Ohm’s papers do make it clear that his thinking was along the lines of the contact theory. The very titles of his papers specify his subject matter as the conduction of “contact electricity” (Contaktelektricität), which evidently included both electricity driven by a wet Voltaic cell and electricity driven by a thermocouple. One pertinent factor here, which I will come back to later, is that it was not at all straightforward to draw any sensible correlations between the values of the thermo-electric potentials and the voltaic-cell potentials. The thermocouple voltages are generally much smaller than the wet-cell voltages, and a metallic pair that gives the highest thermo-electric voltages does not necessarily give very high wet-cell voltages. ⁶

Focal point #3: Quadrant electrometers

For the next few decades after Seebeck and Ohm, no great advances seem to have been made in the study of contact potentials. Meanwhile the dispute between the chemical and contact theories of batteries continued actively, the balance now tipping strongly in favor of the chemical theory, especially with input from Michael Faraday. All of that was to change, however, around 1860, due initially to the work of William Thomson (later Lord Kelvin), the rising star of British physics and engineering, who was at the time engaged in the work on the cross-Atlantic telegraph line that would soon bring him broader fame. Sungook Hong has recounted the story of this new development in a very insightful and detailed paper. As he sums it up, this phase of history began thus: “In 1862, William Thomson revived the contact theory of voltaic phenomena. Based upon quantitative measurements and a new method of measuring small potential differences, Thomson’s contact theory soon became a new orthodoxy” (Hong 1994, 233). It is notable that the key here was an innovation in the detection and measurement of electricity, which was a very different kind of development from the dry pile and the thermocouple, both of which were new setups for power-generation.

There was one major difficulty with contact potential phenomena not also involving chemical reactions, starting with Volta’s classic charging-by-contact experiment (the “Volta effect”): the experimental outcome was feeble and difficult to measure reliably. What Thomson did in order to revitalize this area of study was, first and foremost, to eliminate this difficulty by inventing sensitive and reliable new electrometers. His key innovation was the use of a charged needle, suspended horizontally so that it could rotate, whose tip was placed in or just above the gap between two pieces of metal lying next to each other. This needle would be pulled and pushed very sensitively from the two metallic pieces, depending on the sign and amount of charge held by them.

Thomson developed this idea into his famous “quadrant electrometer” (see Hong 1994, 282–285; Gordon 1880, vol. 1, 35–37). The “quadrant” part of this instrument consisted of a metallic disc divided into four pieces; the diagonal pieces were connected with each other by wires, so that they would be at the same potential (see figure 2). The charged needle, made of aluminium for lightness, was hung from a torsion wire above the disc, ⁷ so that its angle of rotation from the equilibrium point indicated the strength of electrostatic attraction and repulsion from the diagonally connected pairs of quadrants. The diagonal connections were designed so that the electrostatic action on the two halves of the needle would add up rather than cancel each other.

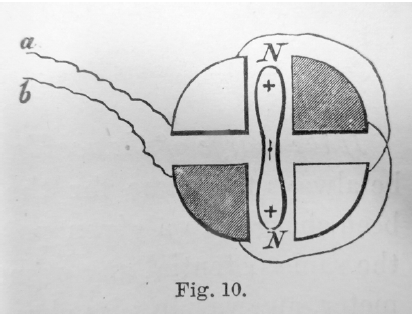
Figure 2. A diagram illustrating the core part of the Thomson quadrant electrometer, from Gordon (1880), vol. 1, 35.

As Thomson’s young contemporary James Edward Henry Gordon nicely explained, this instrument could be employed in two different ways (Gordon 1880, vol. 1, 36–37). First, one pair of the quadrants could be earthed, and the other pair charged up from a source of electricity at a certain potential. The higher the potential, the more charge would be given to the electrometer pieces, and the stronger attraction or repulsion of the needle that would be caused. This meant that there was a clear correlation between the degree of deflection of the needle and the applied potential. Second, using the “zero method,” the quadrant electrometer could be used to compare the potential levels of two other bodies. In that case, the two bodies in question would be connected to the two quadrant-pairs, and the needle would show no deflection if the two potentials were equal.

The excellence of Thomson’s quadrant electrometer was such that the facts established by it were virtually undisputed. One of the first things that Thomson did in this line of work was to demonstrate the reality of the Volta effect and measure the magnitude of the contact potential involved in it. Having started the work in 1858, he gave a brief description of his key experiment in a brief publication from 1862. He seems to have held to the original account of this experiment to the end of his life, demonstrating it at a Friday Evening Discourse at the Royal Institution in 1897; on that occasion he also published a more detailed description of the experiment (Thomson 1898; see Lichtenstein 1978 for a modern reproduction). After Thomson’s work, contact potential was an accepted fact, and the only point of dispute was the interpretation of its causes.

The results from Thomson’s measurement of contact potentials (e.g. 0.75V between zinc and copper), “strongly implied that it was nearly equal to a cell’s EMF [electromotive force]” (Hong 1994, 242). This led Thomson and others to suspect that the bimetallic contact potential was, after all, the main source of the power of a Voltaic cell. Thomson therefore declared that “the proper explanation of voltaic action in the common voltaic arrangement [battery] is very near Volta’s” (Thomson 1862, 177). Having thus located the “seat of the EMF” of a typical Voltaic cell in the metal–metal contact, Thomson then reasoned that the role of the electrolyte in the battery must be only to allow the current to flow by completing the circuit with a non-metallic contact, rather than exert an EMF of its own (ibid.). Without the electrolyte connecting the metals at the other end, the bimetallic contact EMF quickly results in the accumulation of opposite charges on the metal pieces, whose electrostatic forces then set up a counter-EMF that prevents any further movement of electricity.

After some further consideration, however, it was easily admitted that not only bimetallic contact, but any contact between two materials had the capacity to generate an EMF. This was in fact what had happened with Volta’s original theory as well, as Volta and many of his followers had quickly made the same acknowledgment of contact potential in non-bimetallic contacts. With this broadening of the domain of contact potentials, objections like the one from John Ambrose Fleming pointing out that there were cells without bimetallic contact were easily dealt with by Thomson. ⁸

Even while asserting that the bimetallic contact was the chief source of the EMF in a Voltaic cell, Thomson and his allies could allow that the metal–liquid and liquid–liquid contacts contributed some EMF. Moreover, their responses went beyond mere defensiveness, especially in the work of William E. Ayrton and John Perry, two British scientists working in Tokyo at the time. With the help of a Thomson quadrant electrometer, Ayrton and Perry (1878) measured the metal-liquid contact EMF, which was in the order of 0.1V. Even though there was some room for debate in the interpretation of Ayrton and Perry’s experiments, their results served to boost the credibility of Thomson’s work (see Hong 1994, 244–247). Ayrton and Perry’s paper was intended to provide a full “contact theory of Voltaic action,” founded on the following general notion: “At the surface of contact of two conducting substances there is an electromotive force of definite amount which tends to make electricity flow from one of the substances to the other” (Ayrton and Perry 1878, 198). The same kind of view was expressed by George Chrystal, Professor of Mathematics at the University of Edinburgh, who had studied under Maxwell in Cambridge, in his article on electricity in the ninth edition of the Encyclopaedia Britannica: “Perhaps the most general principle concerning the origin of emf [electromotive force] recognized by physicists of the present day is the following:—When two different substances are in contact, there exists in general an emf at the surface of separation, tending to displace electricity across that surface. This emf is commonly called the ‘emf of contact,’ or simply the ‘contact force’” (Chrystal 1878, 83; emphasis in original). ⁹

After the flurry of interest in the Thomsonian work in the 1860s and 1870s, however, it seems that Voltaic potential ultimately failed to command the attention of a broad community of scientists. This was not so much a matter of right and wrong, but a question of productivity. Even though no one tried seriously any more to deny the reality of contact potentials as demonstrated by Thomson, the Thomsonian work became mired in a complex dispute with James Clerk Maxwell and his followers about where exactly the “seat” of the EMF was, rather than producing further significant new results or ideas. Hong summarizes the heart of the dispute as follows:

For Thomson, metallic contact created voltaic potential difference inside the metals. For Maxwell, it annihilated the potential difference inside the metals. Their differences were intimately connected to different definitions and ways of measuring potential. They were also deeply rooted in Thomson’s and Maxwell’s respective beliefs concerning the nature of electricity and of the conduction current as well as the role of the medium in electrical phenomena. (Hong 1994, 237) ¹⁰

While many physicists occupied themselves energetically with this debate without being able to resolve it clearly, most chemists were not interested. While the Ayrton–Perry account of the Voltaic cell was cogent, it did not lead to new results useful for those working on batteries and electrochemistry. Instead, the exciting new developments happening there in the second half of the nineteenth century consisted on the one hand in the invention of practical new devices, such as the carbon–zinc dry cell and the lead-acid storage battery, and on the other hand in new theoretical developments concerning the physical chemistry of electrolytes, based on thermodynamics and Svante Arrhenius’s new ionic theory.

On the whole, Thomson’s pioneering work established the Voltaic contact potential as an accepted effect in physics, but the physicists’ attention on it did not spread to the field of electrochemistry. To the extent that batteries became more and more the exclusive remit of chemists than physicists, this also meant that the contact potential became irrelevant to battery science. The newly established field of physical chemistry, the umbrella under which electrochemistry was placed in the twentieth century, was fundamentally framed in terms of thermodynamics, in which the contact potential did not find a natural place. Irving Langmuir, whose work I will discuss in more detail in the next section, gave the following retrospective view in 1916: “As a general rule, since Ostwald’s and Nernst’s work (1889–1893) on the potentials of electrolytic cells, electrochemists have denied the very existence of contact potentials as an intrinsic property of metals, whereas physicists have been divided in their opinion” (Langmuir [1916] 1961, 175).

Focal point #4: The physics of electrons

One more major phase of development concerning the Voltaic contact potential was to come, and in close connection with the momentous developments that eventually led to the coming of quantum physics in the early twentieth century. This new phase of work was enabled by the discovery of the electron, and the technological developments that allowed the study of electrons in a vacuum. As Kragh notes in the last part of his overview of the contact/chemical dispute: “About 1915 new life was brought to the half-forgotten contact theory from high-vacuum experiments on thermionic effects, photoelectricity and metal vapours” (Kragh 2000, 153). This is as Irving Langmuir already noted at the time: “Thus the long-standing conflict between the two theories was apparently finally brought to a close by the complete victory of the chemical theory. Within the last four or five years, however, some remarkable progress has been made in certain branches of physics, which has resulted in bringing to life again the contact potential theory” ([1916] 1961, 176–177). Langmuir then listed no fewer than six lines of pertinent new work: “I. Electron emission from heated metals [thermionic emission]. II. Thermal effects accompanying the electron emission. III. Photo-electric effect from metals. IV. Measurements of contact potentials. V. Ionizing potentials of metal vapors. VI. Single line spectra of metal vapors” (ibid.).

The advances noted by Kragh and Langmuir were both theoretical and experimental. Theoretically, it suddenly became possible to make deeper sense of the Voltaic contact potential, in terms of the difference between the work functions of different metals (related to the Fermi level), namely the amount of energy required to liberate a conduction electron from a metallic surface. The importance of such notions was clearly recognized by leading experimentalists as well. Particularly notable in my opinion was Robert Millikan’s work, which offered direct empirical confirmation of Einstein’s equation for the photoelectric effect, through which Millikan also made the first-ever precise determination of Planck’s constant (see Leadstone 1990, 173–174; Kragh 2000, 153; Chang 1997).

Working in near-perfect vacuum not only revealed new electron-related phenomena, but also gave experimenters a chance to remove the speculative objections from the old chemical/contact debate, according to which contact potentials were only the insidious chemical effects of air, or the moisture or oxygen contained in air. There was no chance of that, for instance, in Millikan’s “machine shop in vacuo” (1916, 362), in which he shaved off a metallic cylinder to expose a fresh surface, all inside the vacuum chamber, before he carried out his experiment on the photoelectric effect. These objections had already been weakened by earlier experiments done in various gases without much variation in results, but the experiments in a high vacuum were more decisive (see Hong 1994, 273–274 on the earlier work).

Aside from investigating the photoelectric effect itself and the value of Planck’s constant, Millikan took care to check the correlation between the photoelectric effect and the Volta effect (Chalmers 1942b, 429). In measuring the amount of energy required of a photoelectron to be emitted from metal 1 (source) and received by metal 2 (receiver), Millikan reasoned that the overall potential to be overcome by the electron was the sum of the voltage applied between the two metals and the Volta potential between the two types of metals. Calculations carried out on this basis made from measurements between pairs of three different metals were self-consistent.

But did such work by physicists only end up moving contact potentials further away from the realm of battery science, thus intensifying the outcome mentioned at the end of the last section? Were these physicists just working on some new fancy electronic and solid-state physics, totally unconnected to batteries? That may have been the case with many of them, but certainly not all. Informed by results from these new lines of experiments on the contact potential, several scientists did approach the question of Voltaic batteries again.

The American chemist, physicist and engineer Irving Langmuir was a very notable case. Langmuir went from studying light bulbs to doing pioneering work in surface chemistry (Nobel Prize in Chemistry, 1932). The thermionic emission of electrons was very much in his domain, starting with his focused work on the behavior of tungsten filaments in light bulbs. Hong notes that Langmuir “declared in 1916 that the new experimental data on thermal emission and photoelectric phenomena demonstrated the existence of a large contact potential difference” (1994, 281). His view is that Langmuir was rather re-inventing the wheel, without realizing that this topic had been minutely debated with no clear conclusions reached. I, on the other hand, think that Langmuir was quite well aware of the history and, more to the point, that the twentieth-century experimental work (including his own) that stimulated his thinking was genuinely new, different, and better than what had formed the basis of the wrangling between the Maxwellians and the Thomsonians. I therefore believe that Langmuir’s work deserves closer attention.

The importance of new and improved experiments in highly evacuated spaces was clear to Langmuir. He identified three reasons “that have led to the abandonment of the contact theory of electromotive forces by electrochemists,” and one of them was “irregularities in the Volta effect,” which raised doubts about the reality of the effect, and suspicion that whatever was real in contact potential phenomena was really due to chemical reactions (Langmuir [1916] 1961, 176–177). ¹¹ Langmuir’s view, explained at length and in detail in his 1916 paper, was that new and improved techniques for creating a better vacuum, cleaning metal surfaces and controlling other factors had cleared up these alleged irregularities, revealing contact potential as an intrinsic property of pure metals. In this argument, he noted with approval the work of many experimenters, including Millikan, as well as the work of his own team at the General Electric Company’s research laboratory in Schenectady, New York, where he had worked since 1909. In Langmuir’s mind there was no doubt that contact potential was exhibited by pairs of metals unaffected by chemical reactions due to any impurities sticking (adsorbed) to their surfaces or active substances in the gases surrounding them.

Langmuir also tried to sketch a theoretical view of the phenomena discussed, chiding mainstream electrochemists for only paying attention to the thermodynamics of systems in equilibrium, an approach represented by the Nernst equation and standard electrode potentials. “Such values,” he claimed, “give us little information about the real mechanism of the cells or their operation when current actually flows” (ibid., 215). He saw this “thermodynamic viewpoint in electrochemistry” as a hindrance to further progress in the field, because thermodynamics “tells us practically nothing of the kinetics or mechanism of any process”. We want to know, he argued, “how the individual ions and atoms pass from the electrode into the solution, or vice versa, and must know something about the structure of the double layers which are the cause of the potential differences occurring at the surfaces of the electrodes”. Langmuir suggested that, in order to do better, “it will be essential to consider the mechanism of these processes according to the methods which have had such revolutionary effects in the recent developments in physics”. The new approach should not confine itself to chemistry: “contact potential at the junction between metals is one of the factors which must be taken into account in reaching any understanding of the mechanism of electrochemical action”.

Langmuir offered a unified view of relevant phenomena as much as possible, making use of the concept of different degrees of “electron affinity” that different metals have (see Table IV on Langmuir [1916] 1961, 198, which shows the values of electron affinity estimated through five types of phenomena). The consilience of experimental data suggested that “contact potential, photo-electric effect and thermionic emission, are thus shown to be intimately related to each other”. All three phenomena hinge directly on electron affinity, “a quantitative measure of the work done in separating electrons from the metal”. Langmuir was confident that in each case experimental evidence showed that the core effect was not chemical, though chemical reactions did certainly add other effects. Moreover, the evidence of independence from chemistry concerning one phenomenon also constituted the same for the other phenomena, since they were ultimately the same: “Because of the relationships between the different phenomena, a proof that any one of them is not caused by chemical action must be considered to be proof that none of them is so caused” (ibid., 192; see also 209).

After thus laying out his view of the physics of metals and electrons, Langmuir was ready to present his revived contact theory of electrochemical action. He asked the reader to consider a simple bimetallic setup for a Voltaic cell, as shown in figure 3. This figure represents the configuration of a zinc–copper cell in which the two electrodes are connected by a copper wire, with an as yet unspecified electrolyte to be placed in the space (C–F) between the copper and zinc electrodes. Due to the contact potential, the zinc piece is charged positively, and the copper negatively. If there is no conductor in the space between electrodes, an electric field is set up there, and a charge placed in that space would move to one or the other of the two electrodes.

Figure 3. Illustration of the Voltaic cell from Langmuir [1916] 1961, 209.

Now imagine filling that space with an ionized gas. Positive ions would move to copper, pick up electrons there, and become neutral; negative ions would move to zinc, give up electrons there, and become neutral. At the same time, electrons would move from zinc to copper to maintain the same electrical state of the metals. In other words, the movement of the ions creates an electrical current moving through the bimetallic circuit, and that current will continue if a continual supply of ions can be provided in the gas.

It is here that Langmuir’s key insight comes into play:

It is evident that there is no permanent source of energy at the junction of the metals. But it takes energy to produce ionized gas, and this ionization is destroyed by the flow of the current. It is thus clear that the energy of the current is supplied originally by the ionizing agent. On the other hand, the force which causes the current to flow has nothing to do with the ionization. An electric field exists in the space between the electrodes C and F and therefore the ions move towards the electrodes. It is this field which must be looked upon as the immediate cause of the flow of current. (ibid., 211)

Langmuir argued that the essential feature of the situation was the same if we place in the space between electrodes a liquid electrolyte instead of an ionized gas, thereby making an electrochemical cell (ibid., 211). This time the energy is supplied by the chemical reactions. The situation is also more complicated, because the substances created by the neutralization of the ions of a liquid electrolyte tend to be chemically active and cause various secondary reactions, often reacting with the electrode metals themselves. If the reaction products are not removed, they will cause the formation of double layers at the electrode surfaces. But as with the ionized gas cell, here, too, “The fact that the energy is derived from a chemical reaction does not indicate that the electromotive force of the cell is caused by chemical action” (ibid., 212). To illustrate the distinctness of the considerations of energy and EMF, Langmuir proposed an insightful analogy:

Let us imagine a deep hole in the ground, near the shore of a lake. A pipe conveys water from the lake to a water turbine placed at the bottom of the hole. If the turbine is operated, power is generated, but only for a short time, for the well soon becomes filled with water…. In order to obtain power continuously from the turbine it is necessary to pump the water from the well back to the level of the lake. (ibid.)

The depth of the hole is the measure of the force that runs the turbine, but the action of the pump is what supplies the energy that runs the whole arrangement.

The concept of contact potential also proved to be fruitful in solid-state physics. This can be seen most clearly in the discussion of how asymmetric junctions function. These elements are used as diodes in circuits, which allow the flow of current in one direction but not the other. In the early days they served an important function as “rectifiers,” converting alternating currents into direct currents. As Ernest Braun explains, the invention of the solid-state rectifier is usually credited to the work of Walter Schottky around 1940 (1992, 449–451). Schottky understood the workings of his metal-semiconductor rectifier in terms of the contact potential difference between the two substances (e.g., copper and copper(I) oxide), which is the main factor determining the electron densities near the boundary. Schottky actually understood this as the electrochemical potential, equal to the difference of Fermi levels. It is pertinent to our story that Schottky also had an interest in thermionic emission, and the original diode was indeed the “thermionic valve,” a vacuum-tube arrangement in which one electrode was heated, allowing the emission of electrons, and the other was not. So it seems that the link back to the old debates on the Voltaic contact potential would not have been lost on Schottky, and Braun also notes that Russell Ohl, a pioneer of silicon semiconductors, was trained as an electrochemist (ibid., 462). However, today’s semiconductor physics seems very disconnected from electrochemistry, while discussions of semiconductor junctions routinely invoke the contact potential (see, e.g., Hummel 2011, sec. 8.7.2 and 8.7.4).

A rare contemporary work that notes and laments this disjointedness is the 2016 paper on contact potentials by Pekka Peljo, José Manzanares and Hubert Girault, appropriately published in the journal Langmuir (published by the American Chemical Society), which begins with a perspicuous survey of the history of contact potentials (citing Hong 1994 among other sources). These authors take the Volta effect as an established fact, and recognize its relevance in various areas:

The aim of this article is to clarify the position of the Fermi level after contact charging, to understand how much charge is transferred and where this charge is located…. To understand what happens upon metal–metal contact and how this phenomenon affects for example, electrocatalytic, catalytic, and optical properties of bimetallic nanosystems, we must understand the governing principles. (Peljo et al. 2016, 5765)

But they know that there is a disciplinary boundary here. Part of their intended contribution is to “describe the thermodynamics of the contact electrification from the point of view of an electrochemist” because “electrochemists use different terms than solid state and semiconductor physicists, giving rise to additional confusion” (ibid.).

Three modern viewpoints on the contact vs. chemical controversy

Ultimately, none of the four advances discussed above managed to turn the tide of the debate over the reality of Voltaic contact potential in the action of batteries and other related phenomena. After the strong challenge from the chemical theory in the 1830s, the contact theory lost its clear dominance, and then faded away from electrochemistry step by step. However, efforts to bring the contact potential back into consideration in theorizing about batteries did not cease entirely, and carried on actively into the twentieth century. Kragh (2000, 153–154) notes the contributions of several physicists from the first half of the twentieth century. One of these is Alfred Porter, Professor of Physics at University College London, who focused on this topic in his 1928 Presidential Address to the Mathematical and Physical Sciences Section of the British Association for the Advancement of Science (BAAS), titled “The Volta Effect.” Porter’s presentation was notable in three respects. First, he began by straightforwardly accepting the reality of the “charging-by-contact” effect noted by Volta. Second, he drew close parallels between Voltaic and thermoelectric cells, giving prominence to the latter and expressing a suspicion of deeper links between the two effects. Third, he expressed a sense that even in 1928 (as fundamental physics was completing the establishment of the new quantum mechanics) “it is still necessary to be cautious and to avoid dogmatism on this question” (Porter 1928, 33). He argued that “much more detailed experimental knowledge is required before the electric circuit is really understood,” that “the electronic theory in metals still has its difficulties, which it is useless to ignore” (ibid.). “On the other hand,” he added, “the experimental difficulties in connexion with the direct measurement of Volta effect are also very great, as all who have made experiments on it must know”—this, despite all the progress made by an array of top-flight experimental physicists ranging from Thomson to Millikan and Langmuir (ibid.). Porter expressed very clearly the remaining sense of uncertainty and unfinished business that affected those who considered the issues carefully and openly. And among those who continued to take the contact potential seriously, there was no consensus on its role in batteries.

Twentieth-century discussions of contact vs. chemical theory were divided into three distinct directions of thought: “Perspectivism,” “Complementarity” and “Composition.”

Direction #1: Perspectivism. One line of thought was that the contact and the chemical theories were merely two different ways of analyzing the same set of phenomena, both equally valid. This is evident in the work of another twentieth-century scientist noted by Kragh: (John) Alan Chalmers, a Cambridge-trained atmospheric physicist who spent his entire career at the University of Durham and later became well-known for his textbook on the subject, published in 1949. His work on contact potentials was published in a thorough seven-part paper published in 1942 in the Philosophical Magazine. Chalmers began by noting that there was “a considerable controversy on the subject of ‘contact potentials,’ the main exponents of the two rival theories being Kelvin [Thomson], with a ‘contact’ or ‘physical’ theory, and Oliver Lodge, with a ‘chemical’ theory” (Chalmers 1842a, 399). He added that “the controversy died down without ever being finally settled and, as a result, the ideas of both theories still survive, sometimes in the same discussion” (ibid.). Chalmers sought to give a “complete account of the theory of ‘contact potentials,’ bringing both the older theories under consideration”—something for which, in his view, “no previous attempt had been made” (ibid.).

As Kragh notes, Chalmers’s conclusion concerning the contention between the contact and the chemical theories was one of equivalence; at least, he believed that the two theories—if not exactly equivalent to each other—were equally valid alternative perspectives. “The phenomena of the Volta effect,” argued Chalmers, “can be given a consistent interpretation in terms either of the external potential differences, agreeing with the contact theory, or of the internal potential differences, agreeing with the chemical theory; each way of regarding the matter is correct provided the ideas of each are kept separate” (Chalmers 1942b, 429). And his view was that the Voltaic cell was also to be understood according to the same principles (Chalmers 1942c, 505).

Chalmers defined the fundamental phenomenon of interest as follows: “The Volta effect comprises the measurement of the potential difference of a system consisting of two metals and a dielectric, usually air or a vacuum” (Chalmers 1942b, 418–419). As seen in Figure 4, the dielectric is placed between the two metal pieces (in the space indicated by the line with arrows), and the outward-facing metal surfaces (not in contact with the dielectric) are connected to each other by a metallic wire. In considering this system, one can easily imagine assigning the potential difference (or parts of it) to different parts of the system. Volta, and later Thomson, “considered the potential difference to originate at the metal-metal contact,” while De la Rive, and later Lodge, considered that “the Volta potential difference originates at the metal-dielectric contacts” (ibid.). Both accounts are correct, but they mean different things by “potential difference”: for the contact theorists, it is the “external potential difference” defined between just outside the two metal pieces, and for the chemical theorists it is the “internal potential difference” defined from the actual metallic surface layers (Chalmers 1942a, 411–413).

Figure 4. Diagram of the Volta effect, from Chalmers (1942b, 416).

I have not seen evidence that Chalmers’s account had a great influence. However, his sort of idea did not disappear entirely, either. In 1977, the Electrochemical Society (in the United States) organized a historical symposium as part of its seventy-fifth anniversary celebration. The volume resulting from this symposium included a paper by Sydney Ross, Glasgow-born American chemist who taught for many years at the Rensselaer Polytechnic Institute (RPI). ¹² Blasting Ostwald’s old triumphalist denunciation of Volta, Ross opined that:

From our own vantage point in time we are better aware that Volta had indeed got hold of the stick by the right end. Meanwhile, recent developments in our knowledge of the solid state promise us new advances in our understanding of the Voltaic cell, on the basis of Volta’s theory—a belated atonement for its long period of derision and neglect. (1978, 258) ¹³

After a brief yet instructive historical overview of “the story of Volta potential,” Ross concluded that there was a deep equivalence between the contact and chemical theories of Voltaic cell action.

Ross did not claim originality for this observation. He highlighted the little-known work of E. A. Guggenheim from 1929, which in his view should have been the end of the long-running controversy. Guggenheim demonstrated (in Ross’s words) that:

the difference in the chemical potentials of the electrons in the two metallic electrodes is equal to the difference in chemical potentials between the reactants and products of the cell reaction. In other words the cell reaction is simply the mechanism by which electrons are transported from one electrode to another. The change of the Gibbs free energy that determines the electromotive force of the cell can be indifferently ascribed either to the difference in chemical potential of the electrons in the cathode and in the anode, or to the cell reaction, whatever it may be, by means of which electrons are so transferred. (Ross 1978, 266–267)

Ross then noted that this “equivalence of the two theories … was ultimately made painstakingly clear in a series of seven papers by Chalmers” in 1942 (ibid.). George Dubpernell, one of the editors of the Proceedings in which Ross’s paper is published, himself stated in 1975 that contact potentials and the electromotive force of cells were “merely two different methods of measuring the same quantity” (quoted in Ross 1978, 266).

Direction #2: Complementarity. Ross’s discussion of the relation between the contact and the chemical theories also reflects another of the three directions of thought noted above—namely that the contact and chemical theories were complementary, accounting for different aspects of the whole story. Ross emphasized that recognizing the reality of the contact potential does not mean denying the key role played by chemical reactions. He gave a useful analogy: “A potential difference exists, for example, between the top and bottom of a cliff, but no energy can be obtained until water falls over the edge” (Ross 1978, 260). Ross noted that essentially the same idea had been put forward by J. Willard Gibbs through a mechanical analogy, at the 1884 meeting of the BAAS in Montreal, where a heated debate took place about contact potentials: “In a hot-water-circulation system the energy certainly derives from the fuel consumed in the furnace; but the moving force maintaining the circulation is the difference in weight between the cold column and the hot, and, so to speak, is located in the pipes, not in the furnace” (ibid.). Langmuir’s analogy of the water turbine, noted above, made essentially the same point.

Ross emphasized one important point: “mere contact provides no process that can produce energy to maintain a current” (ibid.). The chemical theorists are therefore correct in claiming that what happens in a wet Voltaic cell is the conversion of chemical potential energy to electrical energy (and in a thermocouple, the conversion of thermal energy to electrical energy), as Helmholtz first pointed out clearly and systematically in 1847. The contact potential and chemical reactions thus both have crucial roles to play in the workings of the Voltaic cell. Ross noted that Humphry Davy, in 1806, had already seen this complementarity: “The electrical energies of the metals with regard to each other, or the substances dissolved in the water … seem to be the causes that disturb the equilibrium, and the chemical changes the causes that tend to restore the equilibrium; and the phenomena most probably depend on their joint agency” (Davy quoted by Ross 1978, 260–261). He quoted Neil Kensington Adam, Professor of Chemistry at Southampton, for a “modern” version of Davy’s idea: “All these flows of charged particles across the phase boundaries are attempts, generally vain, to restore the equilibrium state of the double layers at these boundaries” (Adam 1938, 319, quoted by Ross 1978, 261).

Roland M. Lichtenstein, Ross’s physicist colleague at RPI, supplied an appendix to Ross’s paper with a detailed consideration of some of Thomson’s original experiments and reasoning. His conclusion makes interesting reading, along the same lines of Ross’s verdict:

Volta was therefore correct in accounting for the voltage of such a pile in terms of the contact potentials between the plates separated by the dry contacts. However the electrical energy delivered by such a pile when current is drawn comes from the couples formed by those plates that are connected by wet contacts. So the one pair, Cu/Dry/Zn, determines the voltage; whereas another pair, Zn/Wet/Cu, produces the electrical energy from chemical reactions. Thus the seat of power is not the same as the seat of voltage. (Lichstenstein 1978, 278)

Direction #3: Composition. There is yet another view on the whole situation, which is that all kinds of contacts, including the ones at which chemical reactions occur, generate contact potentials, and adding them all up gives the overall voltage of a battery. Langmuir had quite a clear view in this direction: “It is not possible to assume, however, that all the electromotive force of an electrolytic [sic] cell is caused by the contact electromotive force. It is a demonstrated fact that the E. M. F. of cells varies with the electrolyte used, while the contact potential, being an intrinsic property of the metals, cannot depend on the electrolyte” ([1916] 1961, 212–213). Langmuir tried to assess how much of a known electrode potential is due to the contact potential and how much of it is due to other causes, but had to conclude that the available experimental data were not reliable enough for this purpose. However, he considered this an important task because the overall electrode potentials are thermodynamic quantities only applying to reversible equilibrium conditions, and the contact potentials would need to be known for investigations of kinetics and mechanisms, as discussed above.

Adam, citing Langmuir’s work with great appreciation, expressed this view very clearly (this is despite the fact that the passage quoted above suggests a complementarity view): “Thus the driving force of the galvanic [voltaic] cell is the algebraic sum of the escaping tendencies of the charged components across the various phase boundaries in the cell” (Adam 1938, 318). Referring back to Langmuir’s data, Adam concluded that in terms of magnitudes “the contact potential between the two metals (difference between the work functions) is the principal factor determining the e.m.f. of galvanic cells consisting of two metals; it is generally decidedly larger than the difference between the two electrode–electrolyte potentials” (ibid.). If the latter quantity were much larger than the bimetallic contact potential, then the view of the chemical theorists would be justified—namely that the main driving force in a battery is the imbalance in the strengths of chemical reactions occurring at the two electrodes. This was Adam’s answer to the old question about the function of the liquid electrolyte in a battery: “The function of the electrolyte is to introduce two metal–liquid phase boundary potentials, whose magnitudes are such that their algebraic sum is not equal and opposite to the contact potential between the dry metals” (ibid., 319).

Concluding remarks: The pluralistic survival of the contact potential

The Voltaic contact potential seems to be not so much dead as un-dead: it keeps coming back, but it also fails to get fully resurrected. How do we account for this strange historical phenomenon? Looking back at the long and complex history of the contact potential, originating from Volta’s research, I cannot help thinking that it remains a valid and pertinent concept. The contact potential was declared to be non-existent only by those whose own practices did not find much use for it. Time and again, when various scientists took a serious look at the concept of the contact potential and made honest investigations of it, they found that there was something real behind it. In each well-specified context, serious and sustained investigations have made clear what the contact potential means and how it manifests itself in measurable phenomena; and there are phenomena that are difficult to understand without the concept of contact potential.

I think the contact potential has displayed significant meaning and utility in various experimental and theoretical contexts, but it has not been possible to unify its meaning across the different contexts. So there is “something there” in the various phenomena, but it has never been clear what that something is, for anyone looking for a simple and universal entity. The difficulty, for those who want a simple picture, is that various empirical assessments of the strength of contact potential have not matched up nicely (e.g., the electro-chemical, thermo-electric and work-function series of metals), and there has never been a successful overarching theory to explain all manifestations of it. As a result, the contact potential has remained a useful concept, but one marked by a persistent lack of unity.

One might imagine that, by the middle of the twentieth century, quantum mechanics would have provided a unified account of contact-potential phenomena. The situation, however, is not at all as straightforward as it may appear at first glance. The Schrödinger quantum mechanics that was so successful in giving an account of atomic spectra, and later in establishing the quantum chemistry of simpler molecules, has never been so straightforwardly useful in handling macroscopic condensed-matter physics. It is difficult enough to give a quantum-mechanical treatment of a metallic surface by itself, and the work function is treated simply as a phenomenological number in most contexts of application. When it comes to the treatment of metal–electrolyte interfaces, perhaps the most important kind of physical region in electrochemistry, advanced texts in electrochemistry give elaborate treatments, but these are not fully quantum-mechanical. Bockris and Reddy (1970) do start a detailed treatment of “the quantum aspects of charge-transfer fractions at electrode–solution interfaces,” 947 pages into their masterful textbook. However, even this is nothing like the reductionist dream of solving a massive multi-particle Schrödinger equation. In the above-mentioned modern analysis of Volta’s experiment, it is notable that Lichtenstein (1978) does not speak of electrons or quantum mechanics, staying quite strictly at the phenomenal level of metallic plates and electrometer readings, only theorizing very lightly about electric fields. It is only in recent works like Peljo et al. (2016) that we begin to see a serious quantum-mechanical treatment of contact-potential phenomena.

Given the absence of a unified account based on a fundamental theory, it makes sense to consider the contact potential as an example of what Giuliano Pancaldi calls “mid-range” concepts or conceptualizations (Pancaldi 2003, 116–120; Pancaldi 2011, 276). These concepts are designed to handle observable phenomena without commitments to “deep” theoretical explanations and ultimate causes. Such concepts tend to be strongly shaped by iconic instruments and the standard phenomena produced by means of them. Pancaldi’s detailed discussion of the shaping of tension and other related concepts in Volta’s work certainly bears out this characterization. What I would add to Pancaldi’s analysis is an explicit recognition that mid-range concepts tend to be “local,” each deriving its meaning and cogency from a particular set of phenomena and practices. That creates an interesting quandary when a given mid-range concept is used in a wide variety of circumstances. Modern treatments of contact potential have tended to acknowledge the complexity by giving different treatments of various types of situations. The contact potential can appear in very different guises in different situations; that was seen by Chalmers, for example, when he distinguished the external and the internal potential difference.

All this is not to say that there could not be a unified theory of contact potentials. Some advances have been made in synthesis, and Langmuir showed the way here. He made a plausible attempt to explain the EMF of batteries in terms of contact potentials, and to reconcile the values of standard electrode potentials and contact potentials (Langmuir [1916] 1961, 213, Table VI; see also Adam 1938, 310, Table XVI). Langmuir also made a worthy attempt to provide a unified account of Voltaic phenomena and thermoelectricity. The calculations of the contact potential between two metals from thermoelectric phenomena (the Peltier effect, in particular) seemed to show that the values were in the order of “one hundredth of those found by Volta, and seemed to bear no simple relation to these.” But Langmuir followed Thomson’s and Helmholtz’s view that “the Peltier effect does not correspond directly to the contact potential, but is related to the temperature coefficient of the contact potential” (Langmuir [1916] 1961, 174–175, emphasis original; see also Hong 1994, 256).

However, there would still be a long way to go if one were to pursue a complete unified account of all contact-potential phenomena, including the Volta effect (charging-by-contact), electrochemical cells, thermocouples and the Peltier effect, both thermionic and photoelectric work functions, and whatever is really going on in dry piles. W. R. Harper (1951), physicist at Imperial College London, even added frictional electricity to this mix, by demonstrating that—contrary to popular opinion—charging-by-friction was possible between two metals, and that this was due to the contact potential. A unified theory of contact potentials is a tall order. It would have to deal with the quantum mechanics of metals and solutions, somehow combine traditional electrostatic and quantum-mechanical reasoning, reason convincingly about double layers and adsorption, deal with free electrons in a vacuum and solid-state configurations of particles at the same time, and generally combine thermodynamic and kinetic considerations.

As the situation stands, I think it would be sensible to separate out different concepts of contact potential, and preserve and develop each one. And let us not imagine that the contact potential is a strange and unique case in this regard. Fundamental scientific concepts often incorporate many different ideas into one, as Evelyn Fox Keller has shown with the notion of the “gene” in The Century of the Gene (2000). That holds in physics, too. Consider, for example, how the notion of “force” manifests itself in numerous guises in various branches of science, no longer with any kind of unifying meaning like that provided by the framework of Newtonian physics with its F=ma. Or take the concept of light, which is specified as an electromagnetic wave, as a collection of photons, as rays of geometric optics, and so on. Scientists and engineers in their myriad practices have preserved all these concepts of light and employed them effectively in their appropriate domains. Older concepts and theories are preserved where they are effective, while new ones are developed and used in other domains. Most scientists and engineers practice what I have termed “conservationist pluralism,” even as some of them pay lip-service to the ideal of unified theory and universal laws. The story of the Voltaic contact potential is an excellent reminder of this reality of scientific practice.