Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T20:12:20.668Z Has data issue: false hasContentIssue false
  • English
  • Français

QUANTIFIED INTUITIONISTIC LOGIC OVER METRIZABLE SPACES

Published online by Cambridge University Press:  08 April 2019

PHILIP KREMER
PHILIP KREMER*
Affiliation:
Department of Philosophy, University of Toronto Scarborough
*
*DEPARTMENT OF PHILOSOPHY UNIVERSITY OF SCARBOROUGH 1265 MILITARY TRAIL TORONTO, ON M1C 1A4, CANADA E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In the topological semantics, quantified intuitionistic logic, QH, is known to be strongly complete not only for the class of all topological spaces but also for some particular topological spaces — for example, for the irrational line, 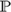${\Bbb P}$, and for the rational line, 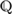${\Bbb Q}$, in each case with a constant countable domain for the quantifiers. Each of 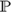${\Bbb P}$ and 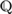${\Bbb Q}$ is a separable zero-dimensional dense-in-itself metrizable space. The main result of the current article generalizes these known results: QH is strongly complete for any zero-dimensional dense-in-itself metrizable space with a constant domain of cardinality ≤ the space’s weight; consequently, QH is strongly complete for any separable zero-dimensional dense-in-itself metrizable space with a constant countable domain. We also prove a result that follows from earlier work of Moerdijk: if we allow varying domains for the quantifiers, then QH is strongly complete for any dense-in-itself metrizable space with countable domains.

Keywords

03B20quantified intuitionistic logictopological semanticscompleteness
Type
Research Article
Information
The Review of Symbolic Logic , Volume 12 , Issue 3 , September 2019 , pp. 405 - 425
Copyright
Copyright © Association for Symbolic Logic 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Awodey, S. & Kishida, K. (2008). Topology and modality: The topological intepretation of first-order modal logic. Review of Symbolic Logic, 1, 146–166.CrossRefGoogle Scholar
Dragalin, A. G. (1988). Mathematical Intuitionism: Introduction to Proof Theory, trans. Mendelson, E., Translations of Mathematical Monographs, Vol. 67. Providence, RI: American Mathematical Society. (Russian original: 1979.)CrossRefGoogle Scholar
Dugundji, J. (1966). Topology. Boston: Allyn and Bacon.Google Scholar
Engelking, R. (1978). Dimension Theory. Amsterdam: North-Holland.Google Scholar
Engelking, R. (1989). General Topology. Berlin: Heldermann Verlag.Google Scholar
Fourman, M. P. & Scott, D. S. (1979). Sheaves and logic. In Fourman, M. P., Mulvey, C. J., and Scott, D. S., editors. Applications of Sheaves, Lecture Notes in Mathematics, Vol. 753. Berlin: Springer-Verlag, pp. 302–401.CrossRefGoogle Scholar
Gabbay, D. M., Shehtman, V. B., & Skvortsov, D. (2009). Quantification in Nonclassical Logic, Vol. 1. Amsterdam: Elsevier.Google Scholar
Gierz, G., Hoffmann, K. H., Keimel, K., Lawson, J. D., Mislove, M., & Scott, D. S. (2003). Continuous Lattices and Domains. Cambridge: Cambridge University Press.CrossRefGoogle Scholar
Kremer, P. (2013). Strong completeness of S4 for any dense-in-itself metric space. Review of Symbolic Logic, 6, 545–570.CrossRefGoogle Scholar
Kremer, P. (2014). Quantified modal logic on the rational line. Review of Symbolic Logic, 7, 439–454.CrossRefGoogle Scholar
Kremer, P. (2014). Quantified S4 in the Lebesgue measure algebra with a constant countable domain, unpublished manuscript.Google Scholar
Lando, T. First order S4 and its measure-theoretic semantics. Annals of Pure and Applied Logic, 166, 187–218.CrossRefGoogle Scholar
McKinsey, J. C. C. (1941). A solution of the decision problem for the Lewis systems S2 and S4, with an application to topology. The Journal of Symbolic Logic, 6, 117–134.CrossRefGoogle Scholar
McKinsey, J. C. C. & Tarski, A. (1944). The algebra of topology. Annals of Mathematics, 45, 141–191.CrossRefGoogle Scholar
Medini, A. & Milovich, D. (2012). The topology of ultrafilters as subspaces of 2ω. Topology and its Applications, 159, 1318–1333.CrossRefGoogle Scholar
Moerdijk, I. (1982). Some topological spaces which are universal for intuitionistic predicate logic. Indagationes Mathematicae (Proceedings), 85, 227–235.CrossRefGoogle Scholar
Moschovakis, J. (2018). Intuitionistic logic. In Zalta, E. N., editor. The Stanford Encyclopedia of Philosophy (Winter 2018 edition). Available at: https://plato.stanford.edu/entries/logic-intuitionistic/.Google Scholar
Mostowski, A. (1948). Proofs of nondeducibility in intuitionistic functional calculus. Journal of Symbolic Logic, 13, 204–207.CrossRefGoogle Scholar
Rasiowa, H. (1951). Algebraic treatment of the functional calculi of Heyting and Lewis. Fundamenta Mathematicae, 38, 99–126.CrossRefGoogle Scholar
Rasiowa, H. & Sikorski, R. (1963). The Mathematics of Metamathematics. Warsaw: Państowowe Wydawnictwo Naukowe.Google Scholar
Sørensen, M. H. & Urzyczyn, P. (2006). Lectures on the Curry-Howard Isomorphism, Studies in Logic and the Foundations of Mathematics, Vol. 149. Amsterdam: Elsevier.Google Scholar