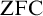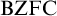1. Introduction
The Zermelo–Fraenkel axiom system,
![]() ${\mathrm {ZFC}}$
, is generally accepted as the foundation of mathematics.
${\mathrm {ZFC}}$
, is generally accepted as the foundation of mathematics.
![]() ${\mathrm {ZFC}}$
is formalized in classical logic, in which any statement is either true or false, and cannot be both at the same time. Is there a possible interest in considering a set theory in which statements can be neither true nor false (paracomplete) or, more significantly, both true and false (paraconsistent)?
${\mathrm {ZFC}}$
is formalized in classical logic, in which any statement is either true or false, and cannot be both at the same time. Is there a possible interest in considering a set theory in which statements can be neither true nor false (paracomplete) or, more significantly, both true and false (paraconsistent)?
The most common motivation for developing such a theory has been the wish to avoid Russell’s paradox and maintain some form of naive comprehension, i.e., the axiom scheme “
![]() $\exists x \forall y (y \in x \leftrightarrow \varphi (y))$
” for every
$\exists x \forall y (y \in x \leftrightarrow \varphi (y))$
” for every
![]() $\varphi $
. In spite of the inconsistency of this scheme, many philosophers have found it more natural and intuitive than
$\varphi $
. In spite of the inconsistency of this scheme, many philosophers have found it more natural and intuitive than
![]() ${\mathrm {ZFC}}$
, and have proposed alternative solutions, among others by adopting a logic in which contradictory statements can co-exist without trivializing. See, for instance, the work of Graham Priest [Reference Priest22], Greg Restall [Reference Restall24], Thierry Libert [Reference Libert17], the survey by Roland Hinnion [Reference Hinnion14], and the recent book by Zach Weber [Reference Weber28].
${\mathrm {ZFC}}$
, and have proposed alternative solutions, among others by adopting a logic in which contradictory statements can co-exist without trivializing. See, for instance, the work of Graham Priest [Reference Priest22], Greg Restall [Reference Restall24], Thierry Libert [Reference Libert17], the survey by Roland Hinnion [Reference Hinnion14], and the recent book by Zach Weber [Reference Weber28].
Nevertheless, it is not hard to see, and has been known for a long time (see, e.g., [Reference Geach12]), that paraconsistency or paracompleteness alone are not sufficient to sustain naive set theory, since one can easily appeal to a version of Curry’s paradox instead, and consider
for an arbitrary proposition
![]() $\varphi $
. Any logic satisfying Modus Ponens and the implication-introduction rule can derive
$\varphi $
. Any logic satisfying Modus Ponens and the implication-introduction rule can derive
![]() $\varphi $
from the assumption that R is a set,Footnote
1
showing that naive comprehension can lead to a trivial theory without mentioning the negation connective at all. The only way to truly avoid paradoxes is to consider a logic that either does not have an implication (such as the logic of paradox of [Reference Priest22]), or whose “
$\varphi $
from the assumption that R is a set,Footnote
1
showing that naive comprehension can lead to a trivial theory without mentioning the negation connective at all. The only way to truly avoid paradoxes is to consider a logic that either does not have an implication (such as the logic of paradox of [Reference Priest22]), or whose “
![]() $\to $
”-symbol is so far removed from its common usage that it violates basic principles of reasoning. Either way, the price one has to pay seems too high.
$\to $
”-symbol is so far removed from its common usage that it violates basic principles of reasoning. Either way, the price one has to pay seems too high.
Perhaps this pre-occupation with naive comprehension explains why most work in paraconsistent set theory has so far remained speculative and philosophical in nature, never really ‘lifting off the ground’.
But what if we focus our attention on other reasons for studying paracomplete and paraconsistent phenomena in mathematics? After all, there are many ‘interesting inconsistencies’ in mathematics quite aside of the semantic paradoxes. To name a prominent example, think of Kunen’s inconsistency, a result that puts an upper bound on the hierarchy of large cardinal axioms [Reference Kunen16]. It is conceivable that paraconsistency can shed new light on this or related phenomena. On a more down-to-earth level, applications are conceivable in computer science, for example in the study of databases or structures with incomplete or inconsistent information. Following Belnap [Reference Belnap7, Reference Belnap8], suppose we want an automated system to derive logical consequences from the information in a given database. Presumably, this system should not be able to derive any consequence whatsoever merely from the fact that
![]() $A(x) \land \lnot A(x)$
holds, which could be due to a wrong entry in the database. Some other, more specific applications to mathematical problems are listed in Section 11.
$A(x) \land \lnot A(x)$
holds, which could be due to a wrong entry in the database. Some other, more specific applications to mathematical problems are listed in Section 11.
If a solid foundational framework for paracomplete and paraconsistent set theory is to be set up, it should, in our view, satisfy a number of criteria:
-
1. It should be intuitive and philosophically motivated.
-
2. It should be sufficiently similar to
 ${\mathrm {ZFC}}$
to enable the development of interesting mathematics in it.
${\mathrm {ZFC}}$
to enable the development of interesting mathematics in it. -
3. There should be a tangible ‘ontology’, i.e., a working mathematician should be able to understand and ‘visualize’ paracomplete and paraconsistent sets and how to manipulate them, without a need to resort to formalism or double-check the axioms.
-
4. The existence of paraconsistent and paracomplete sets should be guaranteed by virtue of the axioms, and these sets should ‘extend’ the von Neumann universe of classical sets.
-
5. It should be possible to construct a model for this theory starting from
 ${\mathrm {ZFC}}$
, showing that it is no more problematic than classical set theory.
${\mathrm {ZFC}}$
, showing that it is no more problematic than classical set theory.
This paper provides an exposition of a system which, we claim, satisfies all of these criteria. To the best of our knowledge, nothing like this has been done before.
Our theory is established in the logic
![]() $\mathrm {BS4}$
: a four-valued logic based on early developments by Dunn [Reference Dunn11], Belnap [Reference Belnap7, Reference Belnap8] and [Reference Avron4], later appearing under the name CLoNs in [Reference Batens, De Clercq and Kurtonina6], and the predicate version due to Omori, Sano and Waragai [Reference Omori and Waragai20, Reference Sano and Omori25] (from where we take its name). These logics are called logics of formal inconsistency by Carnieli et al. [Reference Carnielli and Coniglio9]. In Section 2 we will introduce
$\mathrm {BS4}$
: a four-valued logic based on early developments by Dunn [Reference Dunn11], Belnap [Reference Belnap7, Reference Belnap8] and [Reference Avron4], later appearing under the name CLoNs in [Reference Batens, De Clercq and Kurtonina6], and the predicate version due to Omori, Sano and Waragai [Reference Omori and Waragai20, Reference Sano and Omori25] (from where we take its name). These logics are called logics of formal inconsistency by Carnieli et al. [Reference Carnielli and Coniglio9]. In Section 2 we will introduce
![]() $\mathrm {BS4}$
, with a focus on semantics.
$\mathrm {BS4}$
, with a focus on semantics.
The axiom system we propose is called
![]() ${\mathrm {BZFC}}$
. Intuitively, it can be seen as axiomatising a universe that properly extends the classical universe of sets and includes incomplete sets (u such that for some x the statement
${\mathrm {BZFC}}$
. Intuitively, it can be seen as axiomatising a universe that properly extends the classical universe of sets and includes incomplete sets (u such that for some x the statement
![]() $x \in u \lor x \notin u$
fails to be true) and inconsistent sets (u such that for some x,
$x \in u \lor x \notin u$
fails to be true) and inconsistent sets (u such that for some x,
![]() $x\in u \land x \notin u$
is true). Each non-classical set u can be described by a positive extension (the collection of all x such that
$x\in u \land x \notin u$
is true). Each non-classical set u can be described by a positive extension (the collection of all x such that
![]() $x \in u$
is true) and a negative extension (the collection of all x such that
$x \in u$
is true) and a negative extension (the collection of all x such that
![]() $x \in u$
is false).Footnote
2
$x \in u$
is false).Footnote
2
The first step of our task is finding an appropriate translation of the
![]() ${\mathrm {ZFC}}$
axioms to the non-classical setting. This is more laborious than might be expected, and previous attempts (e.g., [Reference Carnielli and Coniglio9]) may well have stumbled over an insufficiently careful treatment of the
${\mathrm {ZFC}}$
axioms to the non-classical setting. This is more laborious than might be expected, and previous attempts (e.g., [Reference Carnielli and Coniglio9]) may well have stumbled over an insufficiently careful treatment of the
![]() ${\mathrm {ZFC}}$
axioms in this regard. It would be a mistake to copy them literally; instead, one must think what the axioms were designed to achieve, and fine-tune the formulation to match this design. This is done in Sections 3 and 4.
${\mathrm {ZFC}}$
axioms in this regard. It would be a mistake to copy them literally; instead, one must think what the axioms were designed to achieve, and fine-tune the formulation to match this design. This is done in Sections 3 and 4.
This leads us to an intermediate system, which we call
![]() $\mathrm {PZFC}$
: an appropriate translation of the old axioms but not yet guaranteeing that any non-classical sets do exist. Indeed,
$\mathrm {PZFC}$
: an appropriate translation of the old axioms but not yet guaranteeing that any non-classical sets do exist. Indeed,
![]() $\mathrm {PZFC}$
together with the statement that all sets are classical, is easily seen to be equivalent to
$\mathrm {PZFC}$
together with the statement that all sets are classical, is easily seen to be equivalent to
![]() ${\mathrm {ZFC}}$
. The actual system
${\mathrm {ZFC}}$
. The actual system
![]() ${\mathrm {BZFC}}$
is then obtained by adding to
${\mathrm {BZFC}}$
is then obtained by adding to
![]() $\mathrm {PZFC}$
an anti-classicality axiom, postulating the existence of paraconsistent and paracomplete sets, which is done in Section 5. Here we prove an unexpected, yet surprisingly simple result showing that, essentially, all ‘anti-classicality axioms’ are equivalent (Theorem 5.2).
$\mathrm {PZFC}$
an anti-classicality axiom, postulating the existence of paraconsistent and paracomplete sets, which is done in Section 5. Here we prove an unexpected, yet surprisingly simple result showing that, essentially, all ‘anti-classicality axioms’ are equivalent (Theorem 5.2).
Hinging on this crucial fact, it is not difficult to construct a model
![]() ${\mathbb {W}}$
of
${\mathbb {W}}$
of
![]() ${\mathrm {BZFC}}$
starting from classical
${\mathrm {BZFC}}$
starting from classical
![]() ${\mathrm {ZFC}}$
. On the other hand, in
${\mathrm {ZFC}}$
. On the other hand, in
![]() ${\mathrm {BZFC}}$
itself we can define an inner model
${\mathrm {BZFC}}$
itself we can define an inner model
![]() ${\mathbb {HCL}}$
of ‘hereditarily classical’ sets and prove (in
${\mathbb {HCL}}$
of ‘hereditarily classical’ sets and prove (in
![]() ${\mathrm {BZFC}}$
) that
${\mathrm {BZFC}}$
) that
![]() ${\mathbb {HCL}} \models {\mathrm {ZFC}}$
. In addition, this mutual interpretation is reversible leading to the fact that
${\mathbb {HCL}} \models {\mathrm {ZFC}}$
. In addition, this mutual interpretation is reversible leading to the fact that
![]() ${\mathrm {BZFC}}$
is bi-interpretable with
${\mathrm {BZFC}}$
is bi-interpretable with
![]() ${\mathrm {ZFC}}$
(Theorem 9.1) These results are proved in Sections 7–9, preceded by Section 6 in which we build up some needed technology.
${\mathrm {ZFC}}$
(Theorem 9.1) These results are proved in Sections 7–9, preceded by Section 6 in which we build up some needed technology.
Some readers may feel that the bi-interpretability result limits the significance of our theory, but we would argue for quite the contrary. To us, the resulting picture is philosophically very satisfying: if one’s position favours the existence of paracomplete and paraconsistent sets, one can view
![]() ${\mathrm {BZFC}}$
as describing the true universe, an enrichment of the usual universe of classical sets.
${\mathrm {BZFC}}$
as describing the true universe, an enrichment of the usual universe of classical sets.
![]() ${\mathrm {ZFC}}$
is the theory of the inner model
${\mathrm {ZFC}}$
is the theory of the inner model
![]() ${\mathbb {HCL}}$
in which all of classical mathematics takes place. As long as we are only interested in classical mathematics, we can stay within
${\mathbb {HCL}}$
in which all of classical mathematics takes place. As long as we are only interested in classical mathematics, we can stay within
![]() ${\mathbb {HCL}}$
; but whenever we encounters phenomena better described by paracomplete or paraconsistent sets, we can look beyond and take full advantage of
${\mathbb {HCL}}$
; but whenever we encounters phenomena better described by paracomplete or paraconsistent sets, we can look beyond and take full advantage of
![]() ${\mathrm {BZFC}}$
.
${\mathrm {BZFC}}$
.
On the other hand, if one is firmly committed to classical logic and cannot accept true contradictions or the lack of excluded middle, one can still consider
![]() ${\mathrm {BZFC}}$
a useful theory, namely the theory of the model
${\mathrm {BZFC}}$
a useful theory, namely the theory of the model
![]() ${\mathbb {W}}$
, using the semantics of
${\mathbb {W}}$
, using the semantics of
![]() $\mathrm {BS4}$
. All of paraconsistent and paracomplete set theory as layed out in this paper, can then be understood as formal statements that
$\mathrm {BS4}$
. All of paraconsistent and paracomplete set theory as layed out in this paper, can then be understood as formal statements that
![]() ${\mathbb {W}}$
believes to be true; but this fact is established in classical
${\mathbb {W}}$
believes to be true; but this fact is established in classical
![]() ${\mathrm {ZFC}}$
.
${\mathrm {ZFC}}$
.
Here, we would like to draw a parallel to another extension of classical set theory, namely the theory ZFA of non-well-founded sets of Peter Aczel [Reference Aczel1]. Note that ZFA is also bi-interpretable with ZFC, and a similar philosophical freedom is available to the reader as above. At the same time, ZFA and the concept of non-well-founded sets has found many useful applications in mathematics, philosophy and computer science.
The final Section 10 is devoted to a question of interest for the philosophy of logic, namely the relationship between properties of a formal language and the meta-language in which it is defined. Starting from
![]() $\mathrm {PZFC}$
, we show that, if a consequence relation
$\mathrm {PZFC}$
, we show that, if a consequence relation
![]() $\models $
is formalised with standard Tarski semantics, then the logic which is sound and complete with respect to those semantics satisfies the same paraconsistency and paracompleteness as in the meta-theory.
$\models $
is formalised with standard Tarski semantics, then the logic which is sound and complete with respect to those semantics satisfies the same paraconsistency and paracompleteness as in the meta-theory.
The work in this paper was carried out in the course of the Master’s thesis of the second author [Reference Oddsson19]. On occasion, we will refer to the thesis which goes in more depth on some points, and contains more details which have been left out of this paper for clarity.
2. The logic
 $\mathrm {BS4}$
$\mathrm {BS4}$
The logic
![]() $\mathrm {BS4}$
is due to [Reference Sano and Omori25] with the exception that we take the contradictory constant
$\mathrm {BS4}$
is due to [Reference Sano and Omori25] with the exception that we take the contradictory constant
![]() $\bot $
as primary instead of the classicality operator
$\bot $
as primary instead of the classicality operator
![]() $\circ $
. In this section, the meta-theory is classical
$\circ $
. In this section, the meta-theory is classical
![]() ${\mathrm {ZFC}}$
.
${\mathrm {ZFC}}$
.
2.1. Syntax and semantics
The main idea behind
![]() $\mathrm {BS4}$
is the separation of truth from falsity, i.e., if
$\mathrm {BS4}$
is the separation of truth from falsity, i.e., if
![]() $\varphi $
is a sentence and
$\varphi $
is a sentence and
![]() $\mathcal {M}$
a model, then
$\mathcal {M}$
a model, then
![]() $\varphi $
can be or not be true in
$\varphi $
can be or not be true in
![]() $\mathcal {M}$
and, independently, can be or not be false in
$\mathcal {M}$
and, independently, can be or not be false in
![]() $\mathcal {M}$
. This is achieved by considering two interpretation of all predicate symbols (a “true” and a “false” interpretation), adapting the inductive definition for the connectives and quantifiers, and thus obtaining two satisfaction relations:
$\mathcal {M}$
. This is achieved by considering two interpretation of all predicate symbols (a “true” and a “false” interpretation), adapting the inductive definition for the connectives and quantifiers, and thus obtaining two satisfaction relations:
![]() $\models ^T$
and
$\models ^T$
and
![]() $\models ^F$
. For convenience we will consider vocabularies without function symbols.
$\models ^F$
. For convenience we will consider vocabularies without function symbols.
Definition 2.1 (The syntax of
 $\mathrm {BS4}$
)
$\mathrm {BS4}$
)
The syntax of
![]() $\mathrm {BS4}$
is the usual syntax of first-order logic, except that we use “
$\mathrm {BS4}$
is the usual syntax of first-order logic, except that we use “
![]() $\sim $
” to denote negation. We also use the constant connective
$\sim $
” to denote negation. We also use the constant connective
![]() $\bot $
.
$\bot $
.
Definition 2.2 (T/F-models)
Suppose
![]() $\tau $
is a vocabulary with constant and relation symbols. A T/F-model
$\tau $
is a vocabulary with constant and relation symbols. A T/F-model
![]() $\mathcal {M}$
consist of a domain M together with the following:
$\mathcal {M}$
consist of a domain M together with the following:
-
• An element
 $c^{\mathcal {M}} \in M$
for every constant symbol c.
$c^{\mathcal {M}} \in M$
for every constant symbol c. -
• For every
 $n$
-ary relation symbol R, a “positive” interpretation
$n$
-ary relation symbol R, a “positive” interpretation
 $(R^{\mathcal {M}})^+ \subseteq M^n$
and a “negative” interpretation
$(R^{\mathcal {M}})^+ \subseteq M^n$
and a “negative” interpretation
 $(R^{\mathcal {M}})^- \subseteq M^n$
.
$(R^{\mathcal {M}})^- \subseteq M^n$
. -
• A binary relation
 $=^+$
which coincides with the true equality relation; and a binary relation
$=^+$
which coincides with the true equality relation; and a binary relation
 $=^-$
satisfying
$=^-$
satisfying
 $a =^- b$
iff
$a =^- b$
iff
 $b =^- a$
.
$b =^- a$
.
Definition 2.3 (T/F-semantics for
 $\mathrm {BS4}$
)
$\mathrm {BS4}$
)
Suppose
![]() $\tau $
is a vocabulary without function symbols and
$\tau $
is a vocabulary without function symbols and
![]() $\mathcal {M}$
a T/F-model. We define
$\mathcal {M}$
a T/F-model. We define
![]() $\models ^T$
and
$\models ^T$
and
![]() $\models ^F$
inductively:
$\models ^F$
inductively:
-
1.
 $\mathcal {M} \models ^T (t=s)[a,b] \;\;\; \Leftrightarrow \;\;\; a=^+ b$
.
$\mathcal {M} \models ^T (t=s)[a,b] \;\;\; \Leftrightarrow \;\;\; a=^+ b$
. $\mathcal {M} \models ^F (t=s)[a,b] \;\;\; \Leftrightarrow \;\;\; a =^- b$
.
$\mathcal {M} \models ^F (t=s)[a,b] \;\;\; \Leftrightarrow \;\;\; a =^- b$
. -
2.
 $\mathcal {M} \models ^T R(t_1, \dots , t_n)[a_1 \dots a_n] \;\;\; \Leftrightarrow \;\;\; R^+(a_1, \dots , a_n)$
holds.
$\mathcal {M} \models ^T R(t_1, \dots , t_n)[a_1 \dots a_n] \;\;\; \Leftrightarrow \;\;\; R^+(a_1, \dots , a_n)$
holds. $\mathcal {M} \models ^F R(t_1, \dots , t_n)[a_1 \dots a_n] \;\;\; \Leftrightarrow \;\;\; R^-(a_1, \dots , a_n)$
holds.
$\mathcal {M} \models ^F R(t_1, \dots , t_n)[a_1 \dots a_n] \;\;\; \Leftrightarrow \;\;\; R^-(a_1, \dots , a_n)$
holds. -
3.
 $\mathcal {M} \models ^T {\sim } \varphi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi $
.
$\mathcal {M} \models ^T {\sim } \varphi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi $
. $\mathcal {M} \models ^F {\sim } \varphi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
.
$\mathcal {M} \models ^F {\sim } \varphi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
. -
4.
 $\mathcal {M} \models ^T \varphi \land \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
and
$\mathcal {M} \models ^T \varphi \land \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
and
 $\mathcal {M} \models ^T \psi $
.
$\mathcal {M} \models ^T \psi $
. $\mathcal {M} \models ^F \varphi \land \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi $
or
$\mathcal {M} \models ^F \varphi \land \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi $
or
 $\mathcal {M} \models ^F \psi $
.
$\mathcal {M} \models ^F \psi $
. -
5.
 $\mathcal {M} \models ^T \varphi \lor \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
or
$\mathcal {M} \models ^T \varphi \lor \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
or
 $\mathcal {M} \models ^T \psi $
.
$\mathcal {M} \models ^T \psi $
. $\mathcal {M} \models ^F \varphi \lor \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi $
and
$\mathcal {M} \models ^F \varphi \lor \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi $
and
 $\mathcal {M} \models ^F \psi $
.
$\mathcal {M} \models ^F \psi $
. -
6.
 $\mathcal {M} \models ^T \varphi \to \psi \;\;\; \Leftrightarrow \;\;\;$
if
$\mathcal {M} \models ^T \varphi \to \psi \;\;\; \Leftrightarrow \;\;\;$
if
 $ \mathcal {M} \models ^T \varphi $
then
$ \mathcal {M} \models ^T \varphi $
then
 $\mathcal {M} \models ^T \psi $
.
$\mathcal {M} \models ^T \psi $
. $\mathcal {M} \models ^F \varphi \to \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
and
$\mathcal {M} \models ^F \varphi \to \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi $
and
 $\mathcal {M} \models ^F \psi $
.
$\mathcal {M} \models ^F \psi $
. -
7.
 $\mathcal {M} \models ^T \varphi \leftrightarrow \psi \;\;\; \Leftrightarrow \;\;\;$
(
$\mathcal {M} \models ^T \varphi \leftrightarrow \psi \;\;\; \Leftrightarrow \;\;\;$
(
 $\mathcal {M} \models ^T \varphi $
if and only if
$\mathcal {M} \models ^T \varphi $
if and only if
 $\mathcal {M} \models ^T \psi $
).
$\mathcal {M} \models ^T \psi $
). $\mathcal {M} \models ^F \varphi \leftrightarrow \psi \;\;\; \Leftrightarrow \;\;\;$
(
$\mathcal {M} \models ^F \varphi \leftrightarrow \psi \;\;\; \Leftrightarrow \;\;\;$
(
 $\mathcal {M} \models ^T \varphi $
and
$\mathcal {M} \models ^T \varphi $
and
 $\mathcal {M} \models ^F \psi $
) or (
$\mathcal {M} \models ^F \psi $
) or (
 $\mathcal {M} \models ^F \varphi $
and
$\mathcal {M} \models ^F \varphi $
and
 $\mathcal {M} \models ^T \psi $
).
$\mathcal {M} \models ^T \psi $
). -
8.
 $\mathcal {M} \models ^T \exists x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi [a]$
for some
$\mathcal {M} \models ^T \exists x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi [a]$
for some
 $a \in M$
.
$a \in M$
. $\mathcal {M} \models ^F \exists x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi [a]$
for all
$\mathcal {M} \models ^F \exists x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi [a]$
for all
 $a \in M$
.
$a \in M$
. -
9.
 $\mathcal {M} \models ^T \forall x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi [a]$
for all
$\mathcal {M} \models ^T \forall x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^T \varphi [a]$
for all
 $a \in M$
.
$a \in M$
. $\mathcal {M} \models ^F \forall x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi [a]$
for some
$\mathcal {M} \models ^F \forall x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models ^F \varphi [a]$
for some
 $a \in M$
.
$a \in M$
. -
10.
 $\mathcal {M} \models ^T \bot \;\;\; \Leftrightarrow \;\;\;$
never.
$\mathcal {M} \models ^T \bot \;\;\; \Leftrightarrow \;\;\;$
never. $\mathcal {M} \models ^F \bot \;\;\; \Leftrightarrow \;\;\; $
always.
$\mathcal {M} \models ^F \bot \;\;\; \Leftrightarrow \;\;\; $
always.
If
![]() $\mathcal {M} \models ^T \varphi $
then we say that
$\mathcal {M} \models ^T \varphi $
then we say that
![]() $\varphi $
is true in
$\varphi $
is true in
![]() $\mathcal {M}$
, and if
$\mathcal {M}$
, and if
![]() $\mathcal {M} \models ^F \varphi $
then we say that
$\mathcal {M} \models ^F \varphi $
then we say that
![]() $\varphi $
is false in
$\varphi $
is false in
![]() $\mathcal {M}$
.
$\mathcal {M}$
.
Definition 2.4 (Semantic consequence)
If
![]() $\Sigma $
is a set of formulas and
$\Sigma $
is a set of formulas and
![]() $\varphi $
another formula, then semantic consequence is defined by
$\varphi $
another formula, then semantic consequence is defined by
 $$ \begin{align*} &\Sigma \vdash_{\mathrm{BS4}} \varphi\\ \mathrm{iff\ for\ every\ T/F}\text{-}\mathrm{model}\ &\mathcal{M},\ \mathrm{if}\ \mathcal{M} \models^T \Sigma\ \mathrm{then}\ \mathcal{M} \models^T \varphi. \end{align*} $$
$$ \begin{align*} &\Sigma \vdash_{\mathrm{BS4}} \varphi\\ \mathrm{iff\ for\ every\ T/F}\text{-}\mathrm{model}\ &\mathcal{M},\ \mathrm{if}\ \mathcal{M} \models^T \Sigma\ \mathrm{then}\ \mathcal{M} \models^T \varphi. \end{align*} $$
Remark 2.5. While most of the inductive steps in Definition 2.3 are straightforward, two points need to be addressed:
-
1. The falsum symbol “
 $\bot $
” should not be understood as just a ‘contradiction’ but rather as a strong form of falsity, one which cannot be satisfied even in ‘paraconsistent’ models. Some readers might initially find the addition to
$\bot $
” should not be understood as just a ‘contradiction’ but rather as a strong form of falsity, one which cannot be satisfied even in ‘paraconsistent’ models. Some readers might initially find the addition to
 $\bot $
to the logic distasteful, as it seems to run counter to the idea of paraconsistency. However, in a vocabulary with finitely many relation symbols, one can write the following sentence: This sentence is satisfiable, but only in the trivial model consisting of exactly one object a, which is both equal and not equal to itself (
$\bot $
to the logic distasteful, as it seems to run counter to the idea of paraconsistency. However, in a vocabulary with finitely many relation symbols, one can write the following sentence: This sentence is satisfiable, but only in the trivial model consisting of exactly one object a, which is both equal and not equal to itself ( $$ \begin{align*}\forall x\forall y (x=y\land x\neq y)\land \bigwedge_{R \in S} \forall x_1 \dots \forall x_n (R(x_1,\dots,x_n)\land {\sim} R(x_1,\dots,x_n)).\end{align*} $$
$$ \begin{align*}\forall x\forall y (x=y\land x\neq y)\land \bigwedge_{R \in S} \forall x_1 \dots \forall x_n (R(x_1,\dots,x_n)\land {\sim} R(x_1,\dots,x_n)).\end{align*} $$
 $a =^+ a$
and
$a =^+ a$
and
 $a =^- a$
) and for which all relation-interpretations are true and false. Adding “
$a =^- a$
) and for which all relation-interpretations are true and false. Adding “
 $\bot $
” to the language is equivalent to disregarding this trivial model. Since we focus on set theory, we will have no interest in such a model and thus have no reservations about
$\bot $
” to the language is equivalent to disregarding this trivial model. Since we focus on set theory, we will have no interest in such a model and thus have no reservations about
 $\bot $
.
$\bot $
.
-
2. There is some freedom in choosing the truth- and falsity-conditions for the implication. For example, in the logic LP from [Reference Priest22], an implication
 $\varphi \to \psi $
would be an abbreviation for
$\varphi \to \psi $
would be an abbreviation for
 ${\sim } \varphi \lor \psi $
. But such a logic fails to satisfy implication-introduction rule and the deduction theorem, leading to many undesirable consequences, such as finite models of set theory, see [Reference Restall24]. In
${\sim } \varphi \lor \psi $
. But such a logic fails to satisfy implication-introduction rule and the deduction theorem, leading to many undesirable consequences, such as finite models of set theory, see [Reference Restall24]. In
 $\mathrm {BS4}$
, the truth-definition for the material implication reflects semantic consequence and makes sure that the deduction theorem is satisfied, while the falsity-condition reflects the existence of a counterexample.
$\mathrm {BS4}$
, the truth-definition for the material implication reflects semantic consequence and makes sure that the deduction theorem is satisfied, while the falsity-condition reflects the existence of a counterexample.Combining the material implication with
 $\bot $
, we will be able to define classical negation, and refer not only to truth and falsity, but also to the absence of truth and/or falsity, from within the system. But this should not be seen as a drawback of the system; indeed the original formulation of
$\bot $
, we will be able to define classical negation, and refer not only to truth and falsity, but also to the absence of truth and/or falsity, from within the system. But this should not be seen as a drawback of the system; indeed the original formulation of
 $\mathrm {BS4}$
from [Reference Sano and Omori25] explicitly contained a ‘classicality’ operator which generates an equivalent logic.
$\mathrm {BS4}$
from [Reference Sano and Omori25] explicitly contained a ‘classicality’ operator which generates an equivalent logic.
Definition 2.6 (Truth value)
For every
![]() $\varphi $
and T/F-model
$\varphi $
and T/F-model
![]() $\mathcal {M}$
we define:
$\mathcal {M}$
we define:

We can now view
![]() $\mathrm {BS4}$
as a four-valued logic with truth tables for propositional connectives given in Table 1.
$\mathrm {BS4}$
as a four-valued logic with truth tables for propositional connectives given in Table 1.
Table 1 Truth tables for the propositional connectives of
![]() $\mathrm {BS4}$
.
$\mathrm {BS4}$
.

2.2. Defined connectives
We will need several defined connectives to make the presentation more smooth and intuitive.
First let us consider material implication: notice that
![]() $\mathcal {M} \models ^T \varphi \to \psi $
tells us that if
$\mathcal {M} \models ^T \varphi \to \psi $
tells us that if
![]() $\varphi $
is true in
$\varphi $
is true in
![]() $\mathcal {M}$
then
$\mathcal {M}$
then
![]() $\psi $
is true in
$\psi $
is true in
![]() $\mathcal {M}$
, but does not tell us that if
$\mathcal {M}$
, but does not tell us that if
![]() $\psi $
is false in
$\psi $
is false in
![]() $\mathcal {M}$
then
$\mathcal {M}$
then
![]() $\varphi $
is false in
$\varphi $
is false in
![]() $\mathcal {M}$
, as can easily be verified. Similarly, a bi-implication
$\mathcal {M}$
, as can easily be verified. Similarly, a bi-implication
![]() $\varphi \leftrightarrow \psi $
tells us that, in a model
$\varphi \leftrightarrow \psi $
tells us that, in a model
![]() $\mathcal {M}$
,
$\mathcal {M}$
,
![]() $\varphi $
is true iff
$\varphi $
is true iff
![]() $\psi $
is true, but not that
$\psi $
is true, but not that
![]() $\varphi $
is false iff
$\varphi $
is false iff
![]() $\psi $
is false. In particular,
$\psi $
is false. In particular,
![]() $\varphi \leftrightarrow \psi $
does not allow us (as we are used from classical logic) to substitute an arbitrary occurrences of
$\varphi \leftrightarrow \psi $
does not allow us (as we are used from classical logic) to substitute an arbitrary occurrences of
![]() $\varphi $
with
$\varphi $
with
![]() $\psi $
within a larger formula.
$\psi $
within a larger formula.
For this reason, we define the following abbreviations, which we call strong implication and strong bi-implication, respectively.Footnote 3
-
•
 $\varphi \Rightarrow \psi \;\;\; \text {abbreviates} \;\;\; ( \varphi \rightarrow \psi ) \land ( {\sim }\psi \rightarrow {\sim }\varphi ). $
$\varphi \Rightarrow \psi \;\;\; \text {abbreviates} \;\;\; ( \varphi \rightarrow \psi ) \land ( {\sim }\psi \rightarrow {\sim }\varphi ). $
-
•
 $\varphi \Leftrightarrow \psi \;\;\; \text {abbreviates} \;\;\; (\varphi \leftrightarrow \psi ) \land ( {\sim } \varphi \leftrightarrow {\sim } \psi ).$
$\varphi \Leftrightarrow \psi \;\;\; \text {abbreviates} \;\;\; (\varphi \leftrightarrow \psi ) \land ( {\sim } \varphi \leftrightarrow {\sim } \psi ).$
In particular, if
![]() $\varphi \Leftrightarrow \psi $
is true then any occurrence of
$\varphi \Leftrightarrow \psi $
is true then any occurrence of
![]() $\varphi $
may be substituted with
$\varphi $
may be substituted with
![]() $\psi $
, and vice versa. The distinction between regular and strong implication and bi-implication will play a crucial role in the correct formulation of the axioms.
$\psi $
, and vice versa. The distinction between regular and strong implication and bi-implication will play a crucial role in the correct formulation of the axioms.
Next, following up on Remark 2.5 we define classical negation as follows:
-
•
 $\neg \varphi \;\;\; \text {abbreviates} \;\;\; \varphi \rightarrow \bot .$
$\neg \varphi \;\;\; \text {abbreviates} \;\;\; \varphi \rightarrow \bot .$
One can easily check that
![]() $\mathcal {M} \models ^T \lnot \varphi $
iff
$\mathcal {M} \models ^T \lnot \varphi $
iff
![]() $\mathcal {M} \not \models ^T \varphi $
while
$\mathcal {M} \not \models ^T \varphi $
while
![]() $\mathcal {M} \models ^F \lnot \varphi $
iff
$\mathcal {M} \models ^F \lnot \varphi $
iff
![]() $\mathcal {M} \models ^T \varphi $
. So in a model
$\mathcal {M} \models ^T \varphi $
. So in a model
![]() $\mathcal {M}$
,
$\mathcal {M}$
,
![]() $\lnot \varphi $
can be either true and not false (when
$\lnot \varphi $
can be either true and not false (when
![]() $\mathcal {M} \models ^T \varphi $
) or false and not true (when
$\mathcal {M} \models ^T \varphi $
) or false and not true (when
![]() $\mathcal {M} \not \models ^T \varphi $
). Using classical negation as a defined notion we can talk about presence and absence of truth and falsity (and, more generally, specify the truth value of a formula) from within the system. We will use the following important abbreviations:
$\mathcal {M} \not \models ^T \varphi $
). Using classical negation as a defined notion we can talk about presence and absence of truth and falsity (and, more generally, specify the truth value of a formula) from within the system. We will use the following important abbreviations:
-
•
 $! \varphi \;\;\; \text {abbreviates} \;\;\; {\sim } \lnot \varphi. $
$! \varphi \;\;\; \text {abbreviates} \;\;\; {\sim } \lnot \varphi. $
-
•
 $? \varphi \;\;\; \text {abbreviates} \;\;\; \lnot {\sim } \varphi. $
$? \varphi \;\;\; \text {abbreviates} \;\;\; \lnot {\sim } \varphi. $
We think of
![]() $!\varphi $
as presence of truth and
$!\varphi $
as presence of truth and
![]() $?\varphi $
as absence of falsity. The truth tables for
$?\varphi $
as absence of falsity. The truth tables for
![]() ${\sim }, \lnot , !$
and
${\sim }, \lnot , !$
and
![]() $?$
in Table 2 make this clear. Notice that
$?$
in Table 2 make this clear. Notice that
![]() $\lnot \varphi $
,
$\lnot \varphi $
,
![]() $!\varphi $
and
$!\varphi $
and
![]() $?\varphi $
will always have truth value
$?\varphi $
will always have truth value
![]() $1$
or
$1$
or
![]() $0$
. Moreover, the truth value of
$0$
. Moreover, the truth value of
![]() $\lnot \varphi $
and
$\lnot \varphi $
and
![]() $!\varphi $
depends only on whether
$!\varphi $
depends only on whether
![]() $\varphi $
was true in the model, and completely disregards whether
$\varphi $
was true in the model, and completely disregards whether
![]() $\varphi $
was false. Similarly,
$\varphi $
was false. Similarly,
![]() $?\varphi $
depends only on whether
$?\varphi $
depends only on whether
![]() $\varphi $
was false and disregards whether it was true.
$\varphi $
was false and disregards whether it was true.
Table 2 Truth table for
![]() ${\sim }, \lnot , !$
and
${\sim }, \lnot , !$
and
![]() $?$
.
$?$
.

A
![]() $\mathrm {BS4}$
-formula is complete if it can never obtain the truth-value
$\mathrm {BS4}$
-formula is complete if it can never obtain the truth-value
![]() $\mathfrak {n}$
, and consistent if it can never obtain the truth-value
$\mathfrak {n}$
, and consistent if it can never obtain the truth-value
![]() $\mathfrak {b}$
. It is called classical if it is both complete and consistent, i.e., if in every model it has truth-value
$\mathfrak {b}$
. It is called classical if it is both complete and consistent, i.e., if in every model it has truth-value
![]() $1$
or
$1$
or
![]() $0$
. In particular,
$0$
. In particular,
![]() $\lnot \varphi $
,
$\lnot \varphi $
,
![]() $!\varphi $
and
$!\varphi $
and
![]() $?\varphi $
are classical formulas for any
$?\varphi $
are classical formulas for any
![]() $\varphi $
. Notice also that classicality, completeness and consistency can each be expressed within the system, by
$\varphi $
. Notice also that classicality, completeness and consistency can each be expressed within the system, by
![]() $ {!}\varphi \leftrightarrow {?}\varphi $
,
$ {!}\varphi \leftrightarrow {?}\varphi $
,
![]() ${?}\varphi \to {!}\varphi $
and
${?}\varphi \to {!}\varphi $
and
![]() $!\varphi \to ?\varphi $
, respectively. We will use the following abbreviation in accordance to [Reference Avron4]:
$!\varphi \to ?\varphi $
, respectively. We will use the following abbreviation in accordance to [Reference Avron4]:
-
•
 $\circ \varphi \;\;\; \text {abbreviates} \;\;\; {!}\varphi \leftrightarrow {?}\varphi .$
$\circ \varphi \;\;\; \text {abbreviates} \;\;\; {!}\varphi \leftrightarrow {?}\varphi .$
It is easy to see that, taking
![]() $\circ $
as primary rather than
$\circ $
as primary rather than
![]() $\bot $
, we obtain the same logic.
$\bot $
, we obtain the same logic.
2.3. Proof system
A sound and complete proof calculus for
![]() $\mathrm {BS4}$
is presented in [Reference Sano and Omori25]. We use a slightly modified but equivalent version, with the following axioms and rules of inference:
$\mathrm {BS4}$
is presented in [Reference Sano and Omori25]. We use a slightly modified but equivalent version, with the following axioms and rules of inference:
-
• Axioms of classical predicate logic:

-
• Axioms for negation:

-
• The rules of inference:
-
23. From
 $\varphi $
and
$\varphi $
and
 $\varphi \rightarrow \psi $
, infer
$\varphi \rightarrow \psi $
, infer
 $\psi $
(modus ponens).
$\psi $
(modus ponens). -
24. Infer
 $\varphi \rightarrow \forall x\psi $
from
$\varphi \rightarrow \forall x\psi $
from
 $\varphi \rightarrow \psi $
, provided x does not occur free in
$\varphi \rightarrow \psi $
, provided x does not occur free in
 $\varphi .$
$\varphi .$
-
25. Infer
 $\exists x\varphi \rightarrow \psi $
from
$\exists x\varphi \rightarrow \psi $
from
 $\varphi \rightarrow \psi $
, provided x does not occur free in
$\varphi \rightarrow \psi $
, provided x does not occur free in
 $\psi .$
$\psi .$
-
Lemma 2.7. The calculus described above is sound and complete with respect to T/F-semantics.
Proof. This follows by adapting the proof of [Reference Sano and Omori25, corollary 5.15] to refer to
![]() $\bot $
rather than the classicality operator
$\bot $
rather than the classicality operator
![]() $\circ $
as the primary symbol. We leave out the details.
$\circ $
as the primary symbol. We leave out the details.
In practice, we will reason informally within the system
![]() $\mathrm {BS4}$
using arguments formalizable in the calculus. We specifically mention some provable statements concerning defined connectives, which will frequently be needed in later arguments.
$\mathrm {BS4}$
using arguments formalizable in the calculus. We specifically mention some provable statements concerning defined connectives, which will frequently be needed in later arguments.
Lemma 2.8. The following statements are provable in
![]() $\mathrm {BS4}$
:
$\mathrm {BS4}$
:
-
•
 $\varphi \leftrightarrow \; !\varphi .$
$\varphi \leftrightarrow \; !\varphi .$
-
•
 ${\sim } \varphi \leftrightarrow {\sim } ?\varphi .$
${\sim } \varphi \leftrightarrow {\sim } ?\varphi .$
(
 $!$
talks only about truth and
$!$
talks only about truth and
 $?$
only about falsity.)
$?$
only about falsity.) -
•
 $x=y\rightarrow ( \varphi (x)\Leftrightarrow \varphi (y)).$
$x=y\rightarrow ( \varphi (x)\Leftrightarrow \varphi (y)).$
(A true equality allows us to interchange terms.)
-
•
 ${\circ \varphi }\;\; \to \;\; ((\varphi \Leftrightarrow \; !\varphi ) \land (\varphi \Leftrightarrow \; ?\varphi ) \land ({\sim } \varphi \Leftrightarrow \lnot \varphi )).$
${\circ \varphi }\;\; \to \;\; ((\varphi \Leftrightarrow \; !\varphi ) \land (\varphi \Leftrightarrow \; ?\varphi ) \land ({\sim } \varphi \Leftrightarrow \lnot \varphi )).$
-
•
 ${\circ \varphi \land \circ \psi } \;\; \to \;\;( (\varphi \to \psi ) \Leftrightarrow (\varphi \Rightarrow \psi )).$
${\circ \varphi \land \circ \psi } \;\; \to \;\;( (\varphi \to \psi ) \Leftrightarrow (\varphi \Rightarrow \psi )).$
(For classical formulas there is no distinctions between strong and weak implication, nor between native and classical negation, and
 $!$
and
$!$
and
 $?$
may be omitted.)
$?$
may be omitted.)
3. Non-classical sets
Before delving into the axioms, it is helpful to think about the concept of a set in a paraconsistent and paracomplete setting from a naive point of view. In classical ZFC, a set x is identified with the class of its elements
![]() $ \{y : y \in x\}$
and divides the entire universe in two parts: those y that are in x, and those y that are not in x. In the context of paraconsistent and paracomplete logic, we can have a situation where a set y is both in x and not in x, or a situation where y is neither in x, nor is it the case that y is not in x.
$ \{y : y \in x\}$
and divides the entire universe in two parts: those y that are in x, and those y that are not in x. In the context of paraconsistent and paracomplete logic, we can have a situation where a set y is both in x and not in x, or a situation where y is neither in x, nor is it the case that y is not in x.
Therefore, it seems natural to identify a set x with the pair consisting of a positive extension (those y for which “
![]() $y \in x$
” is true) and a negative extension (those y for which “
$y \in x$
” is true) and a negative extension (those y for which “
![]() $y\in x$
” is false), without the added requirement that one be the complement of the other. In fact, it makes sense to call a set consistent if its positive and negative extensions do not intersect, complete if their union is the whole universe, and classical if it is both consistent and complete.
$y\in x$
” is false), without the added requirement that one be the complement of the other. In fact, it makes sense to call a set consistent if its positive and negative extensions do not intersect, complete if their union is the whole universe, and classical if it is both consistent and complete.
There is, however, an asymmetry here: the positive extension is a set, while the negative extension is a proper class. Therefore, it turns out to be more appropriate to talk about the complement of the negative extension, i.e., those y for which the statement “
![]() $y \in x$
” is not false (or, equivalently for which the statement “
$y \in x$
” is not false (or, equivalently for which the statement “
![]() $y \notin x$
” is not true). We will refer to this collection as the ?-extension of x.
$y \notin x$
” is not true). We will refer to this collection as the ?-extension of x.
Although we have just referred to statements being true or false, which are seemingly meta-theoretic notions, recall that the operators
![]() $!$
and
$!$
and
![]() $?$
allow us to discuss truth and falsity from within the system as well. In particular,
$?$
allow us to discuss truth and falsity from within the system as well. In particular,
![]() $!(y\in x)$
is true if and only if
$!(y\in x)$
is true if and only if
![]() $y\in x$
is true, and
$y\in x$
is true, and
![]() $?(y \in x)$
is true if and only if
$?(y \in x)$
is true if and only if
![]() $y \in x$
is not false. This motivates the following:
$y \in x$
is not false. This motivates the following:
Definition 3.1. Let
![]() $x$
be a set:
$x$
be a set:
-
• The !-extension of
 $x$
is
$x$
is  $$ \begin{align*}x^! := \{y \;: \; \; !(y \in x)\}.\end{align*} $$
$$ \begin{align*}x^! := \{y \;: \; \; !(y \in x)\}.\end{align*} $$
-
• The ?-extension of
 $x$
is
$x$
is  $$ \begin{align*}x^? := \{y \; :\; \; ?(y \in x)\}.\end{align*} $$
$$ \begin{align*}x^? := \{y \; :\; \; ?(y \in x)\}.\end{align*} $$
For the time being, it is not clear that the above collections describe sets and not proper classes, but we shall set up the axiomatic framework in such a way that if x is a set, then both
![]() $x^!$
and
$x^!$
and
![]() $x^?$
are sets. The four boolean combinations of
$x^?$
are sets. The four boolean combinations of
![]() $x^!$
and
$x^!$
and
![]() $x^?$
determine the classes consisting of all y for which the statement “
$x^?$
determine the classes consisting of all y for which the statement “
![]() $y \in x$
” has one of the four possible truth-values, as visualized in Figure 1.
$y \in x$
” has one of the four possible truth-values, as visualized in Figure 1.

Fig. 1 The four truth values of “
![]() $y \in x$
” depending on the boolean combination of
$y \in x$
” depending on the boolean combination of
![]() $x^!$
and
$x^!$
and
![]() $x^?$
.
$x^?$
.
We remark that the property of sets being complete, consistent and classical can be expressed within the system. In fact the following holds:
-
• x is complete iff
 $\forall y \; (y \in x^? \to y \in x^!).$
$\forall y \; (y \in x^? \to y \in x^!).$
-
• x is consistent iff
 $\forall y \; (y \in x^! \to y \in x^?).$
$\forall y \; (y \in x^! \to y \in x^?).$
-
• x is classical iff
 $\forall y \; (y \in x^! \leftrightarrow y \in x^?).$
$\forall y \; (y \in x^! \leftrightarrow y \in x^?).$
Classical sets behave as we are used to in
![]() ${\mathrm {ZFC}}$
, i.e., the
${\mathrm {ZFC}}$
, i.e., the
![]() $!$
-extension is exactly the
$!$
-extension is exactly the
![]() $?$
-extension, and consequently it does not matter whether we consider the native negation
$?$
-extension, and consequently it does not matter whether we consider the native negation
![]() $\sim $
or classical negation
$\sim $
or classical negation
![]() $\neg $
, nor whether we use
$\neg $
, nor whether we use
![]() $\to $
or
$\to $
or
![]() $\Rightarrow $
. It is not hard to see that
$\Rightarrow $
. It is not hard to see that
![]() $x^!$
and
$x^!$
and
![]() $x^?$
are themselves classical.
$x^?$
are themselves classical.
Let us now look more closely at the notion of equality of two sets and the extensionality principle. In ZFC, two sets are equal precisely if the classes of their elements are the same; equivalently, two sets are different precisely if one set contains an element which the other does not, or vice versa.
In our setting,
![]() $x=y$
and
$x=y$
and
![]() $x \neq y$
Footnote
5
could both be true statements or it could happen that neither
$x \neq y$
Footnote
5
could both be true statements or it could happen that neither
![]() $x=y$
nor
$x=y$
nor
![]() $x \neq y$
are true statements. But we still require that “
$x \neq y$
are true statements. But we still require that “
![]() $x=y$
” express the idea that x and y are the same set-theoretic objects, meaning that both its !-extension and ?-extension must be the same. Likewise, we still want “
$x=y$
” express the idea that x and y are the same set-theoretic objects, meaning that both its !-extension and ?-extension must be the same. Likewise, we still want “
![]() $x \neq y$
” to express that there is something in x which is not in y, or vice versa. So the guiding principle behind the extensionality axiom must be the following:
$x \neq y$
” to express that there is something in x which is not in y, or vice versa. So the guiding principle behind the extensionality axiom must be the following:
-
•
 $x = y \;\; \leftrightarrow \;\; (x^! = y^! \land x^? = y^?),$
$x = y \;\; \leftrightarrow \;\; (x^! = y^! \land x^? = y^?),$
-
•
 $x \neq y \;\; \leftrightarrow \;\; \exists z ((z \in x \land z\notin y) \lor (z \in y \land z\notin x)).$
$x \neq y \;\; \leftrightarrow \;\; \exists z ((z \in x \land z\notin y) \lor (z \in y \land z\notin x)).$
Figure 2 illustrates the situation in which two sets x and y are equal (because their !-extensions and ?-extensions coincide) but also unequal (because there is a set z such that
![]() $z \in x$
but
$z \in x$
but
![]() $z \notin y$
). In fact, if x is any inconsistent set, then
$z \notin y$
). In fact, if x is any inconsistent set, then
![]() $x=x$
and
$x=x$
and
![]() $x\neq x$
.
$x\neq x$
.

Fig. 2
![]() $x=y$
and
$x=y$
and
![]() $x \neq y$
.
$x \neq y$
.
It is customary in set theory to use notation such as
![]() $\{y : \varphi (y)\}$
to refer to the set (if it exists) of all objects y satisfying property
$\{y : \varphi (y)\}$
to refer to the set (if it exists) of all objects y satisfying property
![]() $\varphi $
. In our setting, where a set is determined by its !-extension and ?-extension, it makes sense to agree on the following:
$\varphi $
. In our setting, where a set is determined by its !-extension and ?-extension, it makes sense to agree on the following:
Convention 3.2.
The use of the strong implication means that
![]() $x^!$
is the set of all y for which
$x^!$
is the set of all y for which
![]() $\varphi (y)$
is true, and
$\varphi (y)$
is true, and
![]() $x^?$
is the set of all y for which
$x^?$
is the set of all y for which
![]() $ \varphi (y)$
is not false.
$ \varphi (y)$
is not false.
Now it should be clear why the system under consideration cannot avoid Russell’s paradox, since
![]() $R := \{x : \lnot (x \in x)\}$
cannot be a set by the usual argument. Likewise, the universe of all sets does not form a set.
$R := \{x : \lnot (x \in x)\}$
cannot be a set by the usual argument. Likewise, the universe of all sets does not form a set.
A last point of subtlety should be discussed: how do we understand notation such as
![]() $\{u\}$
, for a set u? One might initially assume that this is
$\{u\}$
, for a set u? One might initially assume that this is
![]() $\{y : y = u\}$
(referring to Convention 3.2). However, a closer look reveals the following: if
$\{y : y = u\}$
(referring to Convention 3.2). However, a closer look reveals the following: if
![]() $\{y : y = u\}$
is a set, then we would like its
$\{y : y = u\}$
is a set, then we would like its
![]() $?$
-extension to also be a set. However, a bit of work following the definitions shows that this
$?$
-extension to also be a set. However, a bit of work following the definitions shows that this
![]() $?$
-extension would be the collection of all sets a such that
$?$
-extension would be the collection of all sets a such that
![]() $a^! \subseteq u^?$
and
$a^! \subseteq u^?$
and
![]() $u^! \subseteq a^?$
. Since there is no upper bound on
$u^! \subseteq a^?$
. Since there is no upper bound on
![]() $a^?$
, it would seem (following classical intuition) that there are class-many sets a satisfying this condition, meaning that the ?-extension of this set is, in fact, a proper class.Footnote
6
$a^?$
, it would seem (following classical intuition) that there are class-many sets a satisfying this condition, meaning that the ?-extension of this set is, in fact, a proper class.Footnote
6
Instead, we opt for the following:
Convention 3.3. If u is a set, then
![]() $\{u\}$
is the set
$\{u\}$
is the set
![]() $\{y \: : \: !(y=u)\}$
; and more generally if
$\{y \: : \: !(y=u)\}$
; and more generally if
![]() $u_0, \dots , u_n$
are sets, then
$u_0, \dots , u_n$
are sets, then
![]() $\{u_0, \dots , u_n\}$
is the set
$\{u_0, \dots , u_n\}$
is the set
![]() $ \{y \:: \: \: !(y=u_0) \lor \dots \lor !(y=u_n)\}$
. This is always a classical set whose !-extension and ?-extension are exactly the finite set consisting of the elements in question.
$ \{y \:: \: \: !(y=u_0) \lor \dots \lor !(y=u_n)\}$
. This is always a classical set whose !-extension and ?-extension are exactly the finite set consisting of the elements in question.
A similar phenomenon occurs with other set-theoretic operations, most notably the power set operation.
4. The theory
 $\mathrm {PZFC}$
$\mathrm {PZFC}$
We now introduce the intermediate system
![]() $\mathrm {PZFC}$
by carefully analysing the standard axioms of set theory and generalizing them in accordance with the intuition described in the previous section.
$\mathrm {PZFC}$
by carefully analysing the standard axioms of set theory and generalizing them in accordance with the intuition described in the previous section.
4.1. Extensionality
The most essential axiom required to sustain an ontology of sets is the following:
Why do we choose strong rather than weak bi-implications? For the first one the choice is clear: extensionality seeks to define the meaning of the expression “
![]() $x=y$
” in terms of the elements of x and y, and this needs to talk both about truth and falsity. Notice that the use of this strong implications allows us to interchange the expression “
$x=y$
” in terms of the elements of x and y, and this needs to talk both about truth and falsity. Notice that the use of this strong implications allows us to interchange the expression “
![]() $x=y$
” with the expression on the right-hand side within any given formula.
$x=y$
” with the expression on the right-hand side within any given formula.
The second bi-implication is more interesting: recall that we want
![]() $x=y$
to express that both the !-extension and ?-extension of x and y coincide. The statement with a weak implication
$x=y$
to express that both the !-extension and ?-extension of x and y coincide. The statement with a weak implication
![]() $ \forall z(z\in x\leftrightarrow z\in y))$
would only say that the !-extensions coincide.
$ \forall z(z\in x\leftrightarrow z\in y))$
would only say that the !-extensions coincide.
At this point it is instructive to define subsets:
Again, the use of the strong implication makes sure that
![]() $x \subseteq y$
says that both the !-extensions and ?-extensions of x are included in that of y. In particular, the Axiom of Extensionality can now be reformulated as follows:
$x \subseteq y$
says that both the !-extensions and ?-extensions of x are included in that of y. In particular, the Axiom of Extensionality can now be reformulated as follows:
![]() $\forall x \forall y ( x=y \; \Leftrightarrow \; x \subseteq y \land y \subseteq x)$
.
$\forall x \forall y ( x=y \; \Leftrightarrow \; x \subseteq y \land y \subseteq x)$
.
4.2. Comprehension
The next most important axiom is Comprehension.Footnote 7
The use of the strong implication means that, in accordance to Convention 3.2, for any set u, the following is also a set:
4.3. Classical Supersets
The development of our theory is greatly simplified by considering an axiom postulating that every set is contained within a classical superset. In Section 3 we said that a set was classical if its
![]() $!$
-extension and
$!$
-extension and
![]() $?$
-extension are the same. We do not know yet whether !-extensions and
$?$
-extension are the same. We do not know yet whether !-extensions and
![]() $?$
-extensions are sets, but we can express that C is a classical set with the sentence
$?$
-extensions are sets, but we can express that C is a classical set with the sentence
![]() $\forall y \; {\circ } (y \in C)$
, where
$\forall y \; {\circ } (y \in C)$
, where
![]() $\circ $
is the classicality operator defined in Section 2.2.
$\circ $
is the classicality operator defined in Section 2.2.
This axiom is technically superfluous, since it can be proved to follow from the remaining axioms in a roundabout way. However, adopting it at this stage allows us to frame the remaining axioms, and the theory in general, in a more intuitive way. In particular, it allows us to confirm several properties of sets we postulated earlier.
Lemma 4.1 (Cl. Superset + Comprehension)
If
![]() $x$
is a set, then its !-extension and ?-extension are sets.
$x$
is a set, then its !-extension and ?-extension are sets.
Proof. Let C be a classical set with
![]() $x \subseteq C$
. Then
$x \subseteq C$
. Then
are both sets by the Comprehension axiom.
Since
![]() $x^!$
and
$x^!$
and
![]() $x^?$
are classical, a posteriori we see that a particularly convenient classical superset of x is obtained by considering
$x^?$
are classical, a posteriori we see that a particularly convenient classical superset of x is obtained by considering
![]() $x^! \cup x^?$
. This is the smallest classical set containing x and we will call this the realm of x:
$x^! \cup x^?$
. This is the smallest classical set containing x and we will call this the realm of x:
The next lemma elaborates on the meaning of equality and the subset relation between sets.
Lemma 4.2 (Cl. Superset + Extensionality + Comprehension)
-
1.
 $x \subseteq y \;\; \leftrightarrow \;\; x^! \subseteq y^! \land x^? \subseteq y^?, $
$x \subseteq y \;\; \leftrightarrow \;\; x^! \subseteq y^! \land x^? \subseteq y^?, $
-
2.
 $x \not \subseteq y \;\; \leftrightarrow \;\; \exists z (z \in x \land z\notin y) \;\; \leftrightarrow \;\; x^! \not \subseteq y^?,$
$x \not \subseteq y \;\; \leftrightarrow \;\; \exists z (z \in x \land z\notin y) \;\; \leftrightarrow \;\; x^! \not \subseteq y^?,$
-
3.
 $?(x \subseteq y) \;\; \leftrightarrow \;\; x^! \subseteq y^?,$
$?(x \subseteq y) \;\; \leftrightarrow \;\; x^! \subseteq y^?,$
-
4.
 $x = y \;\; \leftrightarrow \;\; x^! = y^! \land x^? = y^?,$
$x = y \;\; \leftrightarrow \;\; x^! = y^! \land x^? = y^?,$
-
5.
 $x \neq y \;\; \leftrightarrow \;\; \exists z ((z \in x \land z\notin y) \lor (z \in y \land z\notin x)) \;\; \leftrightarrow \;\; x^! \not \subseteq y^? \lor y^! \not \subseteq x^?,$
$x \neq y \;\; \leftrightarrow \;\; \exists z ((z \in x \land z\notin y) \lor (z \in y \land z\notin x)) \;\; \leftrightarrow \;\; x^! \not \subseteq y^? \lor y^! \not \subseteq x^?,$
-
6.
 $?(x = y) \;\; \leftrightarrow \;\; x^! \subseteq y^? \land y^! \subseteq x^?.$
$?(x = y) \;\; \leftrightarrow \;\; x^! \subseteq y^? \land y^! \subseteq x^?.$
Proof. We will provide detailed proofs of 1 and 2 in order to illustrate how reasoning within
![]() $\mathrm {BS4}$
works. The remaining proofs are left to the reader.
$\mathrm {BS4}$
works. The remaining proofs are left to the reader.
-
1. We have the following sequence of
 $\mathrm {BS4}$
-provable bi-implications:
$\mathrm {BS4}$
-provable bi-implications: $x \subseteq y$
$x \subseteq y$
 $\Leftrightarrow $
$\Leftrightarrow $
 $ \forall z ( (z \in x \to z \in y) \land ({\sim } (z \in y) \to {\sim } (z \in x))$
$ \forall z ( (z \in x \to z \in y) \land ({\sim } (z \in y) \to {\sim } (z \in x))$
 $\stackrel {(*)}{\leftrightarrow }$
$\stackrel {(*)}{\leftrightarrow }$
 $\forall z ( (!(z \in x) \to !(z \in y)) \land (? (z \in x) \to ? (z \in y))$
$\forall z ( (!(z \in x) \to !(z \in y)) \land (? (z \in x) \to ? (z \in y))$
 $\stackrel {(**)}{\Leftrightarrow }$
$\stackrel {(**)}{\Leftrightarrow }$
 $ \forall z (( z \in x^! \Rightarrow z \in y^!) \land (z \in x^? \Rightarrow z \in y^?)$
$ \forall z (( z \in x^! \Rightarrow z \in y^!) \land (z \in x^? \Rightarrow z \in y^?)$
 $ \Leftrightarrow $
$ \Leftrightarrow $
 $x^! \subseteq y^! \land x^? \subseteq y^? $
.
$x^! \subseteq y^! \land x^? \subseteq y^? $
.Here
 $(*)$
is due to the truth-functional definition of the implications and the
$(*)$
is due to the truth-functional definition of the implications and the
 $!$
and
$!$
and
 $?$
operators, while
$?$
operators, while
 $(**)$
is due to the definition of
$(**)$
is due to the definition of
 $x^!, y^!, x^?, y^?$
and the fact that these sets are classical (so
$x^!, y^!, x^?, y^?$
and the fact that these sets are classical (so
 $\to $
and
$\to $
and
 $\Rightarrow $
are interchangeable). The first and last strong bi-implication is the definition of
$\Rightarrow $
are interchangeable). The first and last strong bi-implication is the definition of
 $\subseteq $
.
$\subseteq $
. -
2. We have the following sequence of bi-implications:
 ${\sim } (x \subseteq y)$
${\sim } (x \subseteq y)$
 $\Leftrightarrow $
$\Leftrightarrow $
 $ {\sim } \forall z (z \in x \Rightarrow z \in y) $
$ {\sim } \forall z (z \in x \Rightarrow z \in y) $
 $\Leftrightarrow $
$\Leftrightarrow $
 $ \exists z {\sim } (z \in x \Rightarrow z\in y) $
$ \exists z {\sim } (z \in x \Rightarrow z\in y) $
 $\stackrel {(*)}{\leftrightarrow }$
$\stackrel {(*)}{\leftrightarrow }$
 $ \exists z (z \in x \land z \notin y), $
$ \exists z (z \in x \land z \notin y), $
where
 $(*)$
is because we are only looking at the truth condition of
$(*)$
is because we are only looking at the truth condition of
 $\Rightarrow $
. Further:
$\Rightarrow $
. Further: $ \dots $
$ \dots $
 $\stackrel {(**)}{\leftrightarrow }$
$\stackrel {(**)}{\leftrightarrow }$
 $ \exists z (z \in x^! \land z \notin y^?) $
$ \exists z (z \in x^! \land z \notin y^?) $
 $\Leftrightarrow $
$\Leftrightarrow $
 $ x^! \not \subseteq y^?$
, where
$ x^! \not \subseteq y^?$
, where
 $(**)$
is again due to the fact that
$(**)$
is again due to the fact that
 $!$
refers to truth and
$!$
refers to truth and
 $?$
to falsity.
$?$
to falsity.
4.4. Replacement
The next axiom we consider is Replacement.Footnote
8
In
![]() ${\mathrm {ZFC}}$
the Replacement axiom tells us that the image of a set under a class function is itself a set. It is not immediately clear how to generalize class function. As a guiding principle we rely on the intuition that in practice, mathematicians apply Replacement when there is a pre-determined recipe by which each element of a given set is replaced by another element in a non-ambiguous way. We will call such a recipe an operation:
${\mathrm {ZFC}}$
the Replacement axiom tells us that the image of a set under a class function is itself a set. It is not immediately clear how to generalize class function. As a guiding principle we rely on the intuition that in practice, mathematicians apply Replacement when there is a pre-determined recipe by which each element of a given set is replaced by another element in a non-ambiguous way. We will call such a recipe an operation:
Definition 4.3. An operation is a formula
![]() $\varphi (x,y)$
such that:
$\varphi (x,y)$
such that:
-
1.
 $\varphi $
is classical, and
$\varphi $
is classical, and -
2.
 $\forall x \exists y(\varphi (x,y) \; \land \; \forall z \;(\varphi (x,z) \to !(y=z))$
.
$\forall x \exists y(\varphi (x,y) \; \land \; \forall z \;(\varphi (x,z) \to !(y=z))$
.
The requirement on
![]() $\varphi $
to be a classical formula reflects the notion that an operation describes a well-defined recipe for replacing input x with output y. Likewise, the “
$\varphi $
to be a classical formula reflects the notion that an operation describes a well-defined recipe for replacing input x with output y. Likewise, the “
![]() $!$
” makes sure that the statement expressing that “every input has at most one output” is a classical sentence, since we would not know how to interpret a situation in which this statement is both true and false.Footnote
9
$!$
” makes sure that the statement expressing that “every input has at most one output” is a classical sentence, since we would not know how to interpret a situation in which this statement is both true and false.Footnote
9
![]() $\boldsymbol {\forall x \exists y \forall z (z \in y \Leftrightarrow \exists w (w \in x \land \varphi (w,z))).}$
$\boldsymbol {\forall x \exists y \forall z (z \in y \Leftrightarrow \exists w (w \in x \land \varphi (w,z))).}$
After adopting this axiom, we can treat an operation
![]() $\varphi $
as a class function F, and use notation such as
$\varphi $
as a class function F, and use notation such as
where X is a set.
4.5. Pairing
The Pairing axiom is normally needed to get set-theory ‘started’ and allow the definition of ordered pairs, relations, functions, and so on. Recall the discussion in Convention 3.3 that we want notation such as
![]() $\{u,v\}$
to stand for the classical set whose !-extension and ?-extension contain exactly the two objects u and v. This motivates the following:
$\{u,v\}$
to stand for the classical set whose !-extension and ?-extension contain exactly the two objects u and v. This motivates the following:
As discussed in Section 3, this falls in line with the intuition of an unordered pair
![]() $\{u,v\}$
as a classical set. Referring to Lemma 4.2(5) and (6), we now see that, if we had used the definition
$\{u,v\}$
as a classical set. Referring to Lemma 4.2(5) and (6), we now see that, if we had used the definition
![]() $\{x : x=u \lor x=v\}$
for the unordered pair, then the ?-extension would have been the collection of all x such that
$\{x : x=u \lor x=v\}$
for the unordered pair, then the ?-extension would have been the collection of all x such that
![]() $x^! \subseteq u^?$
and
$x^! \subseteq u^?$
and
![]() $u^! \subseteq x^?$
, or
$u^! \subseteq x^?$
, or
![]() $x^! \subseteq v^?$
and
$x^! \subseteq v^?$
and
![]() $v^! \subseteq x^?$
. This is problematic since there is no upper bound on the size of
$v^! \subseteq x^?$
. This is problematic since there is no upper bound on the size of
![]() $x^?$
. Indeed, in Lemma 6.6 we will prove that if there exists at least one incomplete set, then
$x^?$
. Indeed, in Lemma 6.6 we will prove that if there exists at least one incomplete set, then
![]() $\{x : x=u \lor x=v\}$
is a proper class.
$\{x : x=u \lor x=v\}$
is a proper class.
4.6. Power Set
Suppose u is any set and we look at
![]() $\mathscr {P}(u) := \{x : x \subseteq u\}$
. Referring to Lemma 4.2, we again notice that
$\mathscr {P}(u) := \{x : x \subseteq u\}$
. Referring to Lemma 4.2, we again notice that
![]() $?(x\subseteq u) \;\; \leftrightarrow \;\; x^! \subseteq u^?$
, so the ?-extension of
$?(x\subseteq u) \;\; \leftrightarrow \;\; x^! \subseteq u^?$
, so the ?-extension of
![]() $\mathscr {P}(u)$
consists of sets x such that
$\mathscr {P}(u)$
consists of sets x such that
![]() $x^! \subseteq u^?$
, but with no special requirement on
$x^! \subseteq u^?$
, but with no special requirement on
![]() $x^?$
. Again, it should be intuitively clear that there is a proper class of possible x satisfying this requirement. As with Pairing, we instead opt for the following definition:
$x^?$
. Again, it should be intuitively clear that there is a proper class of possible x satisfying this requirement. As with Pairing, we instead opt for the following definition:
Now
![]() $\mathscr {P}^!(u)$
is a classical set containing exactly those sets x for which
$\mathscr {P}^!(u)$
is a classical set containing exactly those sets x for which
![]() $x^! \subseteq u^!$
and
$x^! \subseteq u^!$
and
![]() $x^? \subseteq u^?$
. This motivates the axiom:
$x^? \subseteq u^?$
. This motivates the axiom:
4.7. The remaining axioms
We will now list the remaining four axioms since they are, mostly, non-problematic.
After adopting this axiom we can use the abbreviation
![]() $\bigcup u := \{x : \exists z \: (y \in z \land z\in u)\}$
, and this coincides with Convention 3.2.
$\bigcup u := \{x : \exists z \: (y \in z \land z\in u)\}$
, and this coincides with Convention 3.2.
This axiom could more properly be called “set induction schema”. It allows us to view the universe as being constructed by transfinite recursion, where each new level consists of those x for which the realm
![]() $x^! \cup x^?$
is a subset of the previous level. See [Reference Oddsson19, sec. 4.12] for details.
$x^! \cup x^?$
is a subset of the previous level. See [Reference Oddsson19, sec. 4.12] for details.
This is a standard formulation of the axiom of choice, however, it requires the concept of a function which we have not properly defined yet. This will be done in Section 6, however the current axiom is not required for that definition.
5. The anti-classicality axiom and
 ${\mathrm {BZFC}}$
${\mathrm {BZFC}}$
None of the axioms of
![]() $\mathrm {PZFC}$
guarantee the existence of an inconsistent or incomplete set. In fact, the following should be clear:
$\mathrm {PZFC}$
guarantee the existence of an inconsistent or incomplete set. In fact, the following should be clear:
Theorem 5.1.
![]() $\mathrm {PZFC} \: + \: \forall x (x^!=x^?)$
is equivalent to
$\mathrm {PZFC} \: + \: \forall x (x^!=x^?)$
is equivalent to
![]() ${\mathrm {ZFC}}$
.
${\mathrm {ZFC}}$
.
Proof. If every set is classical, then there is no distinction between
![]() ${\sim }$
and
${\sim }$
and
![]() $\lnot $
, nor between
$\lnot $
, nor between
![]() $\to $
and
$\to $
and
![]() $\Rightarrow $
. Likewise,
$\Rightarrow $
. Likewise,
![]() $!$
and
$!$
and
![]() $?$
can be discarded. The Classical Superset Axiom is trivial. So what remains of
$?$
can be discarded. The Classical Superset Axiom is trivial. So what remains of
![]() $\mathrm {PZFC}$
is precisely the collection of
$\mathrm {PZFC}$
is precisely the collection of
![]() ${\mathrm {ZFC}}$
axioms.
${\mathrm {ZFC}}$
axioms.
Since we are interested in theories with non-classical sets, we would like to adopt an axiom postulating their existence. Now we seem to be faced with a choice: exactly which non-classical sets do we want to postulate the existence of? Following a conservative approach, we might want to require only that at least one inconsistent and at least one incomplete set exists:
On the other hand, a maximality approach might lead us to postulate the existence of as many non-classical sets as possible, i.e., for any classical sets u, v, there exists a set x whose !-extension is exactly u and whose ?-extension is exactly v
An unexpected, yet surprisingly simple result now shows that in the presence of the other
![]() $\mathrm {PZFC}$
-axioms, any choice we make is equivalent, since the weakest of them (the conservative one) already implies the strongest (the maximizing one). Indeed, this can be viewed as a very central theorem on which the rest of our theory hinges in a crucial way.
$\mathrm {PZFC}$
-axioms, any choice we make is equivalent, since the weakest of them (the conservative one) already implies the strongest (the maximizing one). Indeed, this can be viewed as a very central theorem on which the rest of our theory hinges in a crucial way.
Theorem 5.2 (
 $\mathrm {PZFC}$
)
$\mathrm {PZFC}$
)
Suppose there is an inconsistent and an incomplete set. Then for any classical sets
![]() $u,v$
, there is x such that
$u,v$
, there is x such that
![]() $x^!=u$
and
$x^!=u$
and
![]() $x^?=v$
.
$x^?=v$
.
Proof. From the assumption we have
![]() $a \in b \land a \notin b$
for some sets
$a \in b \land a \notin b$
for some sets
![]() $a,b$
and also
$a,b$
and also
![]() $\lnot (c \in d \lor c \notin d)$
for some sets
$\lnot (c \in d \lor c \notin d)$
for some sets
![]() $c,d$
. Let us introduce the following abbreviation:
$c,d$
. Let us introduce the following abbreviation:
Note that
![]() $\phi _{\mathfrak {b}}$
and
$\phi _{\mathfrak {b}}$
and
![]() ${\sim } \phi _{\mathfrak {b}}$
are both true, while
${\sim } \phi _{\mathfrak {b}}$
are both true, while
![]() $\phi _{\mathfrak {n}}$
and
$\phi _{\mathfrak {n}}$
and
![]() ${\sim } \phi _{\mathfrak {n}}$
are both not true.
${\sim } \phi _{\mathfrak {n}}$
are both not true.
Let u and v be classical sets and define
Then we have:
-
•
 $z\in x $
$z\in x $
 $\;\;\leftrightarrow \;\;$
$\;\;\leftrightarrow \;\;$
 $z \in (u \cap v) \; \lor \;(z \in (u\setminus v) \land \phi _{\mathfrak {b}}) \; \lor \; (z \in (v\setminus u) \land \phi _{\mathfrak {n}}) $
$z \in (u \cap v) \; \lor \;(z \in (u\setminus v) \land \phi _{\mathfrak {b}}) \; \lor \; (z \in (v\setminus u) \land \phi _{\mathfrak {n}}) $
 $\;\;\leftrightarrow \;\;$
$\;\;\leftrightarrow \;\;$
 $z \in (u \cap v) \; \lor \;z \in (u\setminus v) $
$z \in (u \cap v) \; \lor \;z \in (u\setminus v) $
 $\;\;\leftrightarrow \;\;$
$\;\;\leftrightarrow \;\;$
 $z \in u$
, and
$z \in u$
, and -
•
 $z\notin x $
$z\notin x $
 $\;\;\leftrightarrow \;\;$
$\;\;\leftrightarrow \;\;$
 $z \notin (u \cap v) \; \land \;(z \notin (u\setminus v) \lor {\sim } \phi _{\mathfrak {b}}) \; \land \; (z \notin (v\setminus u) \lor {\sim } \phi _{\mathfrak {n}}) $
$z \notin (u \cap v) \; \land \;(z \notin (u\setminus v) \lor {\sim } \phi _{\mathfrak {b}}) \; \land \; (z \notin (v\setminus u) \lor {\sim } \phi _{\mathfrak {n}}) $
 $\;\;\leftrightarrow \;\;$
$\;\;\leftrightarrow \;\;$
 $z \notin (u \cap v) \; \land \; z \notin (v\setminus u) $
$z \notin (u \cap v) \; \land \; z \notin (v\setminus u) $
 $\;\;\leftrightarrow \;\;$
$\;\;\leftrightarrow \;\;$
 $z \notin v. $
$z \notin v. $
It follows that
![]() $z \in x^! \leftrightarrow z \in u$
and
$z \in x^! \leftrightarrow z \in u$
and
![]() $z \in x^? \leftrightarrow z \in v$
. This completes the proof.
$z \in x^? \leftrightarrow z \in v$
. This completes the proof.
Definition 5.3. We let the anti-classicality axiom be the statement:
and consider the system
This is the main axiomatic system under consideration in this paper.
Remark 5.4. Some readers might be interested in a finer distinction and consider a theory that has only incomplete but not inconsistent sets. The corresponding theory would then be
![]() $\mathrm {PZFC} + \exists x (x^? \not \subseteq x^!) + \forall x (x^! \subseteq x^?)$
. A proof similar to the above would then show that this implies that for any classical sets
$\mathrm {PZFC} + \exists x (x^? \not \subseteq x^!) + \forall x (x^! \subseteq x^?)$
. A proof similar to the above would then show that this implies that for any classical sets
![]() $u,v$
with
$u,v$
with
![]() $u \subseteq v$
, there is x such that
$u \subseteq v$
, there is x such that
![]() $x^! = u$
and
$x^! = u$
and
![]() $x^? = v$
. Likewise, if one is interested in a theory that has only inconsistent but not incomplete sets one can look at
$x^? = v$
. Likewise, if one is interested in a theory that has only inconsistent but not incomplete sets one can look at
![]() $\mathrm {PZFC} + \exists x (x^! \not \subseteq x^?) + \forall x (x^? \subseteq x^!)$
. This implies that for any classical sets
$\mathrm {PZFC} + \exists x (x^! \not \subseteq x^?) + \forall x (x^? \subseteq x^!)$
. This implies that for any classical sets
![]() $u,v$
with
$u,v$
with
![]() $v \subseteq u$
, there is x such that
$v \subseteq u$
, there is x such that
![]() $x^! = u$
and
$x^! = u$
and
![]() $x^? = v$
. We will return to this finer distinction in Section 10 in a specific context, but otherwise will not pursue it in detail.
$x^? = v$
. We will return to this finer distinction in Section 10 in a specific context, but otherwise will not pursue it in detail.
As a nice application of
![]() $\mathrm {ACLA}$
, we can show how to internally define truth values in a succinct way. Let
$\mathrm {ACLA}$
, we can show how to internally define truth values in a succinct way. Let
![]() $\Omega := \mathscr {P}^!(\{\varnothing \})$
, i.e., the classical set containing sets x such that
$\Omega := \mathscr {P}^!(\{\varnothing \})$
, i.e., the classical set containing sets x such that
![]() $x^! \subseteq \{\varnothing \}$
and
$x^! \subseteq \{\varnothing \}$
and
![]() $x^? \subseteq \{\varnothing \}$
. There are four possible combinations for such x, and
$x^? \subseteq \{\varnothing \}$
. There are four possible combinations for such x, and
![]() $\mathrm {ACLA}$
guarantees us that all four of them exist, and are members of
$\mathrm {ACLA}$
guarantees us that all four of them exist, and are members of
![]() $\Omega $
. We can give them names as follows:
$\Omega $
. We can give them names as follows:
-
•
 $x := 1\;\;\;\;$
if
$x := 1\;\;\;\;$
if
 $\;\;\;\;x^! = x^? = \{\varnothing \},$
$\;\;\;\;x^! = x^? = \{\varnothing \},$
-
•
 $x:=0\;\;\;\;$
if
$x:=0\;\;\;\;$
if
 $\;\;\;\;x^! = x^? = \varnothing ,$
$\;\;\;\;x^! = x^? = \varnothing ,$
-
•
 $x:=\mathfrak {n}\;\;\;\;$
if
$x:=\mathfrak {n}\;\;\;\;$
if
 $\;\;\;\;x^! = \varnothing $
and
$\;\;\;\;x^! = \varnothing $
and
 $x^? = \{\varnothing \},$
$x^? = \{\varnothing \},$
-
•
 $x:=\mathfrak {b}\;\;\;\;$
if
$x:=\mathfrak {b}\;\;\;\;$
if
 $\;\;\;\;x^! = \{\varnothing \}$
and
$\;\;\;\;x^! = \{\varnothing \}$
and
 $x^? = \varnothing .$
$x^? = \varnothing .$
Then
![]() $\Omega = \{1, \mathfrak {b},\mathfrak {n},0\}$
is called the set of truth values, and for any formula
$\Omega = \{1, \mathfrak {b},\mathfrak {n},0\}$
is called the set of truth values, and for any formula
![]() $\varphi $
, we can define the truth value of
$\varphi $
, we can define the truth value of
![]() $\varphi $
by
$\varphi $
by
We now have that ![]() is true precisely if
is true precisely if
![]() $\varphi $
is true, and false precisely if
$\varphi $
is true, and false precisely if
![]() $\varphi $
is false. In other words, for any formula
$\varphi $
is false. In other words, for any formula
![]() $\varphi $
, from the point of view of the meta-theory, i.e.,
$\varphi $
, from the point of view of the meta-theory, i.e., ![]()
6. Mathematics in
 ${\mathrm {BZFC}}$
${\mathrm {BZFC}}$
In a typical set theory textbook, the introduction of the
![]() ${\mathrm {ZFC}}$
-axioms is usually followed up by developing the tools needed to sustain modern mathematics, e.g., ordered pairs, Cartesian products, relations, functions, induction and recursion, ordinals, cardinals and their arithmetic.
${\mathrm {ZFC}}$
-axioms is usually followed up by developing the tools needed to sustain modern mathematics, e.g., ordered pairs, Cartesian products, relations, functions, induction and recursion, ordinals, cardinals and their arithmetic.
In general, many non-trivial questions arise in the context of
![]() ${\mathrm {BZFC}}$
, such as the exact nature of functions, images and pre-images, cardinality, cardinal arithmetic and much more. Many of these questions deserve separate investigation. In this paper we will present only as much mathematical formalism as is necessary for the subsequent sections. Readers more interested in the foundational results can safely skip to the next Section 7.
${\mathrm {BZFC}}$
, such as the exact nature of functions, images and pre-images, cardinality, cardinal arithmetic and much more. Many of these questions deserve separate investigation. In this paper we will present only as much mathematical formalism as is necessary for the subsequent sections. Readers more interested in the foundational results can safely skip to the next Section 7.
First, we would like to define an ordered pair
![]() $(a,b)$
in such a way that
$(a,b)$
in such a way that
The task is less trivial than it appears, since the falsity condition
![]() $(a,b) \neq (a',b') \; \leftrightarrow \; (a \neq a' \lor b \neq b')$
refers to the native
$(a,b) \neq (a',b') \; \leftrightarrow \; (a \neq a' \lor b \neq b')$
refers to the native
![]() $\neq $
relation which is governed by the extensionality axiom. The Kuratowski pair will not suffice for this purpose since this defines a classical set. But we can define ordered pairs in a round-about way as follows:
$\neq $
relation which is governed by the extensionality axiom. The Kuratowski pair will not suffice for this purpose since this defines a classical set. But we can define ordered pairs in a round-about way as follows:
-
• First, say that the classical ordered pair
 $\left <u,v\right>$
is
$\left <u,v\right>$
is
 $\{\{u\}, \{u,v\}\}$
.
$\{\{u\}, \{u,v\}\}$
. -
• Then, for sets a and b define
 $(a,b) \; := \; \{ \left <x,0\right>: x \in a\} \cup \{ \left <y,1\right>: y \in b\}$
, where
$(a,b) \; := \; \{ \left <x,0\right>: x \in a\} \cup \{ \left <y,1\right>: y \in b\}$
, where
 $0$
is
$0$
is
 $\varnothing $
and
$\varnothing $
and
 $1$
is
$1$
is
 $\{\varnothing \}$
.
$\{\varnothing \}$
.
Here,
![]() $(a,b)$
is essentially the disjoint union of copies of a and b, so the non-classical structure of the sets is unaffected. Showing that this definition indeed satisfies
$(a,b)$
is essentially the disjoint union of copies of a and b, so the non-classical structure of the sets is unaffected. Showing that this definition indeed satisfies
![]() $(*)$
is somewhat lengthy, so we leave the details to the reader. We should note that there are other ways to encode ordered pairs, for example, see [Reference Oddsson19, appendix A]. The exact method is inconsequential as long as condition
$(*)$
is somewhat lengthy, so we leave the details to the reader. We should note that there are other ways to encode ordered pairs, for example, see [Reference Oddsson19, appendix A]. The exact method is inconsequential as long as condition
![]() $(*)$
is fulfilled.
$(*)$
is fulfilled.
The Cartesian product
![]() $A \times B := \{(a,b) : a \in A \land b \in B\}$
is defined as usual. It is easy to see that
$A \times B := \{(a,b) : a \in A \land b \in B\}$
is defined as usual. It is easy to see that
![]() $(A \times B)^! = A^! \times B^!$
and
$(A \times B)^! = A^! \times B^!$
and
![]() $(A\times B)^? = A^? \times B^?$
, so if A and B are classical,
$(A\times B)^? = A^? \times B^?$
, so if A and B are classical,
![]() $A \times B$
is as well. A binary relation between A and B is any
$A \times B$
is as well. A binary relation between A and B is any
![]() $R \subseteq A \times B$
. We note that R may fail to be classical even if A and B are. We can define the domain and range of a relation by stipulating that
$R \subseteq A \times B$
. We note that R may fail to be classical even if A and B are. We can define the domain and range of a relation by stipulating that
![]() $\mathrm {dom}(R) = \{x : \exists y ((x,y) \in R)\}$
and
$\mathrm {dom}(R) = \{x : \exists y ((x,y) \in R)\}$
and
![]() $\mathrm {ran}(R) = \{y : \exists x ((x,y) \in R)\}$
. It then follows that
$\mathrm {ran}(R) = \{y : \exists x ((x,y) \in R)\}$
. It then follows that
![]() $\mathrm {dom}(R) \subseteq A$
and
$\mathrm {dom}(R) \subseteq A$
and
![]() $\mathrm {ran}(R) \subseteq B$
. The domain and range will generally be non-classical if R is non-classical. The following definition deserves special attention:
$\mathrm {ran}(R) \subseteq B$
. The domain and range will generally be non-classical if R is non-classical. The following definition deserves special attention:
Definition 6.1.
![]() $E \subseteq X \times X$
is called an equivalence relation if for all
$E \subseteq X \times X$
is called an equivalence relation if for all
![]() $x,y,z$
in
$x,y,z$
in
![]() $\mathrm{rlm}(X)$
(i.e., in
$\mathrm{rlm}(X)$
(i.e., in
![]() $X^! \cup X^?$
) we have:
$X^! \cup X^?$
) we have:
-
1.
 $(x,x) \in E$
.
$(x,x) \in E$
. -
2.
 $(x,y) \in E \Leftrightarrow (y,x) \in E$
.
$(x,y) \in E \Leftrightarrow (y,x) \in E$
. -
3.
 $(y,z) \in E \; \to \; ((x,y) \in E \Leftrightarrow (x,z) \in E)$
.
$(y,z) \in E \; \to \; ((x,y) \in E \Leftrightarrow (x,z) \in E)$
.
Notice that this tells us more than E being reflexive, symmetric and transitive; in addition, 3 implies that if y and z are E-related, then they are indistinguishable with respect to being E-related to another element x. If we now define the E-equivalence class of an element
![]() $x \in X$
by
$x \in X$
by
we obtain the following:
Lemma 6.2. For all
![]() $x,y \in \mathrm {rlm}(X)$
we have
$x,y \in \mathrm {rlm}(X)$
we have
![]() $(x,y) \in E \; \Leftrightarrow \; [x]_E = [y]_E$
.
$(x,y) \in E \; \Leftrightarrow \; [x]_E = [y]_E$
.
Proof. The positive equivalence
![]() $(x,y) \in E \; \leftrightarrow \; [x]_E = [y]_E$
is obvious. Also, it is clear that if
$(x,y) \in E \; \leftrightarrow \; [x]_E = [y]_E$
is obvious. Also, it is clear that if
![]() $(x,y) \notin E$
, then by definition
$(x,y) \notin E$
, then by definition
![]() $x \notin [y]_E$
, while
$x \notin [y]_E$
, while
![]() $(x,x) \in E$
implies that
$(x,x) \in E$
implies that
![]() $x \in [x]_E$
. Therefore there is a witness x which is in
$x \in [x]_E$
. Therefore there is a witness x which is in
![]() $[x]_E$
and not in
$[x]_E$
and not in
![]() $[y]_E$
, so
$[y]_E$
, so
![]() $[x]_E \neq [y]_E$
by extensionality.
$[x]_E \neq [y]_E$
by extensionality.
Now, suppose
![]() $[x]_E \neq [y]_E$
. Then there exists z such that
$[x]_E \neq [y]_E$
. Then there exists z such that
![]() $z \in [x]_E$
and
$z \in [x]_E$
and
![]() $z \notin [y]_E$
, or vice versa. Without loss assume the former, so by definition we have
$z \notin [y]_E$
, or vice versa. Without loss assume the former, so by definition we have
![]() $(z,x) \in E$
and
$(z,x) \in E$
and
![]() $(z,y) \notin E$
. But now the strong bi-implication in condition 3 gives us
$(z,y) \notin E$
. But now the strong bi-implication in condition 3 gives us
![]() $(x,y) \notin E$
, as required.
$(x,y) \notin E$
, as required.
Next we look at the notion of a function. It is not entirely straightforward how the concept of a non-classical function should be understood, nor how the related concepts of image, pre-image etc. of such a function mean. However, for the purposes of this paper, it will suffice to look only at classical functions.
Definition 6.3. If A is a classical set, a classical function from A to B is a relation
![]() $R \subseteq A \times B$
which is classical, satisfies
$R \subseteq A \times B$
which is classical, satisfies
![]() $\mathrm {dom}(R) = A$
, and
$\mathrm {dom}(R) = A$
, and
Classical functions take sets as inputs and generate other sets as outputs in a unique way, just as ordinary functions do. Note, however, that even if f is classical, the inputs and outputs need not be classical, so it is dangerous and potentially misleading to use notation like “
![]() $f(x) = y$
”. For example, suppose x and y are such that
$f(x) = y$
”. For example, suppose x and y are such that
![]() $(x,y) \in f$
and
$(x,y) \in f$
and
![]() $y\neq y$
. If we write “
$y\neq y$
. If we write “
![]() $f(x) = y$
” we should also write “
$f(x) = y$
” we should also write “
![]() $f(x) \neq y$
”. But this should not be confused with “
$f(x) \neq y$
”. But this should not be confused with “
![]() $(x,y) \notin f$
”.
$(x,y) \notin f$
”.
Recall that we already introduced the concept of an operation (Definition 4.3). If
![]() $\varphi (x,y)$
is an operation and A a classical set, then
$\varphi (x,y)$
is an operation and A a classical set, then
![]() $\{(x,y) : x \in A \land \varphi (x,y)\}$
is a classical function.Footnote
10
Conversely, if f is a classical function with (classical) domain A, and we fix an arbitrary
$\{(x,y) : x \in A \land \varphi (x,y)\}$
is a classical function.Footnote
10
Conversely, if f is a classical function with (classical) domain A, and we fix an arbitrary
![]() $y_0$
, then the following formula is an operation which coincides with f on
$y_0$
, then the following formula is an operation which coincides with f on
![]() $A = \mathrm {dom}(f)$
:
$A = \mathrm {dom}(f)$
:
When we talk about isomorphisms in the next sections, we will be referring to classical functions when the structures are sets, or operations when they are proper classes.
We now turn our attention to the notions of ordinal, induction on ordinals, and recursive definitions. Informally, ordinals will be classical transitive sets, totally ordered by
![]() $\in $
, and containing only classical sets as members:
$\in $
, and containing only classical sets as members:
Definition 6.4.
![]() $\alpha $
is an ordinal iff:
$\alpha $
is an ordinal iff:
-
•
 $\alpha $
is classical.
$\alpha $
is classical. -
•
 $\forall \beta \: ( \beta \in \alpha \; \to \; \beta $
is classical
$\forall \beta \: ( \beta \in \alpha \; \to \; \beta $
is classical
 $)$
.
$)$
. -
•
 $\forall \beta \: (\beta \in \alpha \; \to \; \beta \subseteq \alpha )$
.
$\forall \beta \: (\beta \in \alpha \; \to \; \beta \subseteq \alpha )$
. -
•
 $\forall \beta \:\forall \gamma \: ((\beta \in \alpha \land \gamma \in \alpha ) \; \to \; (\beta \in \gamma \lor \gamma \in \beta \lor \beta = \gamma ))$
.
$\forall \beta \:\forall \gamma \: ((\beta \in \alpha \land \gamma \in \alpha ) \; \to \; (\beta \in \gamma \lor \gamma \in \beta \lor \beta = \gamma ))$
.
All facts about ordinals known from
![]() ${\mathrm {ZFC}}$
are also true for this definition, because any formula
${\mathrm {ZFC}}$
are also true for this definition, because any formula
![]() $\varphi $
that refers only to ordinals and their members, will be a classical formula. For example, one can show that elements of ordinals are themselves ordinals, that ordinals are unique up to isomorphism, that any well-founded structure is isomorphic to an ordinal, and so on. The same holds for the transfinite induction principle on ordinals, stating that for any formula
$\varphi $
that refers only to ordinals and their members, will be a classical formula. For example, one can show that elements of ordinals are themselves ordinals, that ordinals are unique up to isomorphism, that any well-founded structure is isomorphic to an ordinal, and so on. The same holds for the transfinite induction principle on ordinals, stating that for any formula
![]() $\varphi $
:
$\varphi $
:
From this, the recursion principle follows by usual methods again.
Lemma 6.5 (Recursion principle)
Let G be any operation. Then there exists a unique operation F, such that
![]() $F(x,y)$
implies that x is an ordinal, and such that for every
$F(x,y)$
implies that x is an ordinal, and such that for every
![]() $\alpha $
we have
$\alpha $
we have
We end this section by providing the promised proof that a careless translation of singletons, pairs and power sets would result in proper classes.
Lemma 6.6 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
For sets
![]() $u, u_1, \dots u_n$
, the collections
$u, u_1, \dots u_n$
, the collections
![]() $\{y : y \subseteq u\}$
,
$\{y : y \subseteq u\}$
,
![]() $\{y : y = u\}$
and
$\{y : y = u\}$
and
![]() $\{y : y=u_1 \lor \dots \lor y=u_n\}$
are proper classes.
$\{y : y=u_1 \lor \dots \lor y=u_n\}$
are proper classes.
Proof. We will only prove the first case since the others are similar. We know that
![]() $\{x : \lnot (x \in x)\}$
is a proper class, and from this it easily follows that the collection
$\{x : \lnot (x \in x)\}$
is a proper class, and from this it easily follows that the collection
![]() ${\mathrm {C}}$
of all classical sets forms a proper class.
${\mathrm {C}}$
of all classical sets forms a proper class.
Suppose there is a set
![]() $X = \{y : y \subseteq u\}$
. By Lemma 4.1 we know that
$X = \{y : y \subseteq u\}$
. By Lemma 4.1 we know that
![]() $X^?$
is also a set. By Lemma 4.2(3), we know that
$X^?$
is also a set. By Lemma 4.2(3), we know that
![]() $y \in X^? \Leftrightarrow y^! \subseteq u^?$
. Consider the operation
$y \in X^? \Leftrightarrow y^! \subseteq u^?$
. Consider the operation
Formally, this operation is given by
![]() $\varphi (y,w) \; \equiv \; !(w=y^?)$
which is a classical formula and satisfies the conditions for the Replacement axiom. Moreover, by
$\varphi (y,w) \; \equiv \; !(w=y^?)$
which is a classical formula and satisfies the conditions for the Replacement axiom. Moreover, by
![]() $\mathrm {ACLA}$
the operation is surjective, i.e., for every classical w there is
$\mathrm {ACLA}$
the operation is surjective, i.e., for every classical w there is
![]() $y \in X^?$
such that
$y \in X^?$
such that
![]() $\varphi (y,w)$
holds (take y with
$\varphi (y,w)$
holds (take y with
![]() $y^! = u^?$
and
$y^! = u^?$
and
![]() $y^? = w$
). But then
$y^? = w$
). But then
![]() $F[X^?] = {\mathrm {C}}$
, and since
$F[X^?] = {\mathrm {C}}$
, and since
![]() $X^?$
is a set,
$X^?$
is a set,
![]() ${\mathrm {C}}$
should be a set, which is a contradiction.
${\mathrm {C}}$
should be a set, which is a contradiction.
The above argument is somewhat informal, so readers may wonder what it means to says that something is a proper class, or what a proof by contradiction means in the paraconsistent setting. Formally, what we have proven is that if a set X as above exists, then
![]() $\bot $
.
$\bot $
.
7. A model of
 ${\mathrm {BZFC}}$
${\mathrm {BZFC}}$
In this section we construct a T/F-model for
![]() ${\mathrm {BZFC}}$
starting from
${\mathrm {BZFC}}$
starting from
![]() ${\mathrm {ZFC}}$
. Usually this would yield a relative consistency proof, i.e., a proof that if
${\mathrm {ZFC}}$
. Usually this would yield a relative consistency proof, i.e., a proof that if
![]() ${\mathrm {ZFC}}$
is consistent then
${\mathrm {ZFC}}$
is consistent then
![]() ${\mathrm {BZFC}}$
is consistent. Of course
${\mathrm {BZFC}}$
is consistent. Of course
![]() ${\mathrm {BZFC}}$
is, by design, inconsistent, so instead we will talk of non-triviality.
${\mathrm {BZFC}}$
is, by design, inconsistent, so instead we will talk of non-triviality.
Definition 7.1. A
![]() $\mathrm {BS4}$
-theory
$\mathrm {BS4}$
-theory
![]() $\Gamma $
is called non-trivial if
$\Gamma $
is called non-trivial if
![]() $\Gamma \not \vdash _{\mathrm {BS4}} \bot $
.
$\Gamma \not \vdash _{\mathrm {BS4}} \bot $
.
Definition 7.2 (
 ${\mathrm {ZFC}}$
)
${\mathrm {ZFC}}$
)
By induction on ordinals we define:
-
•
 $W_0 = \varnothing ,$
$W_0 = \varnothing ,$
-
•
 $W_{\alpha +1} := \mathscr {P}(W_\alpha ) \times \mathscr {P}(W_\alpha ),$
$W_{\alpha +1} := \mathscr {P}(W_\alpha ) \times \mathscr {P}(W_\alpha ),$
-
•
 $W_{\lambda } = \bigcup _{\alpha <\lambda }W_\alpha $
for limit
$W_{\lambda } = \bigcup _{\alpha <\lambda }W_\alpha $
for limit
 $\lambda ,$
$\lambda ,$
-
•
 $\displaystyle {\mathbb {W}} := \bigcup _{\alpha \in \text {Ord}}W_\alpha. $
$\displaystyle {\mathbb {W}} := \bigcup _{\alpha \in \text {Ord}}W_\alpha. $
The positive and negative interpretations of
![]() $\in $
and
$\in $
and
![]() $=$
are given by:
$=$
are given by:
-
•
 $(a,b) \in ^+ (c,d)$
iff
$(a,b) \in ^+ (c,d)$
iff
 $(a,b) \in c,$
$(a,b) \in c,$
-
•
 $(a,b) \in ^- (c,d)$
iff
$(a,b) \in ^- (c,d)$
iff
 $(a,b) \notin d,$
$(a,b) \notin d,$
-
•
 $(a,b) =^+ (c,d)$
iff
$(a,b) =^+ (c,d)$
iff
 $(a,b) = (c,d),$
$(a,b) = (c,d),$
-
•
 $(a,b) =^- (c,d)$
iff
$(a,b) =^- (c,d)$
iff
 $\exists z \in a \setminus d$
or
$\exists z \in a \setminus d$
or
 $\exists z \in c \setminus b$
.
$\exists z \in c \setminus b$
.
The following properties of
![]() ${\mathbb {W}}$
are easily verifiable.
${\mathbb {W}}$
are easily verifiable.
Lemma 7.3 (
 ${\mathrm {ZFC}}$
)
${\mathrm {ZFC}}$
)
-
1. If
 $\alpha <\beta $
then
$\alpha <\beta $
then
 $W_\alpha \subseteq W_\beta $
.
$W_\alpha \subseteq W_\beta $
. -
2. If
 $(a,b) \in W_\alpha $
,
$(a,b) \in W_\alpha $
,
 $a' \subseteq a,$
and
$a' \subseteq a,$
and
 $b' \subseteq b$
then
$b' \subseteq b$
then
 $(a',b') \in W_\alpha $
.
$(a',b') \in W_\alpha $
. -
3.
 $x \in {\mathbb {W}}$
iff
$x \in {\mathbb {W}}$
iff
 $x = (a,b)$
for some
$x = (a,b)$
for some
 $a,b \subseteq {\mathbb {W}}$
.
$a,b \subseteq {\mathbb {W}}$
.
Proof. Induction on the definition.
Now
![]() $({\mathbb {W}},\in ^+, \in ^-, =^+,=^-)$
may be considered a T/F-model in the language of set theory, except that
$({\mathbb {W}},\in ^+, \in ^-, =^+,=^-)$
may be considered a T/F-model in the language of set theory, except that
![]() ${\mathbb {W}}$
is a proper class. Therefore, “
${\mathbb {W}}$
is a proper class. Therefore, “
![]() ${\mathbb {W}} \models \varphi $
” must be understood via relativization: for every
${\mathbb {W}} \models \varphi $
” must be understood via relativization: for every
![]() $\varphi $
we define
$\varphi $
we define
![]() $\varphi ^{{\mathbb {W}}, T} $
and
$\varphi ^{{\mathbb {W}}, T} $
and
![]() $\varphi ^{{\mathbb {W}},F}$
by syntactic induction, generalizing from Definition 2.3 in the obvious way (we leave the details to the reader). For a theory
$\varphi ^{{\mathbb {W}},F}$
by syntactic induction, generalizing from Definition 2.3 in the obvious way (we leave the details to the reader). For a theory
![]() $\Gamma $
,
$\Gamma $
,
![]() ${\mathbb {W}} \models \Gamma $
means that for every
${\mathbb {W}} \models \Gamma $
means that for every
![]() $\varphi $
in
$\varphi $
in
![]() $\Gamma $
, there is a proof of
$\Gamma $
, there is a proof of
![]() $\varphi ^{{\mathbb {W}},T}$
.
$\varphi ^{{\mathbb {W}},T}$
.
Theorem 7.4 (
 ${\mathrm {ZFC}}$
)
${\mathrm {ZFC}}$
)
![]() ${\mathbb {W}} \models {\mathrm {BZFC}}$
.
${\mathbb {W}} \models {\mathrm {BZFC}}$
.
Proof. We will prove Extensionality and Comprehension in some detail and leave the rest to the reader.
Written out in full, the relativization (Extensionality)
![]() $^{{\mathbb {W}},T}$
reads as follows:
$^{{\mathbb {W}},T}$
reads as follows:
Assume
![]() $(a,b)$
and
$(a,b)$
and
![]() $(c,d)$
are arbitrary, and we show that the two equivalences hold. For the first we have
$(c,d)$
are arbitrary, and we show that the two equivalences hold. For the first we have
![]() $ \forall z\in {\mathbb {W}} ((z \in ^+ (a,b) \leftrightarrow z \in ^+ (c,d)) \land (z \in ^- (a,b) \leftrightarrow z \in ^- (c,d))$
$ \forall z\in {\mathbb {W}} ((z \in ^+ (a,b) \leftrightarrow z \in ^+ (c,d)) \land (z \in ^- (a,b) \leftrightarrow z \in ^- (c,d))$
![]() $\stackrel {(*)}{\leftrightarrow }\;\;$
$\stackrel {(*)}{\leftrightarrow }\;\;$
![]() $\forall z\in {\mathbb {W}} ((z \in a \leftrightarrow z \in c) \land (z \notin b \leftrightarrow z \notin d))$
$\forall z\in {\mathbb {W}} ((z \in a \leftrightarrow z \in c) \land (z \notin b \leftrightarrow z \notin d))$
![]() $\stackrel {(**)}{\leftrightarrow }\;\;$
$\stackrel {(**)}{\leftrightarrow }\;\;$
![]() $\forall z ((z \in a \leftrightarrow z \in c) \land (z \notin b \leftrightarrow z \notin d))$
$\forall z ((z \in a \leftrightarrow z \in c) \land (z \notin b \leftrightarrow z \notin d))$
![]() $\leftrightarrow \;\; \ a=c$
and
$\leftrightarrow \;\; \ a=c$
and
![]() $b=d$
$b=d$
![]() $\leftrightarrow \;\;\ (a,b) =^+ (c,d),$
$\leftrightarrow \;\;\ (a,b) =^+ (c,d),$
where
![]() $(*)$
refers to the definition of
$(*)$
refers to the definition of
![]() $\in ^+$
and
$\in ^+$
and
![]() $\in ^-$
, and
$\in ^-$
, and
![]() $(**)$
is because
$(**)$
is because
![]() ${\mathbb {W}}$
is “transitive” in the sense that
${\mathbb {W}}$
is “transitive” in the sense that
![]() $a,b,c,d \in {\mathbb {W}}$
only contain sets which are also in
$a,b,c,d \in {\mathbb {W}}$
only contain sets which are also in
![]() ${\mathbb {W}}$
.
${\mathbb {W}}$
.
For the second equivalence we have
![]() $\exists z \in {\mathbb {W}}((z \in ^+ (a,b) \land z \in ^- (c,d)) \lor (z \in ^+ (c,d) \land z \in ^- (a,b))$
$\exists z \in {\mathbb {W}}((z \in ^+ (a,b) \land z \in ^- (c,d)) \lor (z \in ^+ (c,d) \land z \in ^- (a,b))$
![]() $\leftrightarrow \;\;\ \exists z(( z \in a \land z \notin d) \lor (z \in c \land z \notin b))$
$\leftrightarrow \;\;\ \exists z(( z \in a \land z \notin d) \lor (z \in c \land z \notin b))$
![]() $\leftrightarrow \;\;\ (a,b) =^- (c,d).$
$\leftrightarrow \;\;\ (a,b) =^- (c,d).$
Next we look at (Comprehension)
![]() $^{{\mathbb {W}},T}$
which is the following statement:Footnote
12
$^{{\mathbb {W}},T}$
which is the following statement:Footnote
12
Suppose
![]() $(a,b)\in {\mathbb {W}}$
and
$(a,b)\in {\mathbb {W}}$
and
![]() $\varphi $
is given. Define
$\varphi $
is given. Define
Footnote
13
Then
![]() $(c,d) \in {\mathbb {W}}$
by construction and for all z we have
$(c,d) \in {\mathbb {W}}$
by construction and for all z we have
![]() $z \in c \leftrightarrow (z \in a \land \varphi (z)^{{\mathbb {W}},T})$
and
$z \in c \leftrightarrow (z \in a \land \varphi (z)^{{\mathbb {W}},T})$
and
![]() $z \notin d \leftrightarrow (z \notin b \lor \varphi (z)^{{\mathbb {W}},F})$
. This is exactly the statement above as needs to be proved.
$z \notin d \leftrightarrow (z \notin b \lor \varphi (z)^{{\mathbb {W}},F})$
. This is exactly the statement above as needs to be proved.
Corollary 7.5. If
![]() ${\mathrm {ZFC}}$
is consistent then
${\mathrm {ZFC}}$
is consistent then
![]() ${\mathrm {BZFC}}$
is non-trivial.
${\mathrm {BZFC}}$
is non-trivial.
We have thus shown that
![]() ${\mathrm {BZFC}}$
is essentially not a more problematic theory than
${\mathrm {BZFC}}$
is essentially not a more problematic theory than
![]() ${\mathrm {ZFC}}$
. In addition, the canonical model
${\mathrm {ZFC}}$
. In addition, the canonical model
![]() ${\mathbb {W}}$
contains a natural copy of the classical universe V.
${\mathbb {W}}$
contains a natural copy of the classical universe V.
Definition 7.6 (
 ${\mathrm {ZFC}}$
)
${\mathrm {ZFC}}$
)
For every
![]() $x \in V$
, inductively define
$x \in V$
, inductively define
Also let
![]() $\check {V} := \{\check {x} : x \in V\}$
.
$\check {V} := \{\check {x} : x \in V\}$
.
Lemma 7.7 (
 ${\mathrm {ZFC}}$
)
${\mathrm {ZFC}}$
)
The mapping
is an isomorphism between
![]() $(V,\in , \notin ,=,\neq )$
and
$(V,\in , \notin ,=,\neq )$
and
![]() $(\check {V},\in ^+,\in ^-,=^+,=^-)$
.
$(\check {V},\in ^+,\in ^-,=^+,=^-)$
.
Proof. Easy consequence of the definitions.
8. Hereditarily classical sets
Starting in
![]() ${\mathrm {BZFC}}$
, we can also construct a natural model of
${\mathrm {BZFC}}$
, we can also construct a natural model of
![]() ${\mathrm {ZFC}}$
: this is the class of “hereditarily classical” sets.
${\mathrm {ZFC}}$
: this is the class of “hereditarily classical” sets.
Definition 8.1 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
By induction on ordinalsFootnote 14 define:
-
•
 ${\text {HCL}}_0 = \varnothing ,$
${\text {HCL}}_0 = \varnothing ,$
-
•
 ${\text {HCL}}_{\alpha +1} := \{X \subseteq {\text {HCL}}_\alpha : X$
is classical
${\text {HCL}}_{\alpha +1} := \{X \subseteq {\text {HCL}}_\alpha : X$
is classical
 $\},$
Footnote
15
$\},$
Footnote
15
-
•
 ${\text {HCL}}_{\lambda } = \bigcup _{\alpha <\lambda }{\text {HCL}}_\alpha $
for limit
${\text {HCL}}_{\lambda } = \bigcup _{\alpha <\lambda }{\text {HCL}}_\alpha $
for limit
 $\lambda ,$
$\lambda ,$
-
•
 $\displaystyle {\mathbb {HCL}} := \bigcup _{\alpha \in \text {Ord}}{\text {HCL}}_\alpha. $
$\displaystyle {\mathbb {HCL}} := \bigcup _{\alpha \in \text {Ord}}{\text {HCL}}_\alpha. $
![]() ${\mathbb {HCL}}$
is a transitive proper class, and for all x, we have that
${\mathbb {HCL}}$
is a transitive proper class, and for all x, we have that
![]() $x \in {\mathbb {HCL}}$
if and only if x is classical and
$x \in {\mathbb {HCL}}$
if and only if x is classical and
![]() $x \subseteq {\mathbb {HCL}}$
. Again, the notation
$x \subseteq {\mathbb {HCL}}$
. Again, the notation
![]() ${\mathbb {HCL}} \models \varphi $
and
${\mathbb {HCL}} \models \varphi $
and
![]() ${\mathbb {HCL}} \models \Gamma $
refers to relativization where quantification is restricted to range over
${\mathbb {HCL}} \models \Gamma $
refers to relativization where quantification is restricted to range over
![]() ${\mathbb {HCL}}$
.
${\mathbb {HCL}}$
.
Theorem 8.2 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
![]() ${\mathbb {HCL}} \models {\mathrm {ZFC}}$
.
${\mathbb {HCL}} \models {\mathrm {ZFC}}$
.
Proof. It is clear that
![]() ${\mathbb {HCL}} \models $
(every set is classical), so by the discussion in Section 5 it suffices to show that
${\mathbb {HCL}} \models $
(every set is classical), so by the discussion in Section 5 it suffices to show that
![]() ${\mathbb {HCL}} \models \mathrm {PZFC}$
. Most of the axioms are straightforward since none of them postulate the existence of non-classical sets without assuming the existence of non-classical sets.
${\mathbb {HCL}} \models \mathrm {PZFC}$
. Most of the axioms are straightforward since none of them postulate the existence of non-classical sets without assuming the existence of non-classical sets.
We will only show (Comprehension)
![]() $^{\mathbb {HCL}}$
. Suppose u is a hereditarily classical set and
$^{\mathbb {HCL}}$
. Suppose u is a hereditarily classical set and
![]() $\varphi $
any formula. It is enough to show that
$\varphi $
any formula. It is enough to show that
![]() $x := \{y \in u : \varphi ^{{\mathbb {HCL}}}(y,a_1, \dots , a_n)\}$
is a hereditarily classical set if the parameters
$x := \{y \in u : \varphi ^{{\mathbb {HCL}}}(y,a_1, \dots , a_n)\}$
is a hereditarily classical set if the parameters
![]() $a_1,\dots , a_n$
are hereditarily classical.Footnote
16
Since
$a_1,\dots , a_n$
are hereditarily classical.Footnote
16
Since
![]() $x \subseteq u$
and
$x \subseteq u$
and
![]() $u \subseteq {\mathbb {HCL}}$
we know that
$u \subseteq {\mathbb {HCL}}$
we know that
![]() $x \subseteq {\mathbb {HCL}}$
, so it remains to show that x itself is classical. But from the fact that
$x \subseteq {\mathbb {HCL}}$
, so it remains to show that x itself is classical. But from the fact that
![]() $y, a_1, \dots a_n$
are classical sets, it follows by an easy induction that
$y, a_1, \dots a_n$
are classical sets, it follows by an easy induction that
![]() $\varphi $
is a classical formula, i.e.,
$\varphi $
is a classical formula, i.e.,
![]() $!\varphi \leftrightarrow ? \varphi $
. It follows that
$!\varphi \leftrightarrow ? \varphi $
. It follows that
![]() $x^!=x^?$
, so x is classical.
$x^!=x^?$
, so x is classical.
Corollary 8.3. If
![]() ${\mathrm {BZFC}}$
is non-trivial, then
${\mathrm {BZFC}}$
is non-trivial, then
![]() ${\mathrm {ZFC}}$
is consistent.
${\mathrm {ZFC}}$
is consistent.
In analogy to Definition 7.6, we can now consider an embedding from the universe of
![]() ${\mathrm {BZFC}}$
(which we also refer to as V, hopefully not leading to confusion) to a natural copy of it within
${\mathrm {BZFC}}$
(which we also refer to as V, hopefully not leading to confusion) to a natural copy of it within
![]() ${\mathbb {HCL}}$
.
${\mathbb {HCL}}$
.
Definition 8.4 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
For every set
![]() $x$
, inductively define:
$x$
, inductively define:
Also let
![]() $\hat {V} := \{\hat {x} : x \in V\}$
. For
$\hat {V} := \{\hat {x} : x \in V\}$
. For
![]() $\hat {x},\hat {y} \in \hat {V}$
define:
$\hat {x},\hat {y} \in \hat {V}$
define:
-
•
 $ \hat {x} E^+ \hat {y}\ \leftrightarrow \ x\in y,$
$ \hat {x} E^+ \hat {y}\ \leftrightarrow \ x\in y,$
-
•
 $ \hat {x} E^- \hat {y}\ \leftrightarrow \ x\notin y,$
$ \hat {x} E^- \hat {y}\ \leftrightarrow \ x\notin y,$
-
•
 $ \hat {x} \approx ^+ \hat {y}\ \leftrightarrow \ x= y$
$ \hat {x} \approx ^+ \hat {y}\ \leftrightarrow \ x= y$
-
•
 $ \hat {x} \approx ^- \hat {y}\ \leftrightarrow \ x\neq y.$
$ \hat {x} \approx ^- \hat {y}\ \leftrightarrow \ x\neq y.$
Now
![]() $\hat {x}$
are hereditarily classical sets,
$\hat {x}$
are hereditarily classical sets,
![]() $\hat {V}$
is a proper class of hereditarily classical sets, and
$\hat {V}$
is a proper class of hereditarily classical sets, and
![]() $E^+, E^-,\approx ^+, \approx ^-$
are hereditarily classical, class-sized binary relations on
$E^+, E^-,\approx ^+, \approx ^-$
are hereditarily classical, class-sized binary relations on
![]() $\hat {V}$
.
$\hat {V}$
.
Lemma 8.5 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
The mapping
is an isomorphism between
![]() $(V,\in , \notin , =,\neq )$
and
$(V,\in , \notin , =,\neq )$
and
![]() $(\hat {V},E^+,E^-,\approx ^+,\approx ^-)$
.
$(\hat {V},E^+,E^-,\approx ^+,\approx ^-)$
.
Proof. Follows immediately from the definitions.
9. Bi-interpretability
We already know that
![]() ${\mathrm {ZFC}}$
in
${\mathrm {ZFC}}$
in
![]() ${\mathrm {BZFC}}$
are mutually interpretable, i.e., each theory can construct a natural model for the other. In fact we prove more than that:
${\mathrm {BZFC}}$
are mutually interpretable, i.e., each theory can construct a natural model for the other. In fact we prove more than that:
Theorem 9.1.
![]() ${\mathrm {BZFC}}$
and
${\mathrm {BZFC}}$
and
![]() ${\mathrm {ZFC}}$
are bi-interpretable.
${\mathrm {ZFC}}$
are bi-interpretable.
Here the term bi-interpretability may either be understood semantically, saying that the model constructions interpreting the other theory are reversible up to isomorphism, or syntactically, saying that a sentence is a consequence of one theory if and only if its translation (relativisation) is a consequence of the other, and vice versa, in the corresponding logics. Theorems 9.2 and 9.4 together with results from the previous sections concern the semantic understanding while Corollaries 9.3 and 9.5 refer to the syntactic one.
In Lemma 7.7 we defined an isomorphism
![]() $i: x \mapsto \check {x}$
from V and
$i: x \mapsto \check {x}$
from V and
![]() $\check {V}$
, so it remains only to show that
$\check {V}$
, so it remains only to show that
![]() $\check {V}$
is the same thing as what
$\check {V}$
is the same thing as what
![]() ${\mathbb {W}}$
believes
${\mathbb {W}}$
believes
![]() ${\mathbb {HCL}}$
to be (provably in
${\mathbb {HCL}}$
to be (provably in
![]() ${\mathrm {ZFC}}$
).
${\mathrm {ZFC}}$
).
Lemma 9.2 (
 ${\mathrm {ZFC}}$
)
${\mathrm {ZFC}}$
)
![]() ${\mathbb {HCL}}^{\mathbb {W}} = \check {V}$
.
${\mathbb {HCL}}^{\mathbb {W}} = \check {V}$
.
Proof. To show
![]() $\supseteq $
take
$\supseteq $
take
![]() $\check {x} \in \check {V}$
. By construction
$\check {x} \in \check {V}$
. By construction
![]() $\check {x} = ( \{\check {y} : y \in x\}, \{\check {y} : y \in x\})$
. Inductively, we may assume that each
$\check {x} = ( \{\check {y} : y \in x\}, \{\check {y} : y \in x\})$
. Inductively, we may assume that each
![]() $\check {y}$
appearing above is in
$\check {y}$
appearing above is in
![]() ${\mathbb {HCL}}^{\mathbb {W}}$
, therefore
${\mathbb {HCL}}^{\mathbb {W}}$
, therefore
![]() ${\mathbb {W}} \models \check {x} \subseteq {\mathbb {HCL}}$
. Moreover,
${\mathbb {W}} \models \check {x} \subseteq {\mathbb {HCL}}$
. Moreover,
![]() $\check {x}$
has the same !-extension as ?-extension, therefore
$\check {x}$
has the same !-extension as ?-extension, therefore
![]() ${\mathbb {W}} \models (\check {x}$
is classical). Together, this implies
${\mathbb {W}} \models (\check {x}$
is classical). Together, this implies
![]() ${\mathbb {W}} \models \check {x} \in {\mathbb {HCL}}$
.
${\mathbb {W}} \models \check {x} \in {\mathbb {HCL}}$
.
To show
![]() $\subseteq $
, suppose
$\subseteq $
, suppose
![]() $x \in {\mathbb {HCL}}^{\mathbb {W}}$
, i.e.,
$x \in {\mathbb {HCL}}^{\mathbb {W}}$
, i.e.,
![]() $x \in {\mathbb {W}}$
and
$x \in {\mathbb {W}}$
and
![]() ${\mathbb {W}} \models (x$
is hereditarily classical). Let
${\mathbb {W}} \models (x$
is hereditarily classical). Let
![]() $a,b \in {\mathbb {W}}$
be such that
$a,b \in {\mathbb {W}}$
be such that
![]() $x=(a,b)$
. Since
$x=(a,b)$
. Since
![]() ${\mathbb {W}} \models x$
is classical, in particular
${\mathbb {W}} \models x$
is classical, in particular
![]() ${\mathbb {W}} \models x^! = x^?$
, therefore
${\mathbb {W}} \models x^! = x^?$
, therefore
![]() $a=b$
. Moreover,
$a=b$
. Moreover,
![]() ${\mathbb {W}} \models x \subseteq {\mathbb {HCL}}$
which implies that
${\mathbb {W}} \models x \subseteq {\mathbb {HCL}}$
which implies that
![]() $a \subseteq {\mathbb {HCL}}^{\mathbb {W}}$
. Inductively, we may assume that every element in a is of the form
$a \subseteq {\mathbb {HCL}}^{\mathbb {W}}$
. Inductively, we may assume that every element in a is of the form
![]() $\check {z}$
for some
$\check {z}$
for some
![]() $z \in V$
. Let
$z \in V$
. Let
![]() $\tilde {a} := \{z : \check {z} \in a\}$
. Then
$\tilde {a} := \{z : \check {z} \in a\}$
. Then
![]() $x= ( \{\check {z} : z \in \tilde {a}\}, \{\check {z} : z \in \tilde {a}\}) = (a,a)$
by construction. But then, by definition,
$x= ( \{\check {z} : z \in \tilde {a}\}, \{\check {z} : z \in \tilde {a}\}) = (a,a)$
by construction. But then, by definition,
![]() $x = ({\tilde {a}}\check {)} $
.
$x = ({\tilde {a}}\check {)} $
.
Corollary 9.3.
![]() ${\mathrm {ZFC}} \vdash \varphi \;\;$
iff
${\mathrm {ZFC}} \vdash \varphi \;\;$
iff
![]() $\;\; {\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \varphi \right )$
.
$\;\; {\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \varphi \right )$
.
Proof. If
![]() ${\mathrm {ZFC}} \vdash \varphi $
then clearly
${\mathrm {ZFC}} \vdash \varphi $
then clearly
![]() ${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \varphi \right )$
. Suppose
${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \varphi \right )$
. Suppose
![]() ${\mathrm {BZFC}}\vdash_{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \varphi \right )$
. Then
${\mathrm {BZFC}}\vdash_{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \varphi \right )$
. Then
![]() ${\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \left ( {\mathbb {HCL}} \models \varphi \right ) \right )$
, i.e.,
${\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \left ( {\mathbb {HCL}} \models \varphi \right ) \right )$
, i.e.,
![]() ${\mathrm {ZFC}} \vdash ({\mathbb {HCL}}^{\mathbb {W}} \models \varphi )$
. But by the above we have
${\mathrm {ZFC}} \vdash ({\mathbb {HCL}}^{\mathbb {W}} \models \varphi )$
. But by the above we have
![]() ${\mathbb {HCL}}^{\mathbb {W}} = \check {V} \cong V$
, so
${\mathbb {HCL}}^{\mathbb {W}} = \check {V} \cong V$
, so
![]() ${\mathrm {ZFC}} \vdash \varphi $
.
${\mathrm {ZFC}} \vdash \varphi $
.
For the reverse direction, if we start in
![]() ${\mathrm {BZFC}}$
we already have the
${\mathrm {BZFC}}$
we already have the
![]() $j: x \to \hat {x}$
from Definition 8.4, so it remains to show that
$j: x \to \hat {x}$
from Definition 8.4, so it remains to show that
![]() $\hat {V}$
is the same as what
$\hat {V}$
is the same as what
![]() ${\mathbb {HCL}}$
believes
${\mathbb {HCL}}$
believes
![]() ${\mathbb {W}}$
to be, provably in
${\mathbb {W}}$
to be, provably in
![]() ${\mathrm {BZFC}}$
.
${\mathrm {BZFC}}$
.
Lemma 9.4 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
![]() ${\mathbb {W}}^{\mathbb {HCL}} = \hat {V}$
.
${\mathbb {W}}^{\mathbb {HCL}} = \hat {V}$
.
Proof. Suppose
![]() $\hat {x} \in \hat {V}$
. Then
$\hat {x} \in \hat {V}$
. Then
![]() $\hat {x} = ( \{\hat {y} : y \in x^!\}, \{\hat {y} : y \in x^?\})$
, and since every
$\hat {x} = ( \{\hat {y} : y \in x^!\}, \{\hat {y} : y \in x^?\})$
, and since every
![]() $\hat {y}$
appearing here is in
$\hat {y}$
appearing here is in
![]() $ \hat {V}$
, inductively we can assume that it is also in
$ \hat {V}$
, inductively we can assume that it is also in
![]() ${\mathbb {W}}^{\mathbb {HCL}}$
. By definition
${\mathbb {W}}^{\mathbb {HCL}}$
. By definition
![]() $\hat {x}$
is hereditarily classical and we have
$\hat {x}$
is hereditarily classical and we have
![]() ${\mathbb {HCL}} \models (\hat {x} = (a,b)$
and
${\mathbb {HCL}} \models (\hat {x} = (a,b)$
and
![]() $a,b \subseteq {\mathbb {W}})$
. By Lemma 7.3(3) applied in
$a,b \subseteq {\mathbb {W}})$
. By Lemma 7.3(3) applied in
![]() ${\mathbb {HCL}}$
, this means
${\mathbb {HCL}}$
, this means
![]() ${\mathbb {HCL}} \models \hat {x} \in {\mathbb {W}}$
.
${\mathbb {HCL}} \models \hat {x} \in {\mathbb {W}}$
.
Conversely, suppose
![]() $w \in {\mathbb {W}}^{\mathbb {HCL}}$
. Again by Lemma 7.3(3) we know that
$w \in {\mathbb {W}}^{\mathbb {HCL}}$
. Again by Lemma 7.3(3) we know that
![]() ${\mathbb {HCL}} \models (w = (u,v)$
and
${\mathbb {HCL}} \models (w = (u,v)$
and
![]() $u,v \subseteq {\mathbb {W}})$
. Then
$u,v \subseteq {\mathbb {W}})$
. Then
![]() $u,v \subseteq {\mathbb {W}}^{\mathbb {HCL}}$
so, inductively, we have
$u,v \subseteq {\mathbb {W}}^{\mathbb {HCL}}$
so, inductively, we have
![]() $u,v \subseteq \hat {V}$
. Therefore, let
$u,v \subseteq \hat {V}$
. Therefore, let
![]() $\tilde {u} = \{y : \hat {y} \in u\}$
and
$\tilde {u} = \{y : \hat {y} \in u\}$
and
![]() $\tilde {v} = \{y : \hat {y} \in v\}$
. Then
$\tilde {v} = \{y : \hat {y} \in v\}$
. Then
![]() $\tilde {u}$
and
$\tilde {u}$
and
![]() $\tilde {v}$
are classical sets (because
$\tilde {v}$
are classical sets (because
![]() $u,v$
are), so by
$u,v$
are), so by
![]() $\mathrm {ACLA}$
there exists a set x such that
$\mathrm {ACLA}$
there exists a set x such that
![]() $x^! = \tilde {u}$
and
$x^! = \tilde {u}$
and
![]() $x^? = \tilde {v}$
. Then we have
$x^? = \tilde {v}$
. Then we have
This shows that
![]() $w \in \hat {V}$
.
$w \in \hat {V}$
.
Corollary 9.5.
![]() ${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \varphi \;\;$
iff
${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \varphi \;\;$
iff
![]() $\;\; {\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \varphi \right )$
.
$\;\; {\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \varphi \right )$
.
Proof. If
![]() ${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \varphi $
then clearly
${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \varphi $
then clearly
![]() ${\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \varphi \right )$
. Suppose
${\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \varphi \right )$
. Suppose
![]() ${\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \varphi \right )$
. Then
${\mathrm {ZFC}} \vdash \left ( {\mathbb {W}} \models \varphi \right )$
. Then
![]() ${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \left ( {\mathbb {W}} \models \varphi \right )\right )$
, so
${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {HCL}} \models \left ( {\mathbb {W}} \models \varphi \right )\right )$
, so
![]() ${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {W}}^{\mathbb {HCL}} \models \varphi \right )$
. But in
${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \left ( {\mathbb {W}}^{\mathbb {HCL}} \models \varphi \right )$
. But in
![]() ${\mathrm {BZFC}}$
we have that
${\mathrm {BZFC}}$
we have that
![]() ${\mathbb {W}}^{\mathbb {HCL}} = \hat {V} \cong V$
, giving us
${\mathbb {W}}^{\mathbb {HCL}} = \hat {V} \cong V$
, giving us
![]() ${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \varphi $
.
${\mathrm {BZFC}} \vdash _{\mathrm {BS4}} \varphi $
.
10. Tarski semantics in
 $\mathrm {PZFC}$
$\mathrm {PZFC}$
A discussion that sometimes arises in the philosophy of logic is the extent to which the meta-theory affects the formal theory under consideration. For example, the recursive definition of the negation and disjunction in Tarski semantics means that
Thus excluded middle is a tautology in classical logic only because excluded middle is covertly assumed to hold in the meta-theory. Likewise, since we have
a contradiction
![]() $\varphi \land \lnot \varphi $
is classically unsatisfiable only because
$\varphi \land \lnot \varphi $
is classically unsatisfiable only because
![]() $\mathcal {M} \models \varphi $
and
$\mathcal {M} \models \varphi $
and
![]() $\mathcal {M} \not \models \varphi $
cannot both be true in the meta-theory.
$\mathcal {M} \not \models \varphi $
cannot both be true in the meta-theory.
Of course, non-classical logics may be set up by considering alternative (non-Tarski) semantics, so that the formal system in question exhibit non-classicality while the meta-theory remains classical. Such is the case, for example, with Kripke semantics for intuitionistic logic, and the T/F-models for
![]() $\mathrm {BS4}$
from Section 2. But this does not shed any light on the question how the logic generated by Tarski semantics is affected by the logic that holds in the meta-theory.
$\mathrm {BS4}$
from Section 2. But this does not shed any light on the question how the logic generated by Tarski semantics is affected by the logic that holds in the meta-theory.
Questions of this sort have been considered, for example, by Shapiro in [Reference Shapiro27, chaps 6 and 7] or by Bacon in [Reference Bacon5], and some related concerns about logical pluralism are voiced in [Reference Passmann21, Reference Sereni and Fogliani26]. No doubt, early logicians like Gödel and Tarski would have been aware of this issue as well. Nevertheless, although the phenomenon of meta-logic affecting formal logic seems to be a well-known concern in philosophy of language and theories of truth, we do not know of a rigorous mathematical analysis of this phenomenon, certainly not in the context of paraconsistency. Indeed, such an analysis would require a sufficiently developed theory of paraconsistent mathematics to begin with, in which the relevant model-theoretic definitions and proofs might be formalised and carried out. Now that we have
![]() ${\mathrm {BZFC}}$
at our disposal, we are in a position to do exactly that.
${\mathrm {BZFC}}$
at our disposal, we are in a position to do exactly that.
The setup is as follows: in
![]() $\mathrm {PZFC}$
, we can define Tarski semantics by employing the usual inductive definition (although predicates are interpretations non-classically). This gives rise to a semantic consequence relation, which we shall denote by
$\mathrm {PZFC}$
, we can define Tarski semantics by employing the usual inductive definition (although predicates are interpretations non-classically). This gives rise to a semantic consequence relation, which we shall denote by
![]() $\models $
, and one can study the logic which is sound and complete with respect to this relation. It turns out that in
$\models $
, and one can study the logic which is sound and complete with respect to this relation. It turns out that in
![]() ${\mathrm {BZFC}}$
(i.e., in the presence of the Anti-Classicality Axiom), this logic is precisely
${\mathrm {BZFC}}$
(i.e., in the presence of the Anti-Classicality Axiom), this logic is precisely
![]() $\mathrm {BS4}$
(Theorem 10.3). On the other hand, in
$\mathrm {BS4}$
(Theorem 10.3). On the other hand, in
![]() $\mathrm {PZFC} \; + $
“all sets are classical”, it is simply classical logic.
$\mathrm {PZFC} \; + $
“all sets are classical”, it is simply classical logic.
In fact, we can be more specific by looking at only the paraconsistent, or only the paracomplete, fragment of the Anti-Classicality Axiom. In
![]() $\mathrm {PZFC}$
, one can then show that the logic generated by Tarski semantics satisfies the exact same level of non-classicality as we assume to hold in the meta-theory (Theorems 10.10 and 10.11).
$\mathrm {PZFC}$
, one can then show that the logic generated by Tarski semantics satisfies the exact same level of non-classicality as we assume to hold in the meta-theory (Theorems 10.10 and 10.11).
Definition 10.1 (
 $\mathrm {PZFC}$
)
$\mathrm {PZFC}$
)
The syntax of first-order logic is assumed to be coded by classical sets. A Tarski model
![]() $\mathcal {M}$
consists of a classical set M as a domain, and (not necessarily classical) interpretations for all constant and relation symbols. For simplicity we will leave out function symbols. Inductively we define:
$\mathcal {M}$
consists of a classical set M as a domain, and (not necessarily classical) interpretations for all constant and relation symbols. For simplicity we will leave out function symbols. Inductively we define:
-
1.
 $\mathcal {M} \models (t=s)[a,b] \;\;\; \Leftrightarrow \;\;\; a=b$
.
$\mathcal {M} \models (t=s)[a,b] \;\;\; \Leftrightarrow \;\;\; a=b$
. -
2.
 $\mathcal {M} \models R(t_1, \dots , t_n)[a_1, \dots , a_n] \;\;\; \Leftrightarrow \;\;\; R^{\mathcal {M}}(a_1, \dots a_n)$
.
$\mathcal {M} \models R(t_1, \dots , t_n)[a_1, \dots , a_n] \;\;\; \Leftrightarrow \;\;\; R^{\mathcal {M}}(a_1, \dots a_n)$
. -
3.
 $\mathcal {M} \models {\sim } \varphi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \not \models \varphi $
.Footnote
17
$\mathcal {M} \models {\sim } \varphi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \not \models \varphi $
.Footnote
17
-
4.
 $\mathcal {M} \models \varphi \land \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi $
and
$\mathcal {M} \models \varphi \land \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi $
and
 $\mathcal {M} \models \psi $
.
$\mathcal {M} \models \psi $
. -
5.
 $\mathcal {M} \models \varphi \lor \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi $
or
$\mathcal {M} \models \varphi \lor \psi \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi $
or
 $\mathcal {M} \models \psi $
.
$\mathcal {M} \models \psi $
. -
6.
 $\mathcal {M} \models \varphi \to \psi \;\;\; \Leftrightarrow \;\;\;\ ( \mathcal {M} \models \varphi \to \mathcal {M} \models \psi )$
.
$\mathcal {M} \models \varphi \to \psi \;\;\; \Leftrightarrow \;\;\;\ ( \mathcal {M} \models \varphi \to \mathcal {M} \models \psi )$
. -
7.
 $\mathcal {M} \models \varphi \leftrightarrow \psi \;\;\; \Leftrightarrow \;\;\;$
(
$\mathcal {M} \models \varphi \leftrightarrow \psi \;\;\; \Leftrightarrow \;\;\;$
(
 $\mathcal {M} \models \varphi \leftrightarrow \mathcal {M} \models \psi )$
.
$\mathcal {M} \models \varphi \leftrightarrow \mathcal {M} \models \psi )$
. -
8.
 $\mathcal {M} \models \exists x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi [a]$
for some
$\mathcal {M} \models \exists x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi [a]$
for some
 $a \in M$
.
$a \in M$
. -
9.
 $\mathcal {M} \models \forall x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi [a]$
for all
$\mathcal {M} \models \forall x \varphi (x) \;\;\; \Leftrightarrow \;\;\; \mathcal {M} \models \varphi [a]$
for all
 $a \in M$
.
$a \in M$
. -
10.
 $\mathcal {M} \models \bot \;\;\; \Leftrightarrow \;\;\; \bot $
.
$\mathcal {M} \models \bot \;\;\; \Leftrightarrow \;\;\; \bot $
.
For a set of formulas
![]() $\Sigma $
and a formula
$\Sigma $
and a formula
![]() $\varphi $
, the Tarski semantic consequence relation is defined by
$\varphi $
, the Tarski semantic consequence relation is defined by
if for every Tarski model
![]() $\mathcal {M}$
, we have
$\mathcal {M}$
, we have
![]() $(\mathcal {M} \models \Sigma \; \to \; \mathcal {M} \models \varphi ).$
$(\mathcal {M} \models \Sigma \; \to \; \mathcal {M} \models \varphi ).$
For clarity, we will always refer to the above as Tarski models and Tarski semantics, to keep them apart from T/F-semantics from Definition 2.3. We will also reserve the notation
![]() $\models $
for the Tarski satisfaction and consequence relation and use
$\models $
for the Tarski satisfaction and consequence relation and use
![]() $\models ^T$
and
$\models ^T$
and
![]() $\models ^F$
when referring to T/F-semantics.
$\models ^F$
when referring to T/F-semantics.
Remark 10.2.
-
1. Definition 10.1 should be understood in the framework of
 $\mathrm {PZFC}$
, with all equivalences being strong. The interpretations of relations
$\mathrm {PZFC}$
, with all equivalences being strong. The interpretations of relations
 $R^{\mathcal {M}}$
are not necessarily classical. For example, it could happen that
$R^{\mathcal {M}}$
are not necessarily classical. For example, it could happen that
 $R^{\mathcal {M}}(a)$
is both true and false, in which case we would have
$R^{\mathcal {M}}(a)$
is both true and false, in which case we would have
 $\mathcal {M} \models R(x)[a]$
and
$\mathcal {M} \models R(x)[a]$
and
 $\mathcal {M}\not \models R(x)[a]$
, and subsequently
$\mathcal {M}\not \models R(x)[a]$
, and subsequently
 $\mathcal {M} \models (R(x) \land {\sim } R(x)) [a]$
.
$\mathcal {M} \models (R(x) \land {\sim } R(x)) [a]$
. -
2. The reader may easily verify that the defined connectives are also translated the way we would expect, namely:
 $$ \begin{align*}\mathcal{M} \models \lnot \varphi \;\;\; \Leftrightarrow \;\;\; \lnot( \mathcal{M} \models \varphi),\end{align*} $$
$$ \begin{align*}\mathcal{M} \models \lnot \varphi \;\;\; \Leftrightarrow \;\;\; \lnot( \mathcal{M} \models \varphi),\end{align*} $$
 $$ \begin{align*}\mathcal{M} \models\; ! \varphi \;\;\; \Leftrightarrow \;\;\; \; !( \mathcal{M} \models \varphi),\end{align*} $$
$$ \begin{align*}\mathcal{M} \models\; ! \varphi \;\;\; \Leftrightarrow \;\;\; \; !( \mathcal{M} \models \varphi),\end{align*} $$
 $$ \begin{align*}\mathcal{M} \models \; ? \varphi \;\;\; \Leftrightarrow \;\;\; \; ?( \mathcal{M} \models \varphi).\end{align*} $$
$$ \begin{align*}\mathcal{M} \models \; ? \varphi \;\;\; \Leftrightarrow \;\;\; \; ?( \mathcal{M} \models \varphi).\end{align*} $$
Had we, for example, chosen
 $\lnot $
as the primitive connective in
$\lnot $
as the primitive connective in
 $\mathrm {BS4}$
instead of
$\mathrm {BS4}$
instead of
 $\bot $
, then Definition 10.1 would have given rise to the same logic.
$\bot $
, then Definition 10.1 would have given rise to the same logic. -
3. Linguistically, it is useful to distinguish truth in a Tarski model from satisfaction by a Tarski model. We say “
 $\mathcal {M}$
satisfies
$\mathcal {M}$
satisfies
 $\varphi $
” to express “
$\varphi $
” to express “
 $\mathcal {M} \models \varphi $
” and “
$\mathcal {M} \models \varphi $
” and “
 $\varphi $
is true in M” to express
$\varphi $
is true in M” to express
 $!(\mathcal {M} \models \varphi )$
(which is the same as “
$!(\mathcal {M} \models \varphi )$
(which is the same as “
 $\mathcal {M} \models \;!\varphi $
”). Satisfaction captures the entire truth value of
$\mathcal {M} \models \;!\varphi $
”). Satisfaction captures the entire truth value of
 $\varphi $
in
$\varphi $
in
 $\mathcal {M}$
, and we can use the notation concerning truth values from Section 5 to define the truth value of
$\mathcal {M}$
, and we can use the notation concerning truth values from Section 5 to define the truth value of
 $\varphi $
in a Tarski model
$\varphi $
in a Tarski model
 $\mathcal {M}$
:
$\mathcal {M}$
: 
Theorem 10.3 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
![]() $\mathrm {BS4}$
is sound and complete with respect to Tarski semantics:
$\mathrm {BS4}$
is sound and complete with respect to Tarski semantics:
Remark 10.4. We should note here that the bi-implication is not a strong one, i.e., soundness and completeness only talks about truth in a model, not satisfaction by the model. Indeed, note that
![]() $\vdash _{\mathrm {BS4}}$
is a classical relation, while the Tarski-consequence relation
$\vdash _{\mathrm {BS4}}$
is a classical relation, while the Tarski-consequence relation
![]() $\models $
is not. For example, take any
$\models $
is not. For example, take any
![]() $\varphi $
such that some model
$\varphi $
such that some model
![]() $\mathcal {N} \models \varphi \land {\sim } \varphi $
, and consider the classical excluded middle
$\mathcal {N} \models \varphi \land {\sim } \varphi $
, and consider the classical excluded middle
![]() $\varphi \lor \lnot \varphi $
. Then for every
$\varphi \lor \lnot \varphi $
. Then for every
![]() $\mathcal {M}$
we have
$\mathcal {M}$
we have
![]() $\mathcal {M} \models \varphi \lor \lnot \varphi $
, but one can verify that
$\mathcal {M} \models \varphi \lor \lnot \varphi $
, but one can verify that
![]() $\mathcal {N} \not \models \varphi \lor \lnot \varphi $
. This means that “
$\mathcal {N} \not \models \varphi \lor \lnot \varphi $
. This means that “
![]() $\varphi \lor \lnot \varphi $
” is a tautology, and is not tautology, according to Tarski semantics.
$\varphi \lor \lnot \varphi $
” is a tautology, and is not tautology, according to Tarski semantics.
We will now prove Theorem 10.3. While this could be done by a classical soundness and completeness proof within
![]() ${\mathrm {BZFC}}$
, here we will take a short-cut by recalling that
${\mathrm {BZFC}}$
, here we will take a short-cut by recalling that
![]() $\mathrm {BS4}$
is sound and complete with respect to T/F-semantics (Lemma 2.7), arguing that this fact remains true also when adapted to
$\mathrm {BS4}$
is sound and complete with respect to T/F-semantics (Lemma 2.7), arguing that this fact remains true also when adapted to
![]() $\mathrm {PZFC}$
, and then showing that T/F-models can be ‘simulated’ by Tarski models, and vice versa. This ‘simulation’ principle seems to be an interesting phenomenon in its own right.
$\mathrm {PZFC}$
, and then showing that T/F-models can be ‘simulated’ by Tarski models, and vice versa. This ‘simulation’ principle seems to be an interesting phenomenon in its own right.
First we adapt Definition 2.3:
Definition 10.5 (
 $\mathrm {PZFC}$
)
$\mathrm {PZFC}$
)
A T/F-model is defined as in Definition 2.3, with the added condition that the domain M, all interpretations
![]() $(R^{\mathcal {M}})^+$
and
$(R^{\mathcal {M}})^+$
and
![]() $(R^{\mathcal {M}})^-$
are classical subsets of
$(R^{\mathcal {M}})^-$
are classical subsets of
![]() $M^n$
, and
$M^n$
, and
![]() $=^+$
and
$=^+$
and
![]() $=^-$
are classical subsets of
$=^-$
are classical subsets of
![]() $M\times M$
. Moreover, it is required that for
$M\times M$
. Moreover, it is required that for
![]() $a,b \in M$
we have
$a,b \in M$
we have
![]() $a =^+ b \; \leftrightarrow \;\; !(a=b).$
Footnote
18
$a =^+ b \; \leftrightarrow \;\; !(a=b).$
Footnote
18
Lemma 10.6 (
 $\mathrm {PZFC}$
)
$\mathrm {PZFC}$
)
![]() $\mathrm {BS4}$
is sound and complete with respect to T/F-semantics.
$\mathrm {BS4}$
is sound and complete with respect to T/F-semantics.
Proof. Since all relevant sets and relations are classical, the original proof of Lemma 2.7 can be repeated inside
![]() $\mathrm {PZFC}$
, yielding the desired result.
$\mathrm {PZFC}$
, yielding the desired result.
Lemma 10.7 (
 $\mathrm {PZFC}$
)
$\mathrm {PZFC}$
)
For every Tarski model
![]() $\mathcal {M}$
, there exists a T/F-model
$\mathcal {M}$
, there exists a T/F-model
![]() $\mathcal {M}^{\pm }$
such that for every
$\mathcal {M}^{\pm }$
such that for every
![]() $\varphi $
:
$\varphi $
:
Proof. Let
![]() $\mathcal {M}^{\pm }$
have the same domain as
$\mathcal {M}^{\pm }$
have the same domain as
![]() $\mathcal {M}$
. For every relation symbol R define classical relations
$\mathcal {M}$
. For every relation symbol R define classical relations
![]() $(R^{\mathcal {M}^\pm })^+$
and
$(R^{\mathcal {M}^\pm })^+$
and
![]() $(R^{\mathcal {M}^\pm })^-$
(including equality):
$(R^{\mathcal {M}^\pm })^-$
(including equality):
This definition makes sure that we have:
An induction on the complexity of
![]() $\varphi $
then shows that the two equivalences hold for all sentences. To exhibit an example:
$\varphi $
then shows that the two equivalences hold for all sentences. To exhibit an example:
We leave the details to the reader.
Lemma 10.8 (
 ${\mathrm {BZFC}}$
)
${\mathrm {BZFC}}$
)
For every T/F-model
![]() $\mathcal {N}$
, there exists a Tarski model
$\mathcal {N}$
, there exists a Tarski model
![]() $\mathcal {N}^{4}$
such that for every
$\mathcal {N}^{4}$
such that for every
![]() $\varphi $
:
$\varphi $
:
Proof. First we take care of equality, since the negative relation
![]() $=^-$
of
$=^-$
of
![]() $\mathcal {N}$
is not a priori related to the meta-theoretic
$\mathcal {N}$
is not a priori related to the meta-theoretic
![]() $\neq $
for sets. We define an equivalence relation
$\neq $
for sets. We define an equivalence relation
![]() $\equiv $
on N as follows (appealing to the Anti-Classicality Axiom):
$\equiv $
on N as follows (appealing to the Anti-Classicality Axiom):
It is not hard to verify that
![]() $\equiv $
satisfies Definition 6.1, so if we consider equivalence classes
$\equiv $
satisfies Definition 6.1, so if we consider equivalence classes
![]() $[a] := \{b \in N : a \equiv b\}$
then by Lemma 6.2 we know that:
$[a] := \{b \in N : a \equiv b\}$
then by Lemma 6.2 we know that:
Let the domain of
![]() $\mathcal {N}^4$
be
$\mathcal {N}^4$
be
![]() $ \{ [a] : a \in N\}$
and notice that we have
$ \{ [a] : a \in N\}$
and notice that we have
For every relation symbol R, define the interpretation
![]() $R^{\mathcal {N}^4}$
by:
$R^{\mathcal {N}^4}$
by:
which is again possible by appealing to the Anti-Classicality Axiom. Also note that this is well-defined by the usual arguments.
Finally, we leave it to the reader to verify, by induction on the complexity of
![]() $\varphi $
, that for all
$\varphi $
, that for all
![]() $a_1, \dots , a_n \in N$
we have:
$a_1, \dots , a_n \in N$
we have:
In particular this is true for all sentences
![]() $\varphi $
, completing the proof.
$\varphi $
, completing the proof.
Proof of Theorem 10.3
 $({\mathrm {BZFC}})$
$({\mathrm {BZFC}})$
By Lemma 10.6,
![]() $\mathrm {BS4}$
is sound and complete with respect to T/F-semantics. But by Lemmas 10.7 and 10.8, T/F-models can be replaced by Tarski-models and vice versa. Thus T/F-semantics are equivalent to Tarski-semantics, which completes the proof.
$\mathrm {BS4}$
is sound and complete with respect to T/F-semantics. But by Lemmas 10.7 and 10.8, T/F-models can be replaced by Tarski-models and vice versa. Thus T/F-semantics are equivalent to Tarski-semantics, which completes the proof.
Notice that while most of the theory in this section only required
![]() $\mathrm {PZFC}$
, Lemma 10.8 hinges on the Anti-Classicality Axiom. Indeed, we can now analyse the situation in more detail.
$\mathrm {PZFC}$
, Lemma 10.8 hinges on the Anti-Classicality Axiom. Indeed, we can now analyse the situation in more detail.
Recall from Remark 5.4 that if one is interested in a set theory which has only inconsistent but not incomplete sets, or only incomplete but not inconsistent sets, one can consider these two fragments of the Anti-Classicality Axiom:
-
•
 ${\textbf{ACLA}_{\textbf{cons}}:} \boldsymbol { \;\; \forall x (x^! \subseteq x^?) \land \exists x (x^? \not \subseteq x^!)}$
${\textbf{ACLA}_{\textbf{cons}}:} \boldsymbol { \;\; \forall x (x^! \subseteq x^?) \land \exists x (x^? \not \subseteq x^!)}$
(all sets are consistent, but there is an incomplete set).
-
•
 ${\textbf{ACLA}_{\textbf{comp}} : \;\; \forall x (x^? \subseteq x^!) \land \exists x (x^! \not \subseteq x^?)}$
${\textbf{ACLA}_{\textbf{comp}} : \;\; \forall x (x^? \subseteq x^!) \land \exists x (x^! \not \subseteq x^?)}$
(all sets are complete, but there is an inconsistent set).
Now we consider some fragments of
![]() $\mathrm {BS4}$
, referring to them by the name under which they (or a very similar version) have previously appeared in the literature.
$\mathrm {BS4}$
, referring to them by the name under which they (or a very similar version) have previously appeared in the literature.
Definition 10.9 (PZFC)
-
1.
 $\mathrm{K}3^\to $
: this logic is obtained if in Definition 2.2 we require
$\mathrm{K}3^\to $
: this logic is obtained if in Definition 2.2 we require
 $(R^{\mathcal {M}})^+ \cap (R^{\mathcal {M}})^- = \varnothing $
for all relation symbols R (including equality). The propositional version appeared in [Reference Hazen and Pelletier13].
$(R^{\mathcal {M}})^+ \cap (R^{\mathcal {M}})^- = \varnothing $
for all relation symbols R (including equality). The propositional version appeared in [Reference Hazen and Pelletier13]. -
2.
 $\mathrm{LFI}$
1: this logic is obtained if in Definition 2.2 we require
$\mathrm{LFI}$
1: this logic is obtained if in Definition 2.2 we require
 $(R^{\mathcal {M}})^+ \cup (R^{\mathcal {M}})^- = M$
(the whole domain) for all relation symbols R (including equality). The propositional version appeared in [Reference Carnielli, Coniglio and Marcos10] .
$(R^{\mathcal {M}})^+ \cup (R^{\mathcal {M}})^- = M$
(the whole domain) for all relation symbols R (including equality). The propositional version appeared in [Reference Carnielli, Coniglio and Marcos10] . -
3.
 $\mathrm{FDE}$
: the “
$\mathrm{FDE}$
: the “
 $\to $
”- and “
$\to $
”- and “
 $\bot $
”-free fragment of
$\bot $
”-free fragment of
 $\mathrm {BS4}$
, which appeared in [Reference Asenjo3].
$\mathrm {BS4}$
, which appeared in [Reference Asenjo3]. -
4.
 $\mathrm{K}3$
: the “
$\mathrm{K}3$
: the “
 $\to $
”- and “
$\to $
”- and “
 $\bot $
”-free fragment of
$\bot $
”-free fragment of
 $\mathrm{K}3^\to $
. The propositional version is the widely known three-valued logic with indeterminate truth values of Kleene [Reference Kleene15].
$\mathrm{K}3^\to $
. The propositional version is the widely known three-valued logic with indeterminate truth values of Kleene [Reference Kleene15]. -
5.
 $\mathrm{LP}$
: the “
$\mathrm{LP}$
: the “
 $\to $
”- and “
$\to $
”- and “
 $\bot $
”-free fragment of
$\bot $
”-free fragment of
 $\mathrm{LFI}$
1. The propositional version is the well-known logic of paradox of Priest [Reference Priest22].
$\mathrm{LFI}$
1. The propositional version is the well-known logic of paradox of Priest [Reference Priest22].
K3 and K3
![]() $^\to $
are three-valued logics designed to deal only with incompleteness but not inconsistency, while LP and LFI1 are also three-valued and deal inconsistency but not incompleteness. FDE and
$^\to $
are three-valued logics designed to deal only with incompleteness but not inconsistency, while LP and LFI1 are also three-valued and deal inconsistency but not incompleteness. FDE and
![]() $\mathrm {BS4}$
are four-valued and take incompleteness as well as inconsistency into account. FOL refers to classical logic.
$\mathrm {BS4}$
are four-valued and take incompleteness as well as inconsistency into account. FOL refers to classical logic.
Theorem 10.10 (PZFC)
-
1.
 $ \mathrm {ACLA} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {BS4}} \varphi ). $
$ \mathrm {ACLA} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {BS4}} \varphi ). $
-
2.
 $\mathrm {ACLA}_{\mathrm {cons}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \:\mathrm {K3}^\to } \varphi ). $
$\mathrm {ACLA}_{\mathrm {cons}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \:\mathrm {K3}^\to } \varphi ). $
-
3.
 $\mathrm {ACLA}_{\mathrm {comp}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {LFI1}} \varphi ). $
$\mathrm {ACLA}_{\mathrm {comp}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {LFI1}} \varphi ). $
-
4.
 $\forall x (x^! = x^?) \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \: \mathrm {FOL}} \varphi ). $
$\forall x (x^! = x^?) \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \: \mathrm {FOL}} \varphi ). $
-
5. Exactly one of the above holds.
If we restrict attention to formulas not containing implications or
![]() $\bot $
, we obtain the same result for more familiar systems.
$\bot $
, we obtain the same result for more familiar systems.
Theorem 10.11 (PZFC)
Suppose
![]() $\Sigma $
and
$\Sigma $
and
![]() $\varphi $
do not contain “
$\varphi $
do not contain “
![]() $\to $
” or “
$\to $
” or “
![]() $\bot $
”. Then:
$\bot $
”. Then:
-
1.
 $ \mathrm {ACLA} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {FDE}} \varphi ). $
$ \mathrm {ACLA} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {FDE}} \varphi ). $
-
2.
 $\mathrm {ACLA}_{\mathrm {cons}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \:\mathrm {K3}} \varphi ). $
$\mathrm {ACLA}_{\mathrm {cons}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \:\mathrm {K3}} \varphi ). $
-
3.
 $\mathrm {ACLA}_{\mathrm {comp}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {LP}} \varphi ). $
$\mathrm {ACLA}_{\mathrm {comp}} \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{\: \mathrm {LP}} \varphi ). $
-
4.
 $\forall x (x^! = x^?) \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \: \mathrm {FOL}} \varphi ). $
$\forall x (x^! = x^?) \; \leftrightarrow \; ( \Sigma \models \varphi \;\; \leftrightarrow \;\; \Sigma \vdash _{ \: \mathrm {FOL}} \varphi ). $
-
5. Exactly one of the above holds.
The proofs of these theorems are variations of Theorem 10.3, and are left to the reader.
11. Future work
We would like to conclude by reflecting on a number of possible future research directions.
-
1. Algebraic approach. The logic
 $\mathrm {BS4}$
and set theory in
$\mathrm {BS4}$
and set theory in
 $\mathrm {BS4}$
can be approached using algebraic semantics, in a manner similar to the work of Löwe and Tarafder, [Reference Löwe and Tarafder28], via so-called twist algebras. This can even be used to study an analogue of Boolean-valued models (closely related to forcing over classical models of
$\mathrm {BS4}$
can be approached using algebraic semantics, in a manner similar to the work of Löwe and Tarafder, [Reference Löwe and Tarafder28], via so-called twist algebras. This can even be used to study an analogue of Boolean-valued models (closely related to forcing over classical models of
 ${\mathrm {ZFC}}$
) in the
${\mathrm {ZFC}}$
) in the
 $\mathrm {PZFC}$
- or
$\mathrm {PZFC}$
- or
 ${\mathrm {BZFC}}$
-context. A significant part of this has been done in [Reference Oddsson19, chaps 3 and 8] but there are more things that can be studied.
${\mathrm {BZFC}}$
-context. A significant part of this has been done in [Reference Oddsson19, chaps 3 and 8] but there are more things that can be studied. -
2. Constructive
 ${\mathrm {BZFC}}$
. It is also possible to consider an intuitionistic version of
${\mathrm {BZFC}}$
. It is also possible to consider an intuitionistic version of
 $\mathrm {BS4}$
, defined syntactically by changing axioms 1–14 in Section 2.3 to an intuitionistic version, or semantically by considering Kripke frames with T/F-models at the nodes. In this logic, known as N4 and appearing in [Reference Almukdad and Nelson2],
$\mathrm {BS4}$
, defined syntactically by changing axioms 1–14 in Section 2.3 to an intuitionistic version, or semantically by considering Kripke frames with T/F-models at the nodes. In this logic, known as N4 and appearing in [Reference Almukdad and Nelson2],
 $\varphi \lor {\sim } \varphi $
and
$\varphi \lor {\sim } \varphi $
and
 $\varphi \lor \lnot \varphi $
both need not be true, and
$\varphi \lor \lnot \varphi $
both need not be true, and
 $\varphi \land {\sim } \varphi $
does not lead to a strong contradiction, but
$\varphi \land {\sim } \varphi $
does not lead to a strong contradiction, but
 $\varphi \land \lnot \varphi $
does. Moreover,
$\varphi \land \lnot \varphi $
does. Moreover,
 ${\sim } {\sim } \varphi $
is strongly equivalent to
${\sim } {\sim } \varphi $
is strongly equivalent to
 $\varphi $
while
$\varphi $
while
 $\lnot \lnot \varphi $
is not.
$\lnot \lnot \varphi $
is not.What type of set theory do we obtain in such a logic if we combine the ideas of this paper with constructive systems like
 $\mathrm {IZF}$
or
$\mathrm {IZF}$
or
 $\mathrm {CZF}$
?
$\mathrm {CZF}$
? -
3. Computability theory in
 ${\mathrm {BZFC}}$
. There seems to be a natural application of
${\mathrm {BZFC}}$
. There seems to be a natural application of
 ${\mathrm {BZFC}}$
regarding computability theory. Recall that a Turing machine M computes a set
${\mathrm {BZFC}}$
regarding computability theory. Recall that a Turing machine M computes a set
 $A \subseteq \mathbb {N}$
if for all n:
$A \subseteq \mathbb {N}$
if for all n:-
•
 $n \in A \; \leftrightarrow \; M$
halts on input n and outputs some non-
$n \in A \; \leftrightarrow \; M$
halts on input n and outputs some non-
 $0$
value.
$0$
value. -
•
 $n \notin A \; \leftrightarrow \; M$
halts on input n and outputs
$n \notin A \; \leftrightarrow \; M$
halts on input n and outputs
 $0$
.
$0$
.
Moreover, let us say that M recognizes a set
 $A \subseteq \mathbb {N}$
if for all n:
$A \subseteq \mathbb {N}$
if for all n:-
•
 $n \in A \; \leftrightarrow \; M$
halts on input n and outputs a non-
$n \in A \; \leftrightarrow \; M$
halts on input n and outputs a non-
 $0$
value.
$0$
value.
Classically, every Turing machine recognizes a set, but not every Turing machine computes a set (since it may not halt on a given input). Thus, one cannot use sets
 $A \subseteq \mathbb {N}$
to represent decisions of a machine.
$A \subseteq \mathbb {N}$
to represent decisions of a machine.Let us revisit the situation in BZFC (or even
 $\text {PZFC}+\text {ACLA}_{\text {cons}}$
). Using the same definition as above, it follows from Theorem 5.2 that every Turing machine computes a set. If a given machine does not halt on input n, it means only that it computes an incomplete set A, namely a set for which
$\text {PZFC}+\text {ACLA}_{\text {cons}}$
). Using the same definition as above, it follows from Theorem 5.2 that every Turing machine computes a set. If a given machine does not halt on input n, it means only that it computes an incomplete set A, namely a set for which
 $\lnot (n \in A \lor n \notin A)$
. In particular, the decision process of a machine can be completely described by subsets of
$\lnot (n \in A \lor n \notin A)$
. In particular, the decision process of a machine can be completely described by subsets of
 $\mathbb {N}$
.
$\mathbb {N}$
. -
-
4. Cardinal arithmetic in
 ${\mathrm {BZFC}}$
. It is clear that we need a new notion of cardinality and cardinal numbers to describe the size of non-classical sets. Such a notion should capture the size of the entire structure of a non-classical sets, i.e., the size of the
${\mathrm {BZFC}}$
. It is clear that we need a new notion of cardinality and cardinal numbers to describe the size of non-classical sets. Such a notion should capture the size of the entire structure of a non-classical sets, i.e., the size of the
 $!$
-extension,
$!$
-extension,
 $?$
-extension, and all of the combinations, in one go. Some preliminary work on this has already been done, and we expect that this will lead to a rich theory of cardinals and cardinal arithmetic.
$?$
-extension, and all of the combinations, in one go. Some preliminary work on this has already been done, and we expect that this will lead to a rich theory of cardinals and cardinal arithmetic.
Acknowledgments
We would like to thank Benno van den Berg, Luca Incurvati, Benedikt Löwe, Hitoshi Omori, Andrew Tedder, Giorgio Venturi and Heinrich Wansing for insightful conversations, helpful suggestions and ideas. We would also like to thank the anonymous referee whose thorough reading of an earlier version of this paper has contributed significantly to an improved presentation.