Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T19:35:30.225Z Has data issue: false hasContentIssue false
  • English
  • Français

INFINITARY TABLEAU FOR SEMANTIC TRUTH

Published online by Cambridge University Press:  08 April 2015

TOBY MEADOWS
TOBY MEADOWS*
Affiliation:
Department of Philosophy, University of Aberdeen
*
*DEPARTMENT OF PHILOSOPHY, UNIVERSITY OF ABERDEEN, 50-52 COLLEGE BOUNDS, ABERDEEN, AB24 3DS, UK E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We provide infinitary proof theories for three common semantic theories of truth: strong Kleene, van Fraassen supervaluation and Cantini supervaluation. The value of these systems is that they provide an easy method of proving simple facts about semantic theories. Moreover we shall show that they also give us a simpler understanding of the computational complexity of these definitions and provide a direct proof that the closure ordinal for Kripke’s definition is 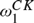$\omega _1^{CK}$. This work can be understood as an effort to provide a proof-theoretic counterpart to Welch’s game-theoretic (Welch, 2009).

Type
Research Article
Information
The Review of Symbolic Logic , Volume 8 , Issue 2 , June 2015 , pp. 207 - 235
Copyright
Copyright © Association for Symbolic Logic 2015 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Barwise, J. (1975). Admissible Sets and Structures. Berlin: Springer-Verlag.Google Scholar
Boolos, G., Burgess, J. P., & Jeffrey, R. C. (2002). Computability and Logic (fourth edition). Melbourne: Cambridge University Press.Google Scholar
Burgess, J. P. (1986). The truth is never simple. The Journal of Symbolic Logic, 51(3), 663–681.Google Scholar
Cantini, A. (1990). A theory of truth arithmetically equivalent to $I\,D_1^1$. The Journal of Symbolic Logic, 55(1), 244–259.Google Scholar
Devlin, K. J. (1984). Constructibility. Berlin: Springer-Verlag.Google Scholar
Halbach, V. (2011). Axiomatic Theories of Truth. London: Cambridge University Press.CrossRefGoogle Scholar
Hjorth, G. (Unpublished notes). Vienna Notes on Effective Descriptive Set Theory and Admissible Sets.Google Scholar
Kripke, S. (1975). Outline of a theory of truth. Journal of Philosophy, 72, 690–716.Google Scholar
Mansfield, R., & Weitkamp, G. (1985). Recursive Aspects of Descriptive Set Theory. Oxford logic guides. New York: Oxford University Press.Google Scholar
Moschovakis, Y. (1974). Elementary Induction on Abstract Structures. Mineola: Dover.Google Scholar
Moschovakis, Y. N. (1980). Descriptive Set Theory. New York: North Holland.Google Scholar
Pohlers, W. (2009). Proof Theory: The First Step into Impredicativity. Berlin: Springer.Google Scholar
Priest, G. (2008). An Introduction to Non-Classical Logic: From If to Is. Melbourne: Cambridge University Press.Google Scholar
Sacks, G. E. (1990). Higher Recursion Theory. Berlin: Springer-Verlag.Google Scholar
Smullyan, R. M. (1968). First-Order Logic. New York: Dover.Google Scholar
Toledo, S. (1975). Tableau Systems for First Order Numbers Theory and Certain Higher Order Theories. Berlin: Springer-Verlag.CrossRefGoogle Scholar
Welch, P. (2009). Games for truth. Bulletin of Symbolic Logic, 15(4), 410–427.Google Scholar