Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-26T19:43:59.427Z Has data issue: false hasContentIssue false
  • English
  • Français

DYNAMIC CONSEQUENCE AND PUBLIC ANNOUNCEMENT

Published online by Cambridge University Press:  14 October 2013

ANDRÉS CORDÓN FRANCO ,
HANS VAN DITMARSCH  and
ANGEL NEPOMUCENO
ANDRÉS CORDÓN FRANCO*
Affiliation:
Universidad de Sevilla
HANS VAN DITMARSCH*
Affiliation:
LORIA, CNRS—Université de Lorraine
ANGEL NEPOMUCENO*
Affiliation:
Universidad de Sevilla
*
*DEPARTMENT OF COMPUTER SCIENCE AND ARTIFICIAL INTELLIGENCE UNIVERSIDAD DE SEVILLA, AV . REINA MERCEDES S/N 41012 SEVILLE, SPAIN E-mail: [email protected]
†LORIA, CNRS—UNIVERSITÉ DE LORRAINE BP 239, 54506 VANDOEUVRE-LÈS-NANCY, FRANCE E-mail: [email protected]
‡DEPARTMENT OF PHILOSOPHY AND LOGIC AND PHILOSOPHY OF SCIENCE UNIVERSIDAD DE SEVILLA, AV . CAMILO JOSÉ CELA S/N 41018 SEVILLE, SPAIN E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In van Benthem (2008), van Benthem proposes a dynamic consequence relation defined as 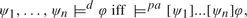${\psi _1}, \ldots ,{\psi _n}{ \models ^d}\phi \,{\rm{iff}}{ \models ^{pa}}[{\psi _1}] \ldots [{\psi _n}]\phi ,$ where the latter denotes consequence in public announcement logic, a dynamic epistemic logic. In this paper we investigate the structural properties of a conditional dynamic consequence relation $\models _{\rm{\Gamma }}^d$ extending van Benthem’s proposal. It takes into account a set of background conditions Γ, inspired by Makinson (2003) wherein Makinson calls this reasoning ‘modulo’ a set Γ. In the presence of common knowledge, conditional dynamic consequence is definable from (unconditional) dynamic consequence. An open question is whether dynamic consequence is compact. We further investigate a dynamic consequence relation for soft instead of hard announcements. Surprisingly, it shares many properties with (hard) dynamic consequence. Dynamic consequence relations provide a novel perspective on reasoning about protocols in multi-agent systems.

Type
Research Article
Information
The Review of Symbolic Logic , Volume 6 , Issue 4 , December 2013 , pp. 659 - 679
Copyright
Copyright © Association for Symbolic Logic 2013 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

BIBLIOGRAPHY

Aliseda, A. (2006). Abductive Reasoning. Logical Investigations into Discovery and Explanation. Synthese Library, Vol. 220. Berlin, Germany: Springer.Google Scholar
Andréka, H., Németi, I., & van Benthem, J. (1998). Modal languages and bounded fragments of predicate logic. Journal of Philosophical Logic, 27(3), 217–274.Google Scholar
Aucher, G. (2011). DEL-sequents for progression. Journal of Applied Non-Classical Logics, 21(3–4), 289–321.Google Scholar
Aucher, G. (2012). DEL-sequents for regression and epistemic planning. Journal of Applied Non-Classical Logics, 22(4), 337–367.Google Scholar
Aucher, G. (2013). Dynamic epistemic logic as a substructural logic. Manuscript to appear.Google Scholar
Aucher, G., Maubert, B., & Schwarzentruber, F. (2012). Generalized DEL-sequents. In del Cerro, L. F., Herzig, A., and Mengin, J., editors. Proceedings of the 13th JELIA. LNCS 7519, Berlin: Springer, pp. 54–66.Google Scholar
Balbiani, P., van Ditmarsch, H., Herzig, A., & de Lima, T. (2010). Tableaux for public announcement logics. Journal of Logic and Computation, 20(1), 55–76.Google Scholar
Baltag, A., Moss, L. S., & Solecki, S. (1998). The logic of public announcements, common knowledge, and private suspicions. In Proceedings of 7th TARK. Morgan Kaufman Publishers Inc. San Francisco, CA. pp. 43–56.Google Scholar
Bolander, T., & Andersen, M. B. (2011). Epistemic planning for single and multi-agent systems. Journal of Applied Non-Classical Logics, 21(1), 9–34.Google Scholar
Cordón-Franco, A., van Ditmarsch, H., Fernández-Duque, D., Gomez-Caminero, E., & Nepomuceno-Fernández, A. (2010). Two mischievous dynamic consequence relations. In Proceedings of the 2nd ILCLI. Zarautz, Spain: University of the Basque Country Press, pp. 197–208.Google Scholar
Etchemendy, J. (1990). The Concept of Logical Consequence. Cambridge, MA: Harvard University Press.Google Scholar
Fagin, R., Halpern, J. Y., Moses, Y., & Vardi, M. Y. (1995). Reasoning About Knowledge. Cambridge, MA: MIT Press.Google Scholar
Fitch, F. B. (1963). A logical analysis of some value concepts. The Journal of Symbolic Logic, 28(2), 135–142.Google Scholar
Gerbrandy, J. D. (1999). Bisimulations on Planet Kripke. PhD Thesis, University of Amsterdam. ILLC Dissertation Series DS-1999-01.Google Scholar
Groeneveld, W. (1995). Logical investigations into dynamic semantics. PhD Thesis, University of Amsterdam. ILLC Dissertation Series DS-1995-18.Google Scholar
Holliday, W., & Icard, T. (2010). Moorean phenomena in epistemic logic. In Beklemishev, L., Goranko, V., and Shehtman, V., editors. Advances in Modal Logic, Vol. 8. London, UK: College Publications, pp. 178–199.Google Scholar
Kracht, M. (2002). Dynamic semantics. In Hamm, F., and Zimmermann, T. E., editors. Semantics. Hamburg, Germany: Buske Verlag, pp. 217–241.Google Scholar
Maffezioli, P., & Negri, S. (2011). A proof theoretical perspective on Public Announcement Logic. Logic and Philosophy of Science, 9, 49–59.Google Scholar
Maffezioli, P., Naibo, A., & Negri, S. (2012). The Church-Fitch knowability paradox in the light of structural proof theory. Synthese. doi: 10.1007/s11229-012-0061-7.Google Scholar
Makinson, D. (2003). Bridges between classical and nonmonotonic logic. Logic Journal of the IGPL, 11, 69–96.Google Scholar
Plaza, J. A. (1989). Logics of public communications. In Proceedings of the 4th ISMIS. Oak Ridge National Laboratory, pp. 201–216.Google Scholar
Restall, G. (2000). An Introduction to Substructural Logics. New York, NY: Routledge.CrossRefGoogle Scholar
Sadzik, T. (2006). Exploring the Iterated Update Universe. Amsterdam, The Netherlands: ILLC. Technical Report PP-2006-18.Google Scholar
Tarski, A. (1936). On the Concept of Logical Consequence. Oxford, UK: Oxford University Press. Reprinted in A. Tarski, Logic, Semantics, Metamathematics (second edition).Google Scholar
van Benthem, J. (1996). Exploring Logical Dynamics. CSLI Publications.Google Scholar
van Benthem, J. (2007). Dynamic logic of belief revision. Journal of Applied Non-Classical Logics, 17(2), 129–155.Google Scholar
van Benthem, J. (2008). Logical dynamics meets logical pluralism? Australasian Journal of Logic, 6, 182–209.CrossRefGoogle Scholar
van Ditmarsch, H., & Kooi, B. (2006). The secret of my success. Synthese, 151, 201–232.CrossRefGoogle Scholar
van Ditmarsch, H., van der Hoek, W., & Kooi, B. (2003). Descriptions of game states. In Mints, G., and Muskens, R., editors. Logic, Games, and Constructive Sets, pp. 43–58. Stanford, CA: CSLI Publications. CSLI Lecture Notes 161.Google Scholar
van Ditmarsch, H., van der Hoek, W., & Kooi, B. (2007). Dynamic Epistemic Logic, Vol. 337. Synthese Library. Springer.Google Scholar
Veltman, F. (1996). Defaults in update semantics. Journal of Philosophical Logic, 25, 221–261.Google Scholar