Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-29T07:15:59.609Z Has data issue: false hasContentIssue false
  • English
  • Français

On Varieties of Literally Idempotent Languages

Published online by Cambridge University Press:  03 June 2008

Ondřej Klíma  and
Libor Polák
Ondřej Klíma
Affiliation:
Department of Mathematics, Masaryk University, Janáčkovo nám 2a, 662 95 Brno, Czech Republic; [email protected]
Libor Polák
Affiliation:
Department of Mathematics, Masaryk University, Janáčkovo nám 2a, 662 95 Brno, Czech Republic; [email protected]
Get access

Abstract

A language L ⊆A* is literally idempotent in case that ua2v ∈ L if and only if uav ∈ L, for each u,v ∈ A*, a ∈ A. Varieties of literally idempotent languages result naturally by taking all literally idempotent languages in a classical (positive) variety or by considering a certain closure operator on classes of languages. We initiate the systematic study of such varieties. Various classes of literally idempotent languages can be characterized using syntactic methods. A starting example is the class of all finite unions of $B^*_1 B^*_2\dots B^*_k$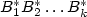 where B1,...,Bk are subsets of a given alphabet A.

Keywords

Literally idempotent languagesvarieties of languages.
Type
Research Article
Information
RAIRO - Theoretical Informatics and Applications , Volume 42 , Issue 3: JM'06 , July 2008 , pp. 583 - 598
Copyright
© EDP Sciences, 2008

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

J. Almeida, Finite Semigroups and Universal Algebra. World Scientific (1994).
Cohen, J., Pin, J.-E. and Perrin, D., On the expressive power of temporal logic. J. Comput. System Sci. 46 (1993) 271–294. CrossRef
Ésik, Z., Extended temporal logic on finite words and wreath product of monoids with distinguished generators, Proc. DLT 02. Lect. Notes Comput. Sci. 2450 (2003) 43–58. CrossRef
Ésik, Z. and Ito, M., Temporal logic with cyclic counting and the degree of aperiodicity of finite automata. Acta Cybernetica 16 (2003) 1–28.
Ésik, Z. and Larsen, K.G., Regular languages defined by Lindström quantifiers. RAIRO-Theor. Inf. Appl. 37 (2003) 197–242.
Kučera, A. and Strejček, J., The stuttering principle revisited. Acta Informatica 41 (2005) 415–434.
Kunc, M., Equationaltion of pseudovarieties of homomorphisms. RAIRO-Theor. Inf. Appl. 37 (2003) 243–254. CrossRef
Peled, D. and Wilke, T., Stutter-invariant temporal properties are expressible without the next-time operator. Inform. Process. Lett. 63 (1997) 243–246. CrossRef
J.-E. Pin, Varieties of Formal Languages. Plenum (1986).
J.-E. Pin, Syntactic semigroups, Chapter 10 in Handbook of Formal Languages, edited by G. Rozenberg and A. Salomaa, Springer (1997).
H. Straubing, On logical descriptions of recognizable languages, Proc. Latin 2002. Lecture Notes Comput. Sci. 2286 (2002) 528–538.
Thérien, D. and Wilke, T., Nesting until and since in linear temporal logic. Theor. Comput. Syst. 37 (2003) 111–131. CrossRef
S. Yu, Regular languages, Chapter 2 in Handbook of Formal Languages, edited by G. Rozenberg and A. Salomaa, Springer (1997).