Published online by Cambridge University Press: 24 April 2007
This note is about functions ƒ : Aω → Bω
whose graph
is recognized by a Büchi finite automaton on the product alphabet A x B. These functions are Baire class 2 in the Baire hierarchy of Borel functions
and it is decidable whether such function are continuous or not.
In 1920 W. Sierpinski showed that a function $f : \mathbb{ R}
\rightarrow \mathbb{R} $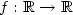 is Baire class 1 if and only if both the
overgraph and the undergraph of f are Fσ. We show that
such characterization is also true for functions on infinite words
if we replace the real ordering by the lexicographical ordering
on Bω. From this we deduce that it is decidable whether
such function are of Baire class 1 or not. We extend this result
to real functions definable by automata in Pisot base.
is Baire class 1 if and only if both the
overgraph and the undergraph of f are Fσ. We show that
such characterization is also true for functions on infinite words
if we replace the real ordering by the lexicographical ordering
on Bω. From this we deduce that it is decidable whether
such function are of Baire class 1 or not. We extend this result
to real functions definable by automata in Pisot base.