Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-18T09:09:36.092Z Has data issue: false hasContentIssue false
  • English
  • Français

Balance properties of the fixed point of the substitutionassociated to quadratic simple Pisot numbers

Published online by Cambridge University Press:  18 July 2007

Ondřej Turek
Ondřej Turek*
Affiliation:
Department of Mathematics, FNSPE, Czech Technical University, Trojanova 13, 120 00 Praha 2, Czech Republic; [email protected]
Get access

Abstract

In this paper we will deal with the balance properties of the infinite binary words associated to β-integers when β is a quadratic simple Pisot number. Those words are the fixed points of the morphisms of the type $\varphi(A)=A^pB$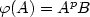, $\varphi(B)=A^q$ 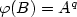for $p\in\mathbb N$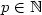, $q\in\mathbb N$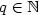, $p\geq q$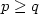, where $\beta=\frac{p+\sqrt{p^2+4q}}{2}$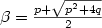. We will prove that such word is t-balanced with $t=1+\left[(p-1)/(p+1-q)\right]$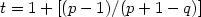. Finally, in the case that p < q it is known [B. Adamczewski, Theoret. Comput. Sci.273 (2002) 197–224] that the fixed point of the substitution $\varphi(A)=A^pB$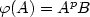, $\varphi(B)=A^q$ 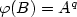is not m-balanced for any m. We exhibit an infinite sequence of pairs of words with the unbalance property.

Keywords

Balance propertysubstitution invariantParry number
Type
Research Article
Information
RAIRO - Theoretical Informatics and Applications , Volume 41 , Issue 2 , April 2007 , pp. 123 - 135
Copyright
© EDP Sciences, 2007

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Adamczewski, B., Balances for fixed points of primitive substitutions. Theoret. Comput. Sci. 273 (2002) 197–224.
Bassino, F., Beta-expansions for cubic Pisot numbers, in LATIN'02, Springer. Lect. notes Comput. Sci. 2286 (2002) 141–152. CrossRef
Berthé, V. and Tijdeman, R., Balance properties of multi-dimensional words. Theoret. Comput. Sci. 60 (1938) 815–866.
Coven, E.M. and Hedlund, G.A., Sequences with minimal block growth. Math. Systems Theory 7 (1973) 138–153. CrossRef
Ch. Frougny, B. Solomyak, Finite beta-expansions. Ergod. Theor. Dyn. Syst. 12 (1992) 713–723. CrossRef
Ch. Frougny, J.P. Gazeau, J. Krejcar, Additive and multiplicative properties of point-sets based on beta-integers. Theoret. Comput. Sci. 303 (2003) 491–516. CrossRef
Ch. Frougny, E. Pelantová, Z. Masáková, Complexity of infinite words associated with beta-expansions. RAIRO-Inf. Theor. Appl. 38 (2004) 163–185. CrossRef
M. Lothaire, Algebraic combinatorics on words. Cambridge University Press (2002).
Morse, M. and Hedlund, G.A., Symbolic dynamics. Amer. J. Math. 60 (1938) 815–866. CrossRef
Morse, M. and Hedlund, G.A., Symbolic dynamics II. Sturmian Trajectories. Amer. J. Math. 62 (1940) 1–42. CrossRef
O. Turek, Complexity and balances of the infinite word of $\beta$ -integers for $\beta = 1+\sqrt{3}$ , in Proc. of WORDS'03, Turku (2003) 138–148.
Vuillon, L., Balanced words. Bull. Belg. Math. Soc. Simon Stevin 10 (2003) 787–805.