Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T17:27:29.374Z Has data issue: false hasContentIssue false
  • English
  • Français

Radial and Latitudinal Gradients in Galactic Cosmic Rays

Published online by Cambridge University Press:  25 April 2016

D. L. Hall ,
J. E. Humble  and
M. L. Duldig
D. L. Hall
Affiliation:
Physics Department, University of Tasmania, GPO Box 252C, Hobart, Tas. 7001
J. E. Humble
Affiliation:
Physics Department, University of Tasmania, GPO Box 252C, Hobart, Tas. 7001
M. L. Duldig
Affiliation:
Australian Antarctic Division, c/o Physics Department, University of Tasmania, GPO Box 252C, Hobart, Tas. 7001
Get access Rights & Permissions [Opens in a new window]

Abstract

We have deduced the yearly averaged value of the solar diurnal variation as observed by a surface muon telescope and three underground muon telescopes over the years 1957 to 1985. This has allowed us to examine the temporal variation in both the latitudinal gradient Gz and the product of the parallel mean free path and the radial gradient 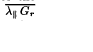of galactic cosmic rays during three consecutive solar cycles. The median rigidities of the primary particles being detected by the telescopes are 50 GV in the case of the surface muon telescope and greater than 150 GV in the case of the underground muon telescopes. We have compared our results with those of a similar study made from observations of the solar diurnal variation by neutron monitors and an ion chamber, which have median rigidities of response between 17 and 70 GV (Bieber and Chen 1991a). The product has a solar magnetic cycle dependence and our values are lower than those observed by neutron monitors, in agreement with the Bieber and Chen observation that reverses after a solar magnetic field reversal, in accordance with drift theories.

Type
High Energy Astrophysics
Information
Publications of the Astronomical Society of Australia , Volume 11 , Issue 2 , August 1994 , pp. 170 - 174
Copyright
Copyright © Astronomical Society of Australia 1994

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Ahluwalia, H. S. and Erickson, J. H., 1969, Acta Phys. Acad. Scient. Hung., 29, supp. 2, 139.Google Scholar
Ahluwalia, H. S. and Riker, J. F., 1987, Planet. Space Sci., 35, 39.CrossRefGoogle Scholar
Baker, C. P., 1988, Honours Thesis, University of Tasmania.Google Scholar
Bieber, J. W. and Chen, J., 1991a, Astrophys. J., 372, 301.CrossRefGoogle Scholar
Bieber, J. W. and Chen, J., 1991b, Proc. 22nd Int. Cosmic Ray Conf., Dublin, Vol. 3, p. 525.Google Scholar
Duldig, M. L., 1990, Proc. 21st Int. Cosmic Ray Conf., Adelaide, Vol. 7, p. 288.Google Scholar
Fenton, A. G., Jacklyn, R. M. and Taylor, R. B., 1961, Nuovo Cimento, 22, 3985.CrossRefGoogle Scholar
Forman, M. A., 1970, Planet. Space Sci., 18, 25.CrossRefGoogle Scholar
Forman, M. A. and Gleeson, L. J., 1975, Astrophys. Space Sci., 32, 77.CrossRefGoogle Scholar
Fujimoto, K., Inoue, A., Murakami, K. and Nagashima, K., 1984, Cosmic Ray Research Lab. Rep. No. 9, Nagoya.Google Scholar
Hall, D. L., Humble, J. E. and Duldig, M. L., 1993, Proc. 23rd Int. Cosmic Ray Conf., Calgary, Vol. 3, p. 648.Google Scholar
Humble, J. E., 1971, Ph.D. thesis, University of Tasmania.Google Scholar
Parsons, N. R., 1959, Ph.D. thesis, University of Tasmania.Google Scholar