Given a sequence of polynomials 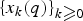 , define the transformation
, define the transformation
for
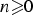
. In this paper, we obtain the relation between the Jacobi continued fraction of the ordinary generating function of
yn(
q) and that of
xn(
q). We also prove that the transformation preserves
q-TP
r+1 (
q-TP) property of the Hankel matrix

, in particular for
r = 2,3, implying the
r-
q-log-convexity of the sequence

. As applications, we can give the continued fraction expressions of Eulerian polynomials of types
A and
B, derangement polynomials types
A and
B, general Eulerian polynomials, Dowling polynomials and Tanny-geometric polynomials. In addition, we also prove the strong
q-log-convexity of derangement polynomials type
B, Dowling polynomials and Tanny-geometric polynomials and 3-
q-log-convexity of general Eulerian polynomials, Dowling polynomials and Tanny-geometric polynomials. We also present a new proof of the result of Pólya and Szegö about the binomial convolution preserving the Stieltjes moment property and a new proof of the result of Zhu and Sun on the binomial transformation preserving strong
q-log-convexity.