Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-24T04:54:04.442Z Has data issue: false hasContentIssue false
  • English
  • Français

Twisted Brauer monoids

Part of: Semigroups Algebraic combinatorics Combinatorics

Published online by Cambridge University Press:  10 April 2018

Igor Dolinka  and
James East
Igor Dolinka
Affiliation:
Department of Mathematics and Informatics, University of Novi Sad, Trg Dositeja Obradovića 4, 21101 Novi Sad, Serbia ([email protected])
James East
Affiliation:
Centre for Research in Mathematics and School of Computing, Engineering and Mathematics, Western Sydney University, Locked Bag 1797, Penrith NSW 2751, Australia ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We investigate the structure of the twisted Brauer monoid 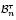, comparing and contrasting it with the structure of the (untwisted) Brauer monoid 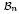. We characterize Green's relations and pre-orders on 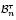, describe the lattice of ideals and give necessary and sufficient conditions for an ideal to be idempotent generated. We obtain formulae for the rank (smallest size of a generating set) and (where applicable) the idempotent rank (smallest size of an idempotent generating set) of each principal ideal; in particular, when an ideal is idempotent generated, its rank and idempotent rank are equal. As an application of our results, we describe the idempotent generated subsemigroup of 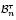(which is not an ideal), as well as the singular ideal of 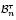(which is neither principal nor idempotent generated), and we deduce that the singular part of the Brauer monoid 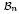is idempotent generated, a result previously proved by Maltcev and Mazorchuk.

Keywords

Brauer monoidstwisted Brauer monoidsidempotentsidealsrankidempotent rank

MSC classification

Secondary: 20M20: Semigroups of transformations, etc. 20M17: Regular semigroups 05A18: Partitions of sets 05E15: Combinatorial aspects of groups and algebras
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 148 , Issue 4 , August 2018 , pp. 731 - 750
Copyright
Copyright © Royal Society of Edinburgh 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)