A symmetrized conjugacy scheme for orthogonal expansions

Abstract

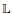We establish a symmetrization procedure in the context of general orthogonal expansions associated with a second-order differential operator L, a Laplacian. Combining with a unified conjugacy scheme from an earlier paper by Nowak and Stempak permits, using a suitable embedding, a differential-difference Laplacian to be associated with the initially given orthogonal system of eigenfunctions of L, so that the resulting extended conjugacy scheme has the natural classical shape. This means, in particular, that the related partial derivatives decomposing are skew-symmetric in an appropriate L² space and they commute with Riesz transforms and conjugate Poisson integrals. The results also shed new light on the issue of defining higher-order Riesz transforms for general orthogonal expansions.