Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Grinfeld, M.
and
Novick-Cohen, A.
1995.
Counting stationary solutions of the Cahn–Hilliard equation by transversality arguments.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 125,
Issue. 2,
p.
351.
Novick-Cohen, A.
and
Zheng, Songmu
1996.
The Penrose–Fife-type equations: counting the one-dimensional stationary solutions.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 126,
Issue. 3,
p.
483.
Cahn, J. W.
Elliott, C. M.
and
Novick-Cohen, A.
1996.
The Cahn–Hilliard equation with a concentration dependent mobility: motion by minus the Laplacian of the mean curvature.
European Journal of Applied Mathematics,
Vol. 7,
Issue. 3,
p.
287.
Fife, Paul C.
and
Wang, Xiao-Ping
1998.
Periodic structures in a van der Waals fluid.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 128,
Issue. 2,
p.
235.
Grinfeld, M.
and
Novick-Cohen, A.
1999.
The viscous Cahn-Hilliard equation: Morse decomposition and structure of the global attractor.
Transactions of the American Mathematical Society,
Vol. 351,
Issue. 6,
p.
2375.
Bertozzi, A L
Grün, G
and
Witelski, T P
2001.
Dewetting films: bifurcations and concentrations.
Nonlinearity,
Vol. 14,
Issue. 6,
p.
1569.
Bertozzi, Andrea L.
and
Bowen, Mark
2002.
Modern Methods in Scientific Computing and Applications.
p.
31.
Norbury, John
Wei, Juncheng
and
Winter, Matthias
2002.
Existence and stability of singular patterns in a Ginzburg Landau equation coupled with a mean field.
Nonlinearity,
Vol. 15,
Issue. 6,
p.
2077.
King, Belinda B.
Stein, Oliver
and
Winkler, Michael
2003.
A fourth-order parabolic equation modeling epitaxial thin film growth.
Journal of Mathematical Analysis and Applications,
Vol. 286,
Issue. 2,
p.
459.
Cartes, C.
Clerc, M. G.
and
Soto, R.
2004.
van der Waals normal form for a one-dimensional hydrodynamic model.
Physical Review E,
Vol. 70,
Issue. 3,
Wu, Hao
and
Zheng, Songmu
2004.
Convergence to equilibrium for the Cahn–Hilliard equation with dynamic boundary conditions.
Journal of Differential Equations,
Vol. 204,
Issue. 2,
p.
511.
Argentina, M.
Clerc, M. G.
Rojas, R.
and
Tirapegui, E.
2005.
Coarsening dynamics of the one-dimensional Cahn-Hilliard model.
Physical Review E,
Vol. 71,
Issue. 4,
Zheng, Songmu
and
Milani, Albert
2005.
Global attractors for singular perturbations of the Cahn–Hilliard equations.
Journal of Differential Equations,
Vol. 209,
Issue. 1,
p.
101.
Korostyshevskiy, Valeriy R.
and
Wanner, Thomas
2007.
A Hermite spectral method for the computation of homoclinic orbits and associated functionals.
Journal of Computational and Applied Mathematics,
Vol. 206,
Issue. 2,
p.
986.
Novick-Cohen, Amy
2008.
Vol. 4,
Issue. ,
p.
201.
Zhang, Yanyan
2009.
The steady states and convergence to equilibria for a 1-D chemotaxis model with volume-filling effect.
Mathematical Methods in the Applied Sciences,
p.
n/a.
Zhang, Yanyan
2009.
Counting the stationary states and the convergence to equilibrium for the 1-D thin film equation.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 71,
Issue. 5-6,
p.
1425.
Bonfoh, Ahmed
Grasselli, Maurizio
and
Miranville, Alain
2010.
Singularly perturbed 1D Cahn–Hilliard equation revisited.
Nonlinear Differential Equations and Applications NoDEA,
Vol. 17,
Issue. 6,
p.
663.
Bonfoh, Ahmed
2011.
The viscous Cahn–Hilliard equation with inertial term.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 74,
Issue. 3,
p.
946.
Li, Fang
and
Zhang, Yanyan
2013.
On steady-state solutions of a 1-D chemotaxis model with volume-filling effect.
Journal of Mathematical Analysis and Applications,
Vol. 408,
Issue. 1,
p.
1.
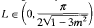 there is an even number of such solutions and for a.e.
there is an even number of such solutions and for a.e.  there is an odd number of such solutions. If m lies within the metastable region, then for a.e. L > 0 there is an even number of solutions. Furthermore, we prove that for all values of m, there are no secondary bifurcations.
there is an odd number of such solutions. If m lies within the metastable region, then for a.e. L > 0 there is an even number of solutions. Furthermore, we prove that for all values of m, there are no secondary bifurcations.