Singular limits and properties of solutions of some degenerate elliptic and parabolic equations

Abstract

Let n ⩾ 3, 0 ⩽ m < n − 2/n, ρ₁ > 0, $\beta>\beta_{0}^{(m)}=(({m\rho_{1}})/({n-2-nm}))$, α_m = ((2β + ρ₁)/(1 − m)) and α = 2β+ρ₁. For any λ > 0, we prove the uniqueness of radially symmetric solution υ^(m) of Δ(υ^m/m) + α_mυ + βx · ∇υ = 0, υ > 0, in ℝⁿ∖{0} which satisfies 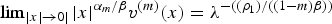$\lim\nolimits_{|x|\to 0|}|x|^{\alpha _m/\beta }v^{(m)}(x) = \lambda ^{-((\rho _1)/((1-m)\beta ))}$ and obtain higher order estimates of υ^(m) near the blow-up point x = 0. We prove that as m → 0⁺, υ^(m) converges uniformly in C²(K) for any compact subset K of ℝⁿ∖{0} to the solution υ of Δlog υ + α υ + β x·∇ υ = 0, υ > 0, in ℝⁿ\{0}, which satisfies 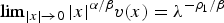$\lim\nolimits_{ \vert x \vert \to 0} \vert x \vert ^{{\alpha}/{\beta}}v(x)=\lambda^{-{\rho_{1}}/{\beta}}$. We also prove that if the solution u^(m) of u_t = Δ (u^m/m), u > 0, in (ℝⁿ∖{0}) × (0, T) which blows up near {0} × (0, T) at the rate 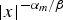$ \vert x \vert ^{-{\alpha_{m}}/{\beta}}$ satisfies some mild growth condition on (ℝⁿ∖{0}) × (0, T), then as m → 0⁺, u^(m) converges uniformly in C^{2 + θ, 1 + θ/2}(K) for some constant θ ∈ (0, 1) and any compact subset K of (ℝⁿ∖{0}) × (0, T) to the solution of u_t = Δlog u, u > 0, in (ℝⁿ∖{0}) × (0, T). As a consequence of the proof, we obtain existence of a unique radially symmetric solution υ⁽⁰⁾ of Δ log υ + α υ + β x·∇ υ = 0, υ > 0, in ℝⁿ∖{0}, which satisfies $\lim\nolimits_{ \vert x \vert \to 0} \vert x \vert ^{{\alpha}/{\beta}}v(x)=\lambda^{-{\rho_{1}}/{\beta}}$.