Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Zhang, Zhengce
and
Li, Zhenjie
2011.
An optimal Liouville-type theorem of the quasilinear parabolic equation with a -Laplace operator.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 74,
Issue. 16,
p.
5735.
Liu, Wenjun
2011.
Extinction properties of solutions for a class of fast diffusive -Laplacian equations.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 74,
Issue. 13,
p.
4520.
Zhang, Zhengce
and
Li, Zhenjie
2012.
A universal bound for radial solutions of the quasilinear parabolic equation with p-Laplace operator.
Journal of Mathematical Analysis and Applications,
Vol. 385,
Issue. 1,
p.
125.
Stan, Diana
and
Vázquez, Juan Luis
2013.
Asymptotic behaviour of the doubly nonlinear diffusion equation on bounded domains.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 77,
Issue. ,
p.
1.
Düzgün, Fatma Gamze
Mosconi, Sunra
and
Vespri, Vincenzo
2019.
Anisotropic Sobolev embeddings and the speed of propagation for parabolic equations.
Journal of Evolution Equations,
Vol. 19,
Issue. 3,
p.
845.
Avelin, Benny
Kuusi, Tuomo
and
Nyström, Kaj
2019.
Boundary behavior of solutions to the parabolic p-Laplace equation.
Analysis & PDE,
Vol. 12,
Issue. 1,
p.
1.
Sánchez, Justino
2020.
Asymptotic behavior of solutions of a k-Hessian evolution equation.
Journal of Differential Equations,
Vol. 268,
Issue. 4,
p.
1840.
Gutiérrez, Susana
and
de Laire, André
2021.
Self-similar shrinkers of the one-dimensional Landau–Lifshitz–Gilbert equation.
Journal of Evolution Equations,
Vol. 21,
Issue. 1,
p.
473.
Liu, Zhipeng
and
Ji, Shanming
2025.
Classification of self-similar solutions of the degenerate polytropic filtration equations.
Acta Mathematica Scientia,
Vol. 45,
Issue. 2,
p.
615.


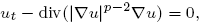
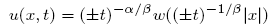
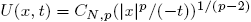 .
.