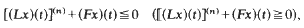Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bainov, D.D.
Myshkis, A.D.
and
Zahariev, A.I.
1988.
Oscillatory properties of the solutions of linear equations of neutral type.
Bulletin of the Australian Mathematical Society,
Vol. 38,
Issue. 2,
p.
255.
Baĭnov, D. D.
Myshkis, A. D.
and
Zahariev, A. I.
1989.
On the oscillatory properties of the solutions of nonlinear neutral functional differential equations of second order.
Hiroshima Mathematical Journal,
Vol. 19,
Issue. 1,