Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kostov, Vladimir Petrov
2016.
О кратных нулях частичной тэта-функции.
Функциональный анализ и его приложения,
Vol. 50,
Issue. 2,
p.
84.
Bohdanov, Anton
and
Vishnyakova, Anna
2016.
On the conditions for entire functions related to the partial theta function to belong to the Laguerre–Pólya class.
Journal of Mathematical Analysis and Applications,
Vol. 434,
Issue. 2,
p.
1740.
Kostov, V. P.
2016.
On multiple zeros of a partial theta function.
Functional Analysis and Its Applications,
Vol. 50,
Issue. 2,
p.
153.
Kostov, Vladimir Petrov
2016.
On a partial theta function and its spectrum.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 146,
Issue. 3,
p.
609.
KIM, BYUNGCHAN
and
LOVEJOY, JEREMY
2017.
PARTIAL INDEFINITE THETA IDENTITIES.
Journal of the Australian Mathematical Society,
Vol. 102,
Issue. 2,
p.
255.
Nguyen, Thu Hien
and
Vishnyakova, Anna
2018.
On the entire functions from the Laguerre–Pólya class having the decreasing second quotients of Taylor coefficients.
Journal of Mathematical Analysis and Applications,
Vol. 465,
Issue. 1,
p.
348.
Kostov, V. P.
2018.
A Separation in Modulus Property of the Zeros of a Partial Theta Function.
Analysis Mathematica,
Vol. 44,
Issue. 4,
p.
501.
Nguyen, Thu Hien
and
Vishnyakova, Anna
2019.
On a necessary condition for an entire function with the increasing second quotients of Taylor coefficients to belong to the Laguerre-Pólya class.
Journal of Mathematical Analysis and Applications,
Vol. 480,
Issue. 2,
p.
123433.
Nguyen, Thu Hien
and
Vishnyakova, Anna
2020.
On the Closest to Zero Roots and the Second Quotients of Taylor Coefficients of Entire Functions from the Laguerre–Pólya I Class.
Results in Mathematics,
Vol. 75,
Issue. 3,
Kostov, Vladimir Petrov
2020.
Partial Theta Function and Separation in Modulus Property of its Zeros.
Vietnam Journal of Mathematics,
Vol. 48,
Issue. 1,
p.
145.
Nguyen, Thu Hien
and
Vishnyakova, Anna
2021.
On the entire functions from the Laguerre–Pólya I class having the increasing second quotients of Taylor coefficients.
Journal of Mathematical Analysis and Applications,
Vol. 498,
Issue. 1,
p.
124955.
Nguyen, T. H.
and
Vishnyakova, A.
2021.
On entire functions from the Laguerre-Polya I class with non-monotonic second quotients of Taylor coefficients.
Matematychni Studii,
Vol. 56,
Issue. 2,
p.
149.
Nguyen, Thu Hien
2022.
On the Conditions for a Special Entire Function Related to the Partial Theta-Function and the q-Kummer Functions to Belong to the Laguerre–Pólya Class.
Computational Methods and Function Theory,
Vol. 22,
Issue. 1,
p.
7.


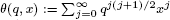 defines a
defines a 