Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-28T02:13:46.506Z Has data issue: false hasContentIssue false
  • English
  • Français

On supercritical nonlinear Schrödinger equations with ellipse-shaped potentials

Part of: Elliptic equations and systems Partial differential equations

Published online by Cambridge University Press:  02 December 2019

Jianfu Yang  and
Jinge Yang
Jianfu Yang
Affiliation:
Department of Mathematics, Jiangxi Normal University, Nanchang, Jiangxi330022, P. R. China ([email protected])
Jinge Yang*
Affiliation:
School of Sciences, Nanchang Institute of Technology, Nanchang330099, P. R. China ([email protected])
*
*Corresponding author.
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we study the existence and concentration of normalized solutions to the supercritical nonlinear Schrödinger equation

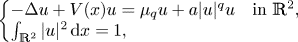\[ \left\{\begin{array}{@{}ll} -\Delta u + V(x) u = \mu_q u + a \vert u \vert ^q u & {\rm in}\ \mathbb{R}^2,\\ \int_{\mathbb{R}^2} \vert u \vert ^2\,{\rm d}x =1, & \end{array} \right.\]
where μq is the Lagrange multiplier. For ellipse-shaped potentials V(x), we show that for q > 2 close to 2, the equation admits an excited solution uq, and furthermore, we study the limiting behaviour of uq when q → 2+. Particularly, we describe precisely the blow-up formation of the excited state uq.

Keywords

Nonlinear elliptic equationL2 supercriticalconstrained minimizationGross–Pitaevskii functionalellipse-shaped potential

MSC classification

Primary: 35B38: Critical points
Secondary: 35J20: Variational methods for second-order elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 150 , Issue 6 , December 2020 , pp. 3187 - 3215
Check for updates
Copyright
Copyright © 2019 The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Bartsch, T. and Soave, N.. A natural constraint approach to normalized solutions of nonlinear Schrödinger equations and systems. J. Funct. Anal. 272 (2017), 4998–5037.CrossRefGoogle Scholar
2Bartsch, T. and deValeriola, S.. Normalized solutions of nonlinear Schrödinger equations. Arch. Math. 100 (2012), 75–83.CrossRefGoogle Scholar
3Bellazzini, J. and Jeanjean, L.. On dipolar quantum gases in the unstable regime. SIAM J. Math. Anal. 48 (2016), 2028–2058.CrossRefGoogle Scholar
4Berestycki, H. and Lions, P. L.. Nonlinear scalar field equations, I and II. Arch. Ration. Mech. Anal. 82 (1983), 313–346, 347–375.CrossRefGoogle Scholar
5Cazenave, T.. Semilinear Schrödinger equations, Courant Lecture Notes in Mathematics, vol. 10 (New York: Courant Institute of Mathematical Science/AMS, 2003).Google Scholar
6Dalfovo, F., Giorgini, S., Pitaevskii, L. P. and Stringari, S.. Theory of Bose–Einstein condensation in trapped gases. Rev. Mod. Phys. 71 (1999), 463–512.CrossRefGoogle Scholar
7Ghoussoub, N.. Duality and perturbation methods in critical point theory, Cambridge Tracts in Mathematics, vol. 107 (Cambridge: Cambridge University Press, 1993), with appendices by David Robinson.CrossRefGoogle Scholar
8Huepe, C., Metens, S., Dewel, G., Borckmans, P. and Brachet, M. E.. Decay rates in attractive Bose–Einstein condensates. Phys. Rev. Lett. 82 (1999), 1616–1619.CrossRefGoogle Scholar
9Guo, H. and Zhou, H.. A constrained variational problem arising in attractive Bose–Einstein condensate with ellipse-shaped potential. Appl. Math. Lett 87 (2019), 35–41.CrossRefGoogle Scholar
10Guo, Y. J. and Seiringer, R.. On the mass concentration for Bose–Einstein condensates with attractive interactions. Lett. Math. Phys. 104 (2014), 141–156.CrossRefGoogle Scholar
11Guo, Y. J., Zeng, X. Y. and Zhou, H. S.. Energy estimates and symmetry breaking in attractive Bose–Einstein condensates with ring-shaped potentials. Ann. I. H. Poincare-AN 33 (2016), 809–828.CrossRefGoogle Scholar
12Guo, Y. J., Zeng, X. Y. and Zhou, H. S.. Concentration behavior of standing waves for almost mass critical nonlinear Schrödinger equations. J. Differ. Equ. 256 (2014), 2079–2100.CrossRefGoogle Scholar
13Jeanjean, L.. Existence of solutions with prescribed norm for semilinear elliptic equations. Nonlinear Anal. 28 (1997), 1633–1659.CrossRefGoogle Scholar
14Kagan, Y., Muryshev, A. E. and Shlyapnikov, G. V.. Collapse and Bose–Einstein condensation in a trapped Bose gas with negative scattering length. Phys. Rev. Lett. 81 (1998), 933–937.CrossRefGoogle Scholar
15Kwong, M. K.. Uniqueness of positive solutions of ∇ u − u + u p = 0 in ℝN. Arch. Ration. Mech. Anal. 105 (1989), 243–266.CrossRefGoogle Scholar
16Han, Q. and Lin, F. H.. Elliptic partial differential equations: second edition, Courant Lecture Notes in Mathematics, vol. 1 (New York: Courant Institute of Mathematical Science/AMS, 2011).Google Scholar
17Sackett, C. A., Stoof, H. T. C. and Hulet, R. G.. Growth and collapse of a Bose–Einstein condensate with attractive interactions. Phys. Rev. Lett. 80 (1998), 2031.CrossRefGoogle Scholar
18Weinstein, M. I.. Nonlinear Schrödinger equations and sharp interpolation estimates, Commun. Math. Phys. 87 (1983), 567–576.Google Scholar
19Willem, M.. Minimax theorems (Boston, Basel, Berlin: Birkhauser, 1996).CrossRefGoogle Scholar
20Yang, Jianfu and Yang, Jinge. Existence and mass concentration of pseudo-relativistic Hartree equation. J. Math. Phys. 58 (2017), 081501.CrossRefGoogle Scholar