Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T08:38:54.914Z Has data issue: false hasContentIssue false
  • English
  • Français

Nodal vector solutions with clustered peaks for nonlinear elliptic equations in ℝ3

Part of: Elliptic equations and systems Partial differential equations

Published online by Cambridge University Press:  01 July 2016

Qihan He  and
Chunhua Wang
Qihan He
Affiliation:
School of Mathematics and Statistics and Hubei Key Laboratory of Mathematical Sciences, Central China Normal University, Wuhan 430079, People's Republic of China(, [email protected]; , [email protected])
Chunhua Wang*
Affiliation:
School of Mathematics and Statistics and Hubei Key Laboratory of Mathematical Sciences, Central China Normal University, Wuhan 430079, People's Republic of China(, [email protected]; , [email protected])
*
†Corresponding author.
Get access Rights & Permissions [Opens in a new window]

Extract

We study the following coupled nonlinear Schr¨odinger system in ℝ3:

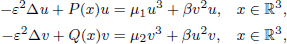
where μ1 > 0, μ2 > 0 and β ∈ ℝ is a coupling constant. Irrespective of whether the system is repulsive or attractive, we prove that it has nodal semi-classical segregated or synchronized bound states with clustered spikes for sufficiently small ε under some additional conditions on P(x), Q(x) and β. Moreover, the number of this type of solutions will go to infinity as ε → 0+.

Keywords

clustered peaksnodal vector solutionsnonlinear coupled equations

MSC classification

Secondary: 35J10: Schrödinger operator 35B99: None of the above, but in this section 35J60: Nonlinear elliptic equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 146 , Issue 5 , October 2016 , pp. 947 - 982
Copyright
Copyright © Royal Society of Edinburgh 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)