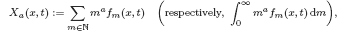Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Bailleul, I. F.
2011.
Spatial coagulation with bounded coagulation rate.
Journal of Evolution Equations,
Vol. 11,
Issue. 3,
p.
675.
Rezakhanlou, Fraydoun
2014.
Pointwise Bounds for the Solutions of the Smoluchowski Equation with Diffusion.
Archive for Rational Mechanics and Analysis,
Vol. 212,
Issue. 3,
p.
1011.
Breden, Maxime
Desvillettes, Laurent
and
Fellner, Klemens
2017.
Smoothness of moments of the solutions of discrete coagulation equations with diffusion.
Monatshefte für Mathematik,
Vol. 183,
Issue. 3,
p.
437.
Singh, Mehakpreet
and
Kaur, Gurmeet
2019.
Convergence analysis of finite volume scheme for nonlinear aggregation population balance equation.
Mathematical Methods in the Applied Sciences,
Vol. 42,
Issue. 9,
p.
3236.
Singh, Mehakpreet
Matsoukas, Themis
and
Walker, Gavin
2020.
Mathematical analysis of finite volume preserving scheme for nonlinear Smoluchowski equation.
Physica D: Nonlinear Phenomena,
Vol. 402,
Issue. ,
p.
132221.
Singh, Mehakpreet
Matsoukas, Themis
and
Walker, Gavin
2021.
Two moments consistent discrete formulation for binary breakage population balance equation and its convergence.
Applied Numerical Mathematics,
Vol. 166,
Issue. ,
p.
76.
Singh, Mehakpreet
Nayak, R.K.
and
Walker, Gavin
2022.
Convergence analysis of volume preserving scheme for mass based coalescence equation.
Applied Numerical Mathematics,
Vol. 173,
Issue. ,
p.
365.
Singh, Mehakpreet
and
Walker, Gavin
2022.
Finite volume approach for fragmentation equation and its mathematical analysis.
Numerical Algorithms,
Vol. 89,
Issue. 2,
p.
465.
Singh, Mehakpreet
Walker, Gavin
and
Randade, Vivek
2022.
New formulations and convergence analysis for reduced tracer mass fragmentation model: an application to depolymerization.
ESAIM: Mathematical Modelling and Numerical Analysis,
Vol. 56,
Issue. 3,
p.
943.
Ansari, Zeeshan
Rae, Mitchell
and
Singh, Mehakpreet
2024.
Two moments preserving sectional approach for an enzymatic coagulation equation.
Physics of Fluids,
Vol. 36,
Issue. 6,
Ansari, Zeeshan
Rae, Mitchell
Kumar, Jitendra
and
Singh, Mehakpreet
2024.
Optimizing numerical performance of enzymatic coagulation models: Insight into proteolysis and gelation dynamics.
Physics of Fluids,
Vol. 36,
Issue. 11,
Singh, Mehakpreet
Sriwastav, Nikhil
and
Shardt, Orest
2024.
Efficient mass-preserving finite volume approach for the rennet-induced coagulation equation.
Chaos, Solitons & Fractals,
Vol. 181,
Issue. ,
p.
114692.
Sriwastav, Nikhil
Das, Ashok
Shardt, Orest
Kumar, Jitendra
and
Singh, Mehakpreet
2025.
A meshfree approach for the rennet-induced coagulation equation: Spline based multistage Bernstein collocation method and its convergence analysis.
Applied Mathematical Modelling,
Vol. 143,
Issue. ,
p.
116035.
 bounds on moments
bounds on moments