Article contents
Higher order Wirtinger inequalities
Published online by Cambridge University Press: 14 November 2011
Synopsis
Wirtinger-type inequalities of order n are inequalities between quadratic forms involving derivatives of order k ≦ n of admissible functions in an interval (a, b). Several methods for establishing these inequalities are investigated, leading to improvements of classical results as well as systematic generation of new ones. A Wirtinger inequality for Hamiltonian systems is obtained in which standard regularity hypotheses are weakened and singular intervals are permitted, and this is employed to generalize standard inequalities for linear differential operators of even order. In particular second order inequalities of Beesack's type are developed, in which the admissible functions satisfy only the null boundary conditions at the endpoints of [a, b] and b does not exceed the first systems conjugate point 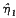 (a) of a. Another approach is presented involving the standard minimization theory of quadratic forms and the theory of “natural boundary conditions”. Finally, inequalities of order n + k are described in terms of (n, n)-disconjugacy of associated 2nth order differential operators.
(a) of a. Another approach is presented involving the standard minimization theory of quadratic forms and the theory of “natural boundary conditions”. Finally, inequalities of order n + k are described in terms of (n, n)-disconjugacy of associated 2nth order differential operators.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 85 , Issue 1-2 , 1980 , pp. 87 - 110
- Copyright
- Copyright © Royal Society of Edinburgh 1980
References
- 4
- Cited by


