Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-13T10:19:08.129Z Has data issue: false hasContentIssue false
  • English
  • Français

Deforming an є-close-to-hyperbolic metric to a hyperbolic metric

Part of: Global differential geometry

Published online by Cambridge University Press:  18 April 2018

Pedro Ontaneda
Pedro Ontaneda*
Affiliation:
Department of Mathematical Sciences, Binghamton University, PO Box 6000, Binghamton, NY 13902-6000, USA ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We show how to deform a metric of the form g = gr + dr2 to a metric 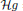= Hr + dr2, which is a hyperbolic metric for r less than some fixed λ, and coincides with g for r large. Here by hyperbolic metric we mean a metric of constant sectional curvature equal to -1. We study the extent to which 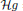is close to hyperbolic everywhere, if we assume g is close to hyperbolic. A precise definition of the close to hyperbolic concept is given. We also deal with a one-parameter version of this problem. The results in this paper are used in the problem of smoothing Charney–Davis strict hyperbolizations.

Keywords

hyperbolic metricmetric deformationhyperbolization

MSC classification

Secondary: 53C20: Global Riemannian geometry, including pinching
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 148 , Issue 3 , June 2018 , pp. 629 - 641
Copyright
Copyright © Royal Society of Edinburgh 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)