Compact embedding results of Sobolev spaces and positive solutions to an elliptic equation
Published online by Cambridge University Press: 11 April 2016
Extract
Using a regular Borel measure μ ⩾ 0 we derive a proper subspace 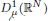 of the commonly used Sobolev space D1(ℝN) when N ⩾ 3. The space
of the commonly used Sobolev space D1(ℝN) when N ⩾ 3. The space 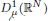 resembles the standard Sobolev space H1(Ω) when Ω is a bounded region with a compact Lipschitz boundary ∂Ω. An equivalence characterization and an example are provided that guarantee that
resembles the standard Sobolev space H1(Ω) when Ω is a bounded region with a compact Lipschitz boundary ∂Ω. An equivalence characterization and an example are provided that guarantee that 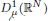 is compactly embedded into L1(RN). In addition, as an application we prove an existence result of positive solutions to an elliptic equation in ℝN that involves the Laplace operator with the critical Sobolev nonlinearity, or with a general nonlinear term that has a subcritical and superlinear growth. We also briefly discuss the compact embedding of
is compactly embedded into L1(RN). In addition, as an application we prove an existence result of positive solutions to an elliptic equation in ℝN that involves the Laplace operator with the critical Sobolev nonlinearity, or with a general nonlinear term that has a subcritical and superlinear growth. We also briefly discuss the compact embedding of 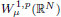 to Lp(ℝN) when N ⩾ 2 and 2 ⩽ p ⩽ N.
to Lp(ℝN) when N ⩾ 2 and 2 ⩽ p ⩽ N.
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 146 , Issue 4 , August 2016 , pp. 693 - 721
- Copyright
- Copyright © Royal Society of Edinburgh 2016
- 6
- Cited by


