Hostname: page-component-6bf8c574d5-2jptb Total loading time: 0 Render date: 2025-02-25T18:47:17.762Z Has data issue: false hasContentIssue false
  • English
  • Français

Weighted estimates for Bochner–Riesz operators on Lorentz spaces

Part of: Harmonic analysis in several variables Linear function spaces and their duals

Published online by Cambridge University Press:  28 February 2022

Sergi Baena-Miret Open the ORCID record for Sergi Baena-Miret [Opens in a new window]  and
María J. Carro Open the ORCID record for María J. Carro [Opens in a new window]
Sergi Baena-Miret
Affiliation:
Departament de Matemàtiques i Informàtica, Universitat de Barcelona, 08007 Barcelona, Spain ([email protected])
María J. Carro
Affiliation:
Departamento de Análisis Matemático y Matemática Aplicada, Universidad Complutense de Madrid, Madrid, Spain ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

We present new estimates in the setting of weighted Lorentz spaces of operators satisfying a limited Rubio de Francia condition; namely $T$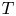 is bounded on $L^{p}(v)$ 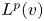for every $v$ 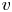in an strictly smaller class of weights than the Muckenhoupt class $A_p$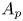. Important examples will be the Bochner–Riesz operators $BR_\lambda$ 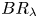with $0<\lambda <{(n-1)}/2$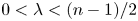, sparse operators, Hörmander multipliers with a limited regularity condition and rough operators with $\Omega \in L^{r}(\Sigma )$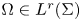, $1 < r < \infty$.

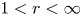Keywords

Bochner–Riesz operatorsweighted classical Lorentz spacesMuckenhoupt weightsrestricted weak type extrapolationHörmander multipliersrough operators

MSC classification

Primary: 42B99: None of the above, but in this section
Secondary: 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.)
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 153 , Issue 2 , April 2023 , pp. 654 - 678
Check for updates
Copyright
Copyright © The Author(s), 2022. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Agora, E., Carro, M. J. and Soria, J.. Boundedness of the Hilbert transform on weighted Lorentz spaces. J. Math. Anal. Appl. 395 (2012), 218–229.CrossRefGoogle Scholar
Andersen, K. F.. Weighted generalized Hardy inequalities for nonincreasing functions. Can. J. Math. 43 (1991), 1121–1135.CrossRefGoogle Scholar
Ariño, M. A. and Muckenhoupt, B.. Maximal functions on classical Lorentz spaces and Hardy's inequality with weights for nonincreasing functions. Trans. Am. Math. Soc. 320 (1990), 727–735.Google Scholar
Baena-Miret, S. and Carro, M. J.. Boundedness of sparse and rough operators on weighted Lorentz spaces. J. Fourier Anal. Appl. 27 (2021), 1–22.CrossRefGoogle Scholar
Bennett, C. and Sharpley, R. C., Interpolation of operators, Pure and Applied Mathematics, Vol. 129 (Academic Press, Inc., Boston, MA, 1988).Google Scholar
Bochner, S.. Summation of multiple Fourier series by spherical means. Trans. Am. Math. Soc. 40 (1936), 175–207.CrossRefGoogle Scholar
Bourgain, J. and Guth, L.. Bounds on oscillatory integral operators based on multilinear estimates. Geom. Funct. Anal. 21 (2011), 1239–1295.CrossRefGoogle Scholar
Carleson, L. and Sjölin, P.. Oscillatory integrals and a multiplier problem for the disc. Stud. Math. 44 (1972), 287–299.CrossRefGoogle Scholar
Carro, M. J., Duoandikoetxea, J. and Lorente, M.. Weighted estimates in a limited range with applications to the Bochner–Riesz operators. Indiana Univ. Math. J. 61 (2012), 1485–1511.CrossRefGoogle Scholar
Carro, M. J., García del Amo, A. and Soria, J.. Weak-type weights and normable Lorentz spaces. Proc. Am. Math. Soc. 124 (1996), 849–857.CrossRefGoogle Scholar
Carro, M. J., Grafakos, L. and Soria, J.. Weighted weak-type $(1,\, 1)$ estimates via Rubio de Francia extrapolation. J. Funct. Anal. 269 (2015), 1203–1233.CrossRefGoogle Scholar
Carro, M. J., Raposo, J. A. and Soria, J.. Recent developments in the theory of Lorentz spaces and weighted inequalities. Mem. Am. Math. Soc. 187 (2007), xii+128.Google Scholar
Carro, M. J. and Soria, J.. Boundedness of some integral operators. Can. J. Math. 45 (1993), 1155–1166.CrossRefGoogle Scholar
Christ, M.. On almost everywhere convergence of Bochner–Riesz means in higher dimensions. Proc. Am. Math. Soc. 95 (1985), 16–20.CrossRefGoogle Scholar
Christ, M.. Weak type endpoint bounds for Bochner–Riesz multipliers. Rev. Mat. Iberoamericana 3 (1987), 25–31.CrossRefGoogle Scholar
Christ, M.. Weak type $(1,\, 1)$ bounds for rough operators. Ann. Math. (2) 128 (1988), 19–42.CrossRefGoogle Scholar
Duoandikoetxea, J.. Weighted norm inequalities for homogeneous singular integrals. Trans. Am. Math. Soc. 336 (1993), 869–880.CrossRefGoogle Scholar
Duoandikoetxea, J., ‘Fourier analysis. Translated and revised from the 1995 Spanish original by David Cruz-Uribe’, Graduate Studies in Mathematics, Vol. 29 (American Mathematical Society, Providence, RI, 2001), xviii+222 pp.CrossRefGoogle Scholar
Duoandikoetxea, J.. Extrapolation of weights revisited: New proofs and sharp bounds. J. Funct. Anal. 260 (2011), 1886–1901.CrossRefGoogle Scholar
Fefferman, C.. The multiplier problem for the ball. Ann. Math. (2) 94 (1971), 330–336.CrossRefGoogle Scholar
Fefferman, C. and Stein, E. M.. Some maximal inequalities. Am. J. Math. 93 (1971), 107–115.Google Scholar
Grafakos, L., Modern Fourier Analysis. Third edition, Graduate Texts in Mathematics, Vol. 250, Springer, New York, 2014), xvi+624.CrossRefGoogle Scholar
Guo, S., Oh, C., Wang, H., Wu, S. and Zhang, R., The Bochner–Riesz problem: an old approach revisited, preprint, arXiv:2104.11188 (2021).Google Scholar
Guth, L., Hickman, J. and Iliopoulou, M.. Sharp estimates for oscillatory integral operators via polynomial partitioning. Acta Math. 223 (2019), 251–376.CrossRefGoogle Scholar
Hytönen, T. P.. The sharp weighted bound for general Calderón–Zygmund operators. Ann. Math. (2) 175 (2012), 1473–1506.CrossRefGoogle Scholar
Johnson, R. and Neugebauer, C. J.. Change of variable results for $A_p$- and reverse Hölder $RH_r$-classes. Trans. Am. Math. Soc. 328 (1991), 639–666.Google Scholar
Kesler, R. and Lacey, M. T.. Sparse endpoint estimates for Bochner–Riesz multipliers on the plane. Collect. Math. 69 (2018), 427–435.CrossRefGoogle Scholar
Kurtz, D. S. and Wheeden, R. L.. Results on weighted norm inequalities for multipliers. Trans. Am. Math. Soc. 255 (1979), 343–362.CrossRefGoogle Scholar
Lacey, M. T., Mena, D. and Reguera, M. C.. Sparse bounds for Bochner–Riesz multipliers. J. Fourier Anal. Appl. 25 (2019), 523–537.CrossRefGoogle Scholar
Lai, S.. Weighted norm inequalities for general operators on monotone functions. Trans. Am. Math. Soc. 340 (1993), 811–836.CrossRefGoogle Scholar
Lerner, A. K.. A simple proof of the $A_2$ conjecture. Int. Math. Res. Not. IMRN 14 (2013), 3159–3170.CrossRefGoogle Scholar
Lerner, A. K.. On an estimate of Calderón–Zygmund operators by dyadic positive operators. J. Anal. Math. 121 (2013), 141–161.CrossRefGoogle Scholar
Martín, J. and Milman, M.. Weighted norm inequalities and indices. J. Funct. Spaces Appl. 4 (2006), 43–71.CrossRefGoogle Scholar
Muckenhoupt, B.. Weighted norm inequalities for the Hardy maximal function. Trans. Am. Math. Soc. 165 (1972), 207–226.CrossRefGoogle Scholar
Neugebauer, C. J.. Weighted norm inequalities for averaging operators of monotone functions. Publ. Mat. 35 (1991), 429–447.CrossRefGoogle Scholar
Neugebauer, C. J.. Some classical operators on Lorentz space. Forum Math. 4 (1992), 135–146.CrossRefGoogle Scholar
Seeger, A.. Endpoint inequalities for Bochner–Riesz multipliers in the plane. Pac. J. Math. 174 (1996), 543–553.CrossRefGoogle Scholar
Shi, X. L. and Sun, Q. Y.. Weighted norm inequalities for Bochner–Riesz operators and singular integral operators. Proc. Am. Soc. 116 (1992), 665–673.CrossRefGoogle Scholar
Soria, J.. Lorentz spaces of weak-type. Quart. J. Math. Oxford Ser. (2) 49 (1998), 93–103.CrossRefGoogle Scholar
Tao, T.. Weak-type endpoint bounds for Riesz means. Proc. Am. Math. Soc. 124 (1996), 2797–2805.CrossRefGoogle Scholar
Tao, T.. The weak-type endpoint Bochner–Riesz conjecture and related topics. Indiana Univ. Math. J. 47 (1998), 1097–1124.CrossRefGoogle Scholar
Vargas, A. M.. Weighted weak type $(1,\, 1)$ bounds for rough operators. J. London Math. Soc. (2) 54 (1996), 297–310.CrossRefGoogle Scholar
Wu, S., On the Bochner–Riesz operator in $\mathbb {R}^{3}$, preprint, arXiv:2008.13043 (2020).Google Scholar