Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-12-01T03:16:50.749Z Has data issue: false hasContentIssue false
  • English
  • Français

The regularity and stability of solutions to semilinear fourth-order elliptic problems with negative exponents

Part of: Partial differential equations Elliptic equations and systems

Published online by Cambridge University Press:  07 January 2016

Baishun Lai
Baishun Lai*
Affiliation:
Institute of Contemporary Mathematics, Henan University, Kaifeng 475004, People's Republic of China ([email protected])
Get access Rights & Permissions [Opens in a new window]

Extract

We examine the regularity of the extremal solution of the nonlinear eigenvalue problem

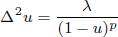
on a general bounded domain Ω in ℝN, with Navier boundary condition u = Δu on ∂Ω. Firstly, we prove the extremal solution is smooth for any p > 1 and N ⩽ 4, which improves the result of Guo and Wei (Discrete Contin. Dynam. Syst. A 34 (2014), 2561–2580). Secondly, if p = 3, N = 3, we prove that any radial weak solution of this nonlinear eigenvalue problem is smooth in the case Ω = 𝔹, which completes the result of Dávila et al. (Math. Annalen348 (2009), 143–193). Finally, we also consider the stability of the entire solution of Δ2u = –l/up in ℝN with u > 0.

Keywords

biharmonic equationentire solutionregularitystability

MSC classification

Secondary: 35J91: Semilinear elliptic equations with Laplacian, bi-Laplacian or poly-Laplacian 35B08: Entire solutions 35B53: Liouville theorems, Phragmén-Lindelöf theorems 35B40: Asymptotic behavior of solutions
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 146 , Issue 1 , February 2016 , pp. 195 - 212
Copyright
Copyright © Royal Society of Edinburgh 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)