Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Schemm, Sabine
2011.
Partial regularity of minimizers of higher order integrals with (p,q)-growth.
ESAIM: Control, Optimisation and Calculus of Variations,
Vol. 17,
Issue. 2,
p.
472.
Campos Cordero, Judith
2022.
Partial Regularity for Local Minimizers of Variational Integrals With Lower-Order Terms.
The Quarterly Journal of Mathematics,
Vol. 73,
Issue. 3,
p.
805.
Gmeineder, Franz
and
Kristensen, Jan
2024.
Quasiconvex Functionals of (p, q)-Growth and the Partial Regularity of Relaxed Minimizers.
Archive for Rational Mechanics and Analysis,
Vol. 248,
Issue. 5,
Irving, Christopher
2025.
Partial regularity for minima of higher-order quasiconvex integrands with natural Orlicz growth.
Annali di Matematica Pura ed Applicata (1923 -),


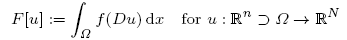
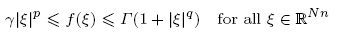
 .
.