Hostname: page-component-586b7cd67f-t7fkt Total loading time: 0 Render date: 2024-11-30T23:08:36.177Z Has data issue: false hasContentIssue false
  • English
  • Français

On the loss of compactness in the vectorial heteroclinic connection problem

Part of: Normed linear spaces and Banach spaces; Banach lattices Qualitative theory Infinite-dimensional Hamiltonian systems Equilibrium statistical mechanics

Published online by Cambridge University Press:  22 March 2016

Nikos Katzourakis
Nikos Katzourakis*
Affiliation:
Department of Mathematics and Statistics, University of Reading, Whiteknights Campus, PO Box 220, Reading RG6 6AX, UK ([email protected])
Get access Rights & Permissions [Opens in a new window]

Extract

We give an alternative proof of the theorem of Alikakos and Fusco concerning existence of heteroclinic solutions U : ℝ → ℝN to the system

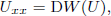
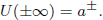
Here a± are local minima of a potential W ∈ C2(ℝN) with W(a±) = 0. This system arises in the theory of phase transitions. Our method is variational but differs from the original artificial constraint method of Alikakos and Fusco and establishes existence by analysing the loss of compactness in minimizing sequences of the action in the appropriate functional space. Our assumptions are slightly different from those considered previously and also imply a priori estimates for the solution.

Keywords

Heteroclinic connection problemloss of compactnessphase transitionsHamiltonian system

MSC classification

Secondary: 34C37: Homoclinic and heteroclinic solutions 46B50: Compactness in Banach (or normed) spaces 82B26: Phase transitions (general) 37K05: Hamiltonian structures, symmetries, variational principles, conservation laws
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 146 , Issue 3 , June 2016 , pp. 595 - 608
Copyright
Copyright © Royal Society of Edinburgh 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)