Numerical invariants on twisted noncommutative polyballs: curvature and multiplicity

Abstract

The goal of this paper is to show that the theory of curvature invariant, as introduced by Arveson, admits a natural extension to the framework of 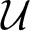${\mathcal U}$-twisted polyballs 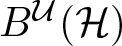$B^{\mathcal U}({\mathcal H})$ which consist of k-tuples $(A_1,\ldots, A_k)$ of row contractions 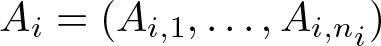$A_i=(A_{i,1},\ldots, A_{i,n_i})$ satisfying certain ${\mathcal U}$-commutation relations with respect to a set 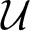${\mathcal U}$ of unitary commuting operators on a Hilbert space 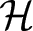${\mathcal H}$. Throughout this paper, we will be concerned with the curvature of the elements 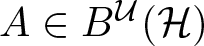$A\in B^{\mathcal U}({\mathcal H})$ with positive trace class defect operator 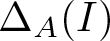$\Delta_A(I)$. We prove the existence of the curvature invariant and present some of its basic properties. A distinguished role as a universal model among the pure elements in 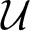${\mathcal U}$-twisted polyballs is played by the standard $I\otimes{\mathcal U}$-twisted multi-shift S acting on 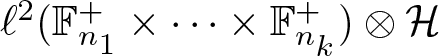$\ell^2({\mathbb F}_{n_1}^+\times\cdots\times {\mathbb F}_{n_k}^+)\otimes {\mathcal H}$. The curvature invariant $\mathrm{curv} (A)$ can be any non-negative real number and measures the amount by which A deviates from the universal model S. Special attention is given to the $I\otimes {\mathcal U}$-twisted multi-shift S and the invariant subspaces (co-invariant) under S and $I\otimes {\mathcal U}$, due to the fact that any pure element 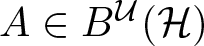$A\in B^{\mathcal U}({\mathcal H})$ with 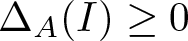$\Delta_A(I)\geq 0$ is the compression of S to such a co-invariant subspace.