Article contents
Morse index and symmetry for elliptic problems with nonlinear mixed boundary conditions
Published online by Cambridge University Press: 27 December 2018
Abstract
We consider an elliptic problem of the type
 $$\left\{ {\matrix{ {-\Delta u = f(x,u)\quad } \hfill & {{\rm in}\,\Omega } \hfill \cr {u = 0} \hfill & {{\rm on}\,\Gamma _1} \hfill \cr {\displaystyle{{\partial u} \over {\partial \nu }} = g(x,u)} \hfill & {{\rm on}\,\Gamma _2} \hfill \cr } } \right.$$
$$\left\{ {\matrix{ {-\Delta u = f(x,u)\quad } \hfill & {{\rm in}\,\Omega } \hfill \cr {u = 0} \hfill & {{\rm on}\,\Gamma _1} \hfill \cr {\displaystyle{{\partial u} \over {\partial \nu }} = g(x,u)} \hfill & {{\rm on}\,\Gamma _2} \hfill \cr } } \right.$$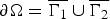 $\partial \Omega = \overline {\Gamma _1} \cup \overline {\Gamma _2} $.
$\partial \Omega = \overline {\Gamma _1} \cup \overline {\Gamma _2} $.
Under a Morse index condition, we prove cylindrical symmetry results for solutions of the above problem.
As an intermediate step, we relate the Morse index of a solution of the nonlinear problem to the eigenvalues of the following linear eigenvalue problem
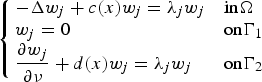 $$\left\{ {\matrix{ {-\Delta w_j + c(x)w_j = \lambda _jw_j} \hfill & {{\rm in }\Omega } \hfill \cr {w_j = 0} \hfill & {{\rm on }\Gamma _1} \hfill \cr {\displaystyle{{\partial w_j} \over {\partial \nu }} + d(x)w_j = \lambda _jw_j} \hfill & {{\rm on }\Gamma _2} \hfill \cr } } \right.$$
$$\left\{ {\matrix{ {-\Delta w_j + c(x)w_j = \lambda _jw_j} \hfill & {{\rm in }\Omega } \hfill \cr {w_j = 0} \hfill & {{\rm on }\Gamma _1} \hfill \cr {\displaystyle{{\partial w_j} \over {\partial \nu }} + d(x)w_j = \lambda _jw_j} \hfill & {{\rm on }\Gamma _2} \hfill \cr } } \right.$$
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 149 , Issue 2 , April 2019 , pp. 305 - 324
- Copyright
- Copyright © Royal Society of Edinburgh 2018
References
- 3
- Cited by


