Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-25T18:27:58.533Z Has data issue: false hasContentIssue false
  • English
  • Français

Lp-Theory of degenerate-elliptic and parabolic operators of second order

Published online by Cambridge University Press:  14 November 2011

Baoswan Wong-Dzung
Baoswan Wong-Dzung
Affiliation:
Department of Mathematics, University of California, Berkeley, CA 94720, U.S.A
Get access Rights & Permissions [Opens in a new window]

Synopsis

We consider the formal operator given by

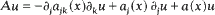
in the Banach space X = LP(Rn), 1<p<∞. The coefficients ajk(x), aj(x), and a(x) are real-valued functions, ajk ε C2(Rn) has bounded second derivatives, aj ε Cl(Rn) has bounded first derivatives, and aεL∞(Rn). Furthermore, we assume that the n × n matrix (ajk(x)) is symmetric and positive semidefinite (i.e. ajk(x)ξjξk≧0 for all (ξ1,…,ξn)ε Rn and x ε Rn). We prove that the degenerate-elliptic differential operator given by –A and restricted to , the minimal realization of –A, is essentially quasi-m-dispersive in Lp(Rn), (hence that the minimal realization of +A is quasi-m-accretive) and that its closure coincides with the maximal realization of –A.

Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 95 , Issue 1-2 , 1983 , pp. 95 - 113
Copyright
Copyright © Royal Society of Edinburgh 1983

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1Arendt, W., Chernoff, P. R. and Kato, T.. A generalization of dissipativity and positive semigroups. J. Operator Theory 8 (1982), 167–180.Google Scholar
2Devinatz, A.. Selfadjointness of second order elliptic and degenerate elliptic differential operators. Proc. Intemat. Conf. on Differential Equations, Uppsala, Sweden pp. 37–51. (Acta Universitatis Upsaliensis, 1977).Google Scholar
3Devinatz, A.. Selfadjointness of second order degenerate-elliptic operators. Indiana Univ. Math. J. 27 (1978), 255–266.CrossRefGoogle Scholar
4Devinatz, A.. On an inequality of Tosio Kato for degenerate-elliptic operators. J. Functional Anal. 32 (1979), 312–335.CrossRefGoogle Scholar
5Fichera, G.. Sulle equazioni differenziali lineari ellitico-paraboliche del secondo ordine. Atti Accad. Naz. Lincei Mem. Cl. Sci. Fis. Mat. Nat. Sez. I 5 (1956), 1–30.Google Scholar
6Fichera, G.. On a unified theory of boundary value problems for elliptic-parabolic equations of second order. In Boundary Problems in Differential Equations, pp. 97–120 (Univ. of Wisconsin Press, 1960).Google Scholar
7Frehse, J.. Essential selfadjointness of singular elliptic operators. Bol. Soc. Brasil. Mat. 8 (1977), 87–107.CrossRefGoogle Scholar
8Freidlin, M. I.. On the factorization of non-negative definite matrices. Theory Probab. Appl. 13 (1968), 354–356.CrossRefGoogle Scholar
9Friedrichs, K. O.. The identity of weak and strong extensions of differential operators. Trans. Amer. Math. Soc. 55 (1944), 132–151.CrossRefGoogle Scholar
10Kato, T.. Remarks on pseudo-resolvents and infinitesimal generators of semigroups. Proc. Japan Acad. 35 (1959), 467–468.Google Scholar
11Kato, T.. Linear evolution equations of ‘hyperbolic’ type. J. Fac. Sci. Univ. Tokyo Sect. I A Math. 17 (1970), 241–258.Google Scholar
12Kato, T.. Schrödinger operators with singular potentials. Israel J. Math. 13 (1972), 135–148.CrossRefGoogle Scholar
13 J. Moser. A new proof of de Giorgi's theorem concerning the regularity problem for elliptic differential equations. Comm. Pure Appl. Math. 13 (1960), 457–468.CrossRefGoogle Scholar
14Oleinik, O. A.. Linear equations of second order with non-negative characteristic form. Mat. Sb. 69 (111) (1966), 111–140; Amer. Math. Soc. Transl. Ser. 2 65 (1967), 167–199.Google Scholar
15Phillips, R. S.. Semigroups of positive contraction operators. Czechoslovak Math. J. 12(87) (1962), 294–313.CrossRefGoogle Scholar
16Stampacchia, G.. Equations elliptiques du second ordre à coefficients discontinus (Montréal: Les Presses de l'Université de Montréal, 1966).Google Scholar
17Stroock, D. W. and Varadhan, S. R. S.. Multidimensional diffusion processes. Grundlehren der math. Wiss. 233 (Berlin: Springer, 1979).Google Scholar