Article contents
Analytic continuation and boundary continuity of functions of several complex variables
Published online by Cambridge University Press: 14 November 2011
Synopsis
This note treats some questions about analytic continuation in several variables. The first theorem in effect determines the envelops of holomorphy of certain domains in ℂn. The second main result is a continuity theorem: If a bounded holomorphic function f on a convex domain ∆ in ℂn has boundary values that are continuous on the complement (in b∆) of a set of the form int∏ (b∆∩∏) where ∏ is a real hyperplane in ℂn that misses ∆, then f is continuous on 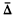 . In addition, we obtain what may be regarded as a local version of the theorem in our earlier paper concerning the one-dimensional extension property. Our methods depend on Hartogs' theorem (n ≧ 3) and directly on the BochnerMartinelli formula (n = 2).
. In addition, we obtain what may be regarded as a local version of the theorem in our earlier paper concerning the one-dimensional extension property. Our methods depend on Hartogs' theorem (n ≧ 3) and directly on the BochnerMartinelli formula (n = 2).
- Type
- Research Article
- Information
- Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 89 , Issue 1-2 , 1981 , pp. 63 - 74
- Copyright
- Copyright © Royal Society of Edinburgh 1981
References
- 12
- Cited by


