No CrossRef data available.
Article contents
Solar Surface Anisotropy effect on the Magnetic Field
Published online by Cambridge University Press: 24 July 2015
Abstract
Within the literature there are at least 15 references indicating that the horizontal magnetic flux does not exactly balance vertical flux in sunspots, leading to the surprising result that div 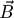 $\vec{B}$ would depart from zero. Intuitively, this has to be related to the stratification at the surface of the star, due to which horizontal and vertical typical lengths are different. This surface anisotropy results from gravity, but how does gravity influence the magnetic field? To answer this question, a scenario has been proposed in two recent publications, based on anisotropic Debye shielding. The presentation reported in this paper was devoted to investigate the possibility and causes of a non-zero div
$\vec{B}$ would depart from zero. Intuitively, this has to be related to the stratification at the surface of the star, due to which horizontal and vertical typical lengths are different. This surface anisotropy results from gravity, but how does gravity influence the magnetic field? To answer this question, a scenario has been proposed in two recent publications, based on anisotropic Debye shielding. The presentation reported in this paper was devoted to investigate the possibility and causes of a non-zero div 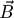 $\vec{B}$. A scaling law associated with the anisotropy is able to reestablish the nullity of div
$\vec{B}$. A scaling law associated with the anisotropy is able to reestablish the nullity of div 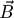 $\vec{B}$, which would lead to a renewed MHD in the solar photosphere layer. An eventual observation in the laboratory is also reported.
$\vec{B}$, which would lead to a renewed MHD in the solar photosphere layer. An eventual observation in the laboratory is also reported.
- Type
- Contributed Papers
- Information
- Proceedings of the International Astronomical Union , Volume 10 , Symposium S305: Polarimetry: From the Sun to Stars and Stellar Environments , December 2014 , pp. 28 - 34
- Copyright
- Copyright © International Astronomical Union 2015


