Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Tong, Jingcheng
1988.
Segre's theorem on asymmetric diophantine approximation.
Journal of Number Theory,
Vol. 28,
Issue. 1,
p.
116.
Tong, Jing Cheng
1989.
The conjugate property for Diophantine approximation of continued fractions.
Proceedings of the American Mathematical Society,
Vol. 105,
Issue. 3,
p.
535.
Kraaikamp, Cor
1990.
On the approximation by continued fractions, II.
Indagationes Mathematicae,
Vol. 1,
Issue. 1,
p.
63.
Tong, Jingcheng
1991.
Diophantine approximation by continued fractions.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 51,
Issue. 2,
p.
324.
Prasad, K. C.
and
Prasad, Arjun
1991.
A note on asymmetric approximation.
Mathematika,
Vol. 38,
Issue. 1,
p.
34.


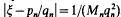 . The following two theorems were proved by Müller [
. The following two theorems were proved by Müller [