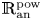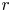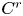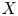Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Van Hille, Siegfried
2022.
Mild parametrizations of power-subanalytic sets.
Annals of Pure and Applied Logic,
Vol. 173,
Issue. 6,
p.
103105.