Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lyubarskii, Yurii I.
and
Seip, Kristian
1997.
Sampling and interpolating sequences for multiband-limited functions and exponential bases on disconnected sets.
The Journal of Fourier Analysis and Applications,
Vol. 3,
Issue. 5,
p.
597.
Herley, C.
and
Ping Wah Wong
1999.
Minimum rate sampling and reconstruction of signals with arbitrary frequency support.
IEEE Transactions on Information Theory,
Vol. 45,
Issue. 5,
p.
1555.
Avdonin, Sergei
and
Moran, William
1999.
Optimal Control of Partial Differential Equations.
p.
43.
Butzer, Paul L.
Higgins, J. R.
and
Stens, R. L.
2000.
Development of Mathematics, 1950–2000.
p.
193.
Christensen, Ole
and
Lindner, Alexander M.
2001.
Frames of exponentials: lower frame bounds for finite subfamilies and approximation of the inverse frame operator.
Linear Algebra and its Applications,
Vol. 323,
Issue. 1-3,
p.
117.
Avdonin, Sergei
Bulanova, Anna
and
Moran, William
2007.
Construction of Sampling and Interpolating Sequences for Multi-Band Signals. The Two-Band Case.
International Journal of Applied Mathematics and Computer Science,
Vol. 17,
Issue. 2,
p.
143.
Kozma, Gady
and
Lev, Nir
2011.
Exponential Riesz Bases, Discrepancy of Irrational Rotations and BMO.
Journal of Fourier Analysis and Applications,
Vol. 17,
Issue. 5,
p.
879.
Baranov, A.
Dumont, A.
Hartmann, A.
and
Kellay, K.
2015.
Sampling, interpolation and Riesz bases in small Fock spaces.
Journal de Mathématiques Pures et Appliquées,
Vol. 103,
Issue. 6,
p.
1358.
Benedetto, John J.
Nava-Tudela, Alfredo
Powell, Alexander M.
and
Wang, Yang
2017.
Frames and Other Bases in Abstract and Function Spaces.
p.
185.
Pfander, Gotz E.
Revay, Shauna
and
Walnut, David
2019.
Riesz bases of exponentials for partitions of intervals.
p.
1.
Debernardi, Alberto
and
Lev, Nir
2021.
Extended Abstracts Fall 2019.
Vol. 12,
Issue. ,
p.
69.
Baranov, Anton
Belov, Yurii
and
Gröchenig, Karlheinz
2022.
Complete interpolating sequences for the Gaussian shift-invariant space.
Applied and Computational Harmonic Analysis,
Vol. 61,
Issue. ,
p.
191.
Caragea, Andrei
and
Lee, Dae Gwan
2022.
A note on exponential Riesz bases.
Sampling Theory, Signal Processing, and Data Analysis,
Vol. 20,
Issue. 2,
Lee, Dae Gwan
Pfander, Götz E.
and
Walnut, David
2023.
Bases of complex exponentials with restricted supports.
Journal of Mathematical Analysis and Applications,
Vol. 521,
Issue. 2,
p.
126917.
Baranov, Anton
Belov, Yurii
and
Kuznetsov, Alexander
2023.
Systems Biorthogonal to Exponential Systems on a Finite Union of Intervals.
Journal of Fourier Analysis and Applications,
Vol. 29,
Issue. 2,
Belov, Yurii
and
Mironov, Mikhail
2024.
Exponential Riesz Bases in L2 on Two Intervals.
International Mathematics Research Notices,
Vol. 2024,
Issue. 7,
p.
5403.
Lee, Dae Gwan
2024.
Unions of exponential Riesz bases.
AIMS Mathematics,
Vol. 9,
Issue. 9,
p.
23890.
Lee, Dae Gwan
Pfander, Götz E.
and
Walnut, David
2024.
Cube Tilings with Linear Constraints.
Results in Mathematics,
Vol. 79,
Issue. 5,
Pfander, Götz
Revay, Shauna
and
Walnut, David
2024.
Exponential bases for partitions of intervals.
Applied and Computational Harmonic Analysis,
Vol. 68,
Issue. ,
p.
101607.
Lee, Dae Gwan
Pfander, Götz E.
and
Walnut, David
2024.
Exponential Bases for Parallelepipeds with Frequencies Lying in a Prescribed Lattice.
Results in Mathematics,
Vol. 79,
Issue. 6,


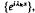 , {λ
, {λ