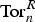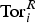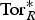Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Nakamura, Tsutomu
and
Thompson, Peder
2020.
Minimal semi-flat-cotorsion replacements and cosupport.
Journal of Algebra,
Vol. 562,
Issue. ,
p.
587.
Dailey, Douglas J.
Iyengar, Srikanth B.
and
Marley, Thomas
2020.
Detecting finite flat dimension of modules via iterates of the Frobenius endomorphism.
Journal of Commutative Algebra,
Vol. 12,
Issue. 1,
Christensen, Lars Winther
Ferraro, Luigi
and
Thompson, Peder
2023.
Rigidity of Ext and Tor via flat–cotorsion theory.
Proceedings of the Edinburgh Mathematical Society,
Vol. 66,
Issue. 4,
p.
1142.
Hai, Phùng Hô
Nguyen, Hop D.
and
dos Santos, João Pedro
2025.
Fiber criteria for flatness and homomorphisms of flat affine group schemes.
Journal of Pure and Applied Algebra,
Vol. 229,
Issue. 6,
p.
107949.