Hostname: page-component-745bb68f8f-mzp66 Total loading time: 0 Render date: 2025-02-07T10:51:16.532Z Has data issue: false hasContentIssue false
  • English
  • Français

Reversibility of affine transformations

Part of: Complex spaces with a group of automorphisms Special matrices Automorphic functions Structure and classification of infinite or finite groups Classical differential geometry

Published online by Cambridge University Press:  08 November 2023

Krishnendu Gongopadhyay Open the ORCID record for Krishnendu Gongopadhyay [Opens in a new window] ,
Tejbir Lohan Open the ORCID record for Tejbir Lohan [Opens in a new window]  and
Chandan Maity Open the ORCID record for Chandan Maity [Opens in a new window]
Krishnendu Gongopadhyay
Affiliation:
Department of Mathematical Sciences, Indian Institute of Science Education and Research (IISER) Mohali, Knowledge City, Punjab, India ([email protected]; [email protected]; [email protected])
Tejbir Lohan
Affiliation:
Department of Mathematical Sciences, Indian Institute of Science Education and Research (IISER) Mohali, Knowledge City, Punjab, India ([email protected]; [email protected]; [email protected])
Chandan Maity
Affiliation:
Department of Mathematical Sciences, Indian Institute of Science Education and Research (IISER) Mohali, Knowledge City, Punjab, India ([email protected]; [email protected]; [email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

An element g in a group G is called reversible if g is conjugate to g−1 in G. An element g in G is strongly reversible if g is conjugate to g−1 by an involution in G. The group of affine transformations of 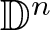$\mathbb D^n$ may be identified with the semi-direct product 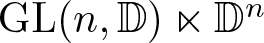$\mathrm{GL}(n, \mathbb D) \ltimes \mathbb D^n $, where 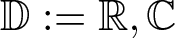$\mathbb D:=\mathbb R, \mathbb C$ or $ \mathbb H $. This paper classifies reversible and strongly reversible elements in the affine group 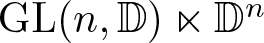$\mathrm{GL}(n, \mathbb D) \ltimes \mathbb D^n $.

Keywords

affine groupreversible elementsstrongly reversible elementsreal elementsstrongly real elementsadjoint reality

MSC classification

Primary: 53A15: Affine differential geometry
Secondary: 20E45: Conjugacy classes 32M17: Automorphism groups of $^n$ and affine manifolds 15B33: Matrices over special rings (quaternions, finite fields, etc.)
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 66 , Issue 4 , November 2023 , pp. 1217 - 1228
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on Behalf of The Edinburgh Mathematical Society.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Arnol’d, V.I. and Avez, A., Ergodic problems of classical mechanics. Translated from French, by Avez, A. and Benjamin, W. A., (Addison-Wesley, New York–Amsterdam, 1968)Google Scholar
Bhunia, S. and Gongopadhyay, K., Reversible quaternionic hyperbolic isometries, Linear Algebra Appl. 591 (2020), 268–283. doi:10.1016/j.laa.2019.12.043.CrossRefGoogle Scholar
Devaney, R. L., Reversible diffeomorphisms and flows, Trans. Amer. Math. Soc. 218 (1976), 89–113. doi:10.2307/1997429.CrossRefGoogle Scholar
Goldman, W. M., Geometric structures on manifolds, Graduate Studies in Mathematics, Volume 227, (American Mathematical Society, Providence, RI, 2022).CrossRefGoogle Scholar
Gongopadhyay, K. and Lohan, T., Reversibility of Hermitian isometries, Linear Algebra Appl. 639 (2022), 159–176. doi:10.1016/j.laa.2022.01.009.CrossRefGoogle Scholar
Gongopadhyay, K., Lohan, T. and Maity, C., Reversibility and real adjoint orbits of linear maps, in Essays in Geometry, Dedicated to Norbert A’Campo (ed. Papadopoulos, A.) (European Mathematical Society Press, Berlin, 2023). doi:10.4171/IRMA/34/15.Google Scholar
Gongopadhyay, K. and Maity, C., Reality of unipotent elements in classical Lie groups, Bull. Sci. Math. 185 (2023), . doi:10.1016/j.bulsci.2023.103261.CrossRefGoogle Scholar
Gustafson, W. H., Halmos, P. R. and Radjavi, H., Products of involutions, Linear Algebra Appl. 13(1–2) (1976), 157–162.CrossRefGoogle Scholar
Knapp, A. W., Lie groups beyond an introduction, Progress in Mathematics, 2nd edn, Volume 140, (Birkhäuser Boston, Inc., Boston, MA, 2002).Google Scholar
Lamb, J. S. W., Reversing symmetries in dynamical systems, J. Phys. A 25(4) (1992), 925–937.CrossRefGoogle Scholar
O’Farrell, A. G. and Short, I., Reversibility in dynamics and group theory, London Mathematical Society Lecture Note Series, Volume 416, (Cambridge University Press, Cambridge, 2015).CrossRefGoogle Scholar
Rodman, L., Topics in quaternion linear algebra, Princeton Series in Applied Mathematics, (Princeton University Press, Princeton, NJ, 2014).Google Scholar
Sevryuk, M. B., Reversible systems, Lecture Notes in Mathematics, Volume 1211, (Springer-Verlag, Berlin, 1986).CrossRefGoogle Scholar
Short, I., Reversible maps in isometry groups of spherical, Euclidean and hyperbolic space, Math. Proc. R. Ir. Acad. 108(1) (2008), 33–46.CrossRefGoogle Scholar
Wonenburger, M. J., Transformations which are products of two involutions, J. Math. Mech 16 (4) (1966), 327–338.Google Scholar