Article contents
Representations of normed algebras with minimal left ideals
Published online by Cambridge University Press: 20 January 2009
Extract
The theory of *-representations of Banach *-algebras on Hilbert space is one of the most useful and most successful parts of the theory of Banach algebras. However, there are only scattered results concerning the representations of general Banach algebras on Banach spaces. It may be that a comprehensive representation theory is impossible. Nevertheless, for some special algebras interesting and worthwhile results can be proved. This is true for  (Y), the algebra of all bounded operators on a Banach space Y, and for
(Y), the algebra of all bounded operators on a Banach space Y, and for 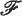 (Y), the subalgebra of
(Y), the subalgebra of  (Y) consisting of operators with finite dimensional range. The representations of
(Y) consisting of operators with finite dimensional range. The representations of  (Y) are studied in a recent paper by H. Porta and E. Berkson (6), and in another recent paper (8), P. Chernoff determines the structure of the representations of
(Y) are studied in a recent paper by H. Porta and E. Berkson (6), and in another recent paper (8), P. Chernoff determines the structure of the representations of 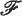 (Y) (and also of some more general algebras of operators). In both these papers,
(Y) (and also of some more general algebras of operators). In both these papers, 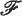 (Y), which is the socle of the algebras under consideration, plays an important role in the theory. This suggests the possibility that a more general representation theory can be formulated in the case of a normed algebra with a nontrivial socle. This we attempt to do in this paper.
(Y), which is the socle of the algebras under consideration, plays an important role in the theory. This suggests the possibility that a more general representation theory can be formulated in the case of a normed algebra with a nontrivial socle. This we attempt to do in this paper.
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 19 , Issue 2 , September 1974 , pp. 173 - 190
- Copyright
- Copyright © Edinburgh Mathematical Society 1974
References
REFERENCES
- 1
- Cited by


