Article contents
A Pair of Dual Integral Equations Involving Bessel Functions of the First and the Second Kind
Published online by Cambridge University Press: 20 January 2009
Extract
In this paper we give a method for the solution of the dual integral equations
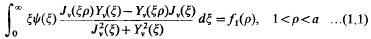
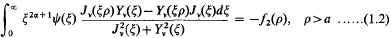
where Jv and Yv are Bessel functions of the first and second kind, −½≦α≦½, f1(ρ) and f2(ρ) are known functions and ψ(ξ) is to be determined. Such equations arise in the discussion of boundary value problems for half-spaces containing a cylindrical cavity. For example, let us take the problem of finding a potential function φ(ρ, θ, z) which satisfies Laplace's equation for

subject to the usual regularity conditions and the following boundary conditions:

- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 14 , Issue 2 , December 1964 , pp. 149 - 158
- Copyright
- Copyright © Edinburgh Mathematical Society 1964
References
REFERENCES
- 3
- Cited by


