Article contents
On the “elementary” solution of Laplace's Equation
Published online by Cambridge University Press: 20 January 2009
Extract
Hadamard defines the “elementary solution” of the general linear partial differential equation of the second order, namely
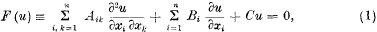
(Aik, BiC being functions of the n variables x1, x2, .., xn, which may be regarded as coordinates in a space of n dimensions), to be one of those solutions which are infinite to as low an order as possible at a given point and on every bicharacteristic through that point.
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 2 , Issue 3 , January 1931 , pp. 135 - 139
- Copyright
- Copyright © Edinburgh Mathematical Society 1931
References
page 135 note 1Lectures on Gauchy's Problem in Linear Partial Differential Equations (Yale, 1923), p. 70, et seq.Google Scholar
page 135 note 2The solutions satisfying this condition differ only in the values of arbitrary constants, the elementary solution being obtained by choosing these according to a certain rule. For the purpose of this paper it suffices to say that the elementary solution is the one which reduces to  , where
, where  , when the differential equation (1) is of the particular form
, when the differential equation (1) is of the particular form 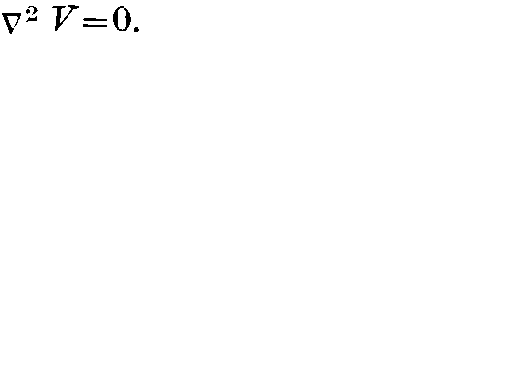 .Google Scholar
.Google Scholar
page 136 note 1The summation convention is adopted throughout. The notation of the succeeding paragraphs will differ to some extent from that of Hadamard, in order that it should be brought into conformity with the notation now usual in the Tensor Calculus.Google Scholar
page 136 note 2 Thus if in § 1 (qi) is taken at 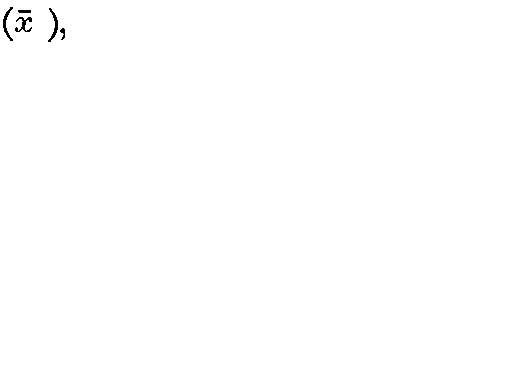 , we have
, we have 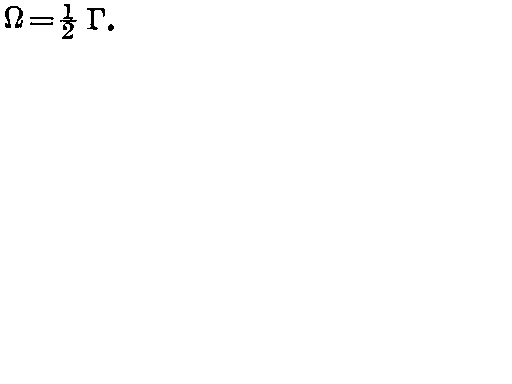 .
.
page 137 note 1The y i are the normal variables of Lipschitz :Google ScholarHadamard, , loc. cit., p. 87.Google Scholar A full account of them is given by Veblen, , Invariants of Quadratic Differential Forms (Cambridge Tract no. 24, 1927), ch. VI.Google Scholar
page 137 note 2Veblen, , loc. cit., ch. VI (14.8).Google Scholar
page 138 note 1This equation is essentially the same as Hadamard's, loc. cit., p. 91 (37).Google Scholar
page 138 note 2In a paper by the author to appear shortly in Proc. London Math. Soc.Google Scholar
- 7
- Cited by


