No CrossRef data available.
Article contents
On the Dvoretzky-Rogers theorem
Published online by Cambridge University Press: 20 January 2009
Extract
The classical Dvoretzky-Rogers theorem states that if E is a normed space for which l1(E)=l1{E} (or equivalently 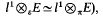 , then E is finite dimensional (see [12] p. 67). This property still holds for any lp (l<p<∞) in place of l1 (see [7]p. 104 and [2] Corollary 5.5). Recently it has been shown that this result remains true when one replaces l1 by any non nuclear perfect sequence space having the normal topology (see [14]). In this context, De Grande-De Kimpe [4] gives an extension of the Devoretzky-Rogers theorem for perfect Banach sequence spaces.
, then E is finite dimensional (see [12] p. 67). This property still holds for any lp (l<p<∞) in place of l1 (see [7]p. 104 and [2] Corollary 5.5). Recently it has been shown that this result remains true when one replaces l1 by any non nuclear perfect sequence space having the normal topology (see [14]). In this context, De Grande-De Kimpe [4] gives an extension of the Devoretzky-Rogers theorem for perfect Banach sequence spaces.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1984


