Article contents
On joins with group congruences
Published online by Cambridge University Press: 20 January 2009
Abstract
Let  be an arbitrary semigroup. A congruence γ on
be an arbitrary semigroup. A congruence γ on  is a group congruence if
is a group congruence if  /γ is a group. The set of group congruences on
/γ is a group. The set of group congruences on  is non-empty since
is non-empty since  ×
×  is a group congruence. The lattice of congruences on a semigroup
is a group congruence. The lattice of congruences on a semigroup  will be denoted by
will be denoted by 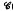 (
( ) and the set of group congruences on
) and the set of group congruences on  will be denoted by
will be denoted by 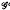
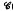 (
( ). If
). If 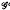
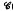 (
( ) is a lattice then it is modular and γ ∨ ρ = γ ο ρ = ρ ο γ for all γ, ρ ε
) is a lattice then it is modular and γ ∨ ρ = γ ο ρ = ρ ο γ for all γ, ρ ε 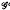
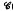 (
( ). The main result is that γ ν ρ = γ ο ρ ο γ for any γ ε
). The main result is that γ ν ρ = γ ο ρ ο γ for any γ ε 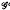
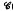 (
( ) and ρ ε
) and ρ ε 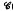 (
( ) (whence every element of the set
) (whence every element of the set 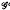
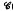 (
( ) is dually right modular in
) is dually right modular in 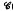 (
( ). This result has appeared, for particular classes of semigroups, many times in the literature. Also γ ν ρ = γ ο ρ ο γ = ρ ο γ ο ρ for all γ, ρ ε
). This result has appeared, for particular classes of semigroups, many times in the literature. Also γ ν ρ = γ ο ρ ο γ = ρ ο γ ο ρ for all γ, ρ ε 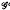
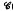 (
( ) which is similar to the well known result for the join of congruences on a group. Furthermore, if γ ∩ ρ ε
) which is similar to the well known result for the join of congruences on a group. Furthermore, if γ ∩ ρ ε 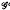
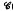 (
( ) then γ ν ρ = γ ο ρ = ρ ο γ.
) then γ ν ρ = γ ο ρ = ρ ο γ.
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 40 , Issue 1 , February 1997 , pp. 63 - 67
- Copyright
- Copyright © Edinburgh Mathematical Society 1997
References
REFERENCES
- 4
- Cited by


