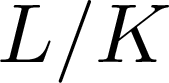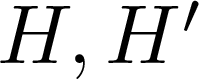1. Introduction
A Hopf–Galois structure on a finite extension of fields  $ L/K $ consists of a cocommutative K-Hopf algebra H making L into an H-module algebra satisfying a certain non-degeneracy condition (see [Reference Childs6, (2.7) Definition] for the full definition). The motivating example is a Galois extension
$ L/K $ consists of a cocommutative K-Hopf algebra H making L into an H-module algebra satisfying a certain non-degeneracy condition (see [Reference Childs6, (2.7) Definition] for the full definition). The motivating example is a Galois extension  $ L/K $ with Galois group G: in this case, the group algebra
$ L/K $ with Galois group G: in this case, the group algebra  $ K[G] $ (with its usual action on L) gives a Hopf–Galois structure on the extension. Hopf–Galois structures allow many results from Galois theory to be generalized to extensions which are inseparable or non-normal, but there is also considerable interest in their applications to Galois extensions: various authors have studied the number of Hopf–Galois structures admitted by particular extensions, the structure of the Hopf algebras involved and questions concerning the Hopf–Galois analogue of the Galois correspondence. We highlight two applications in particular. First, it has recently been discovered that each Hopf–Galois structure on a Galois extension yields a skew left brace; these objects are intensively studied because they can be used to generate set-theoretic solutions of the Yang–Baxter equation. The translation of existence, classification and structural results between Hopf–Galois theory and skew brace theory has enriched both disciplines. Second, Hopf–Galois theory has been applied fruitfully to questions originating in Galois module theory: if
$ K[G] $ (with its usual action on L) gives a Hopf–Galois structure on the extension. Hopf–Galois structures allow many results from Galois theory to be generalized to extensions which are inseparable or non-normal, but there is also considerable interest in their applications to Galois extensions: various authors have studied the number of Hopf–Galois structures admitted by particular extensions, the structure of the Hopf algebras involved and questions concerning the Hopf–Galois analogue of the Galois correspondence. We highlight two applications in particular. First, it has recently been discovered that each Hopf–Galois structure on a Galois extension yields a skew left brace; these objects are intensively studied because they can be used to generate set-theoretic solutions of the Yang–Baxter equation. The translation of existence, classification and structural results between Hopf–Galois theory and skew brace theory has enriched both disciplines. Second, Hopf–Galois theory has been applied fruitfully to questions originating in Galois module theory: if  $ L/K $ is a finite extension of local or global fields and H is a Hopf algebra giving a Hopf–Galois structure on the extension then we may study each fractional ideal
$ L/K $ is a finite extension of local or global fields and H is a Hopf algebra giving a Hopf–Galois structure on the extension then we may study each fractional ideal  $ \mathfrak B $ of L as a module over its associated order in H. This raises the possibility of comparing the structure of
$ \mathfrak B $ of L as a module over its associated order in H. This raises the possibility of comparing the structure of  $ \mathfrak B $ as a module over its associated orders in the various Hopf–Galois structures admitted by the extension. We refer the reader to [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9] for a recent survey of these and other related topics.
$ \mathfrak B $ as a module over its associated orders in the various Hopf–Galois structures admitted by the extension. We refer the reader to [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9] for a recent survey of these and other related topics.
A theorem of Greither and Pareigis [Reference Greither and Pareigis12] classifies the Hopf–Galois structures admitted by a finite separable extension of fields  $ L/K $. In the special case of Galois extension with Galois group G, their theorem implies that Hopf–Galois structures admitted by
$ L/K $. In the special case of Galois extension with Galois group G, their theorem implies that Hopf–Galois structures admitted by  $ L/K $ correspond bijectively with regular subgroups N of
$ L/K $ correspond bijectively with regular subgroups N of  $ \operatorname{Perm}(G) $ that are normalized by the image of the left regular representation
$ \operatorname{Perm}(G) $ that are normalized by the image of the left regular representation  $ \lambda : G \hookrightarrow \operatorname{Perm}(G) $. In [Reference Koch, Kohl, Truman and Underwood17], it is noted that such a subgroup N need not be normalized by the image of the right regular representation
$ \lambda : G \hookrightarrow \operatorname{Perm}(G) $. In [Reference Koch, Kohl, Truman and Underwood17], it is noted that such a subgroup N need not be normalized by the image of the right regular representation  $ \rho : G \hookrightarrow \operatorname{Perm}(G) $, and that for each
$ \rho : G \hookrightarrow \operatorname{Perm}(G) $, and that for each  $ g \in G $ the subgroup
$ g \in G $ the subgroup  $ \rho(g)N\rho(g)^{-1} $ is again regular and normalized by
$ \rho(g)N\rho(g)^{-1} $ is again regular and normalized by  $ \lambda(G) $ and therefore corresponds to a Hopf–Galois structure on
$ \lambda(G) $ and therefore corresponds to a Hopf–Galois structure on  $ L/K $. Following [Reference Koch and Truman16], we call this method of partitioning the set of Hopf–Galois structures on
$ L/K $. Following [Reference Koch and Truman16], we call this method of partitioning the set of Hopf–Galois structures on  $L/K\;\rho$-conjugation. We review existing results concerning ρ-conjugate Hopf–Galois structures in more detail in
$L/K\;\rho$-conjugation. We review existing results concerning ρ-conjugate Hopf–Galois structures in more detail in ![]() $\S$ 2 of this paper.
$\S$ 2 of this paper.
In ![]() $\S$ 3, we study the Hopf–Galois analogue of the Galois correspondence, showing that the lattices of subfields realized by ρ-conjugate Hopf–Galois structures are intimately related to one another. Section 4 concerns the skew braces corresponding to ρ-conjugate Hopf–Galois structures; we show that the number of distinct ρ-conjugates of a given Hopf–Galois structure is determined by the corresponding skew brace and obtain a criterion for a Hopf–Galois structure to have no non-trivial ρ-conjugates.
$\S$ 3, we study the Hopf–Galois analogue of the Galois correspondence, showing that the lattices of subfields realized by ρ-conjugate Hopf–Galois structures are intimately related to one another. Section 4 concerns the skew braces corresponding to ρ-conjugate Hopf–Galois structures; we show that the number of distinct ρ-conjugates of a given Hopf–Galois structure is determined by the corresponding skew brace and obtain a criterion for a Hopf–Galois structure to have no non-trivial ρ-conjugates.
In ![]() $\S$ 5, we suppose that
$\S$ 5, we suppose that  $ L/K $ is a Galois extension of local or global fields and study questions of integral module structure. We show that if an ambiguous ideal
$ L/K $ is a Galois extension of local or global fields and study questions of integral module structure. We show that if an ambiguous ideal  $ \mathfrak B $ of L is free over its associated order in a particular Hopf-Galois structure then it is free over its associated order in each of the Hopf–Galois structures that are ρ-conjugate to the original one. Finally, in
$ \mathfrak B $ of L is free over its associated order in a particular Hopf-Galois structure then it is free over its associated order in each of the Hopf–Galois structures that are ρ-conjugate to the original one. Finally, in ![]() $\S$ 6, we study how ρ-conjugation interacts with other constructions in the literature, including Hopf–Galois structures arising from fixed point free pairs of homomorphisms (due to Byott and Childs), those arising from abelian maps (instigated by Childs and generalized by Koch) and induced Hopf–Galois structures (due to Crespo, Rio and Vela).
$\S$ 6, we study how ρ-conjugation interacts with other constructions in the literature, including Hopf–Galois structures arising from fixed point free pairs of homomorphisms (due to Byott and Childs), those arising from abelian maps (instigated by Childs and generalized by Koch) and induced Hopf–Galois structures (due to Crespo, Rio and Vela).
2. ρ-Conjugate Hopf–Galois structures
In this section, we recall the definition of ρ-conjugate Hopf–Galois structures and some of their known properties.
Let  $ L/K $ be a Galois extension of fields with Galois group G. As mentioned in
$ L/K $ be a Galois extension of fields with Galois group G. As mentioned in ![]() $\S$ 1, a theorem of Griether and Pareigis [Reference Greither and Pareigis12] implies that the Hopf–Galois structures admitted by
$\S$ 1, a theorem of Griether and Pareigis [Reference Greither and Pareigis12] implies that the Hopf–Galois structures admitted by  $ L/K $ correspond bijectively with regular subgroups N of
$ L/K $ correspond bijectively with regular subgroups N of  $ \operatorname{Perm}(G) $ that are normalized by the image of G under the left regular representation
$ \operatorname{Perm}(G) $ that are normalized by the image of G under the left regular representation  $ \lambda : G \hookrightarrow \operatorname{Perm}(G) $. The normalization condition is equivalent to requiring that N is stable under the action of G on
$ \lambda : G \hookrightarrow \operatorname{Perm}(G) $. The normalization condition is equivalent to requiring that N is stable under the action of G on  $ \operatorname{Perm}(G) $ given by
$ \operatorname{Perm}(G) $ given by  $g\ast\eta=\lambda(g)\eta\lambda(g)^{-1}$ for all
$g\ast\eta=\lambda(g)\eta\lambda(g)^{-1}$ for all  $ \eta \in \operatorname{Perm}(G) $ and all
$ \eta \in \operatorname{Perm}(G) $ and all  $ g \in G $; accordingly, we shall refer to the subgroups of interest as G-stable regular subgroups. To ease notation when dealing with long compositions of permutations, we shall write
$ g \in G $; accordingly, we shall refer to the subgroups of interest as G-stable regular subgroups. To ease notation when dealing with long compositions of permutations, we shall write  $ \eta[g] $ for the effect of a permutation
$ \eta[g] $ for the effect of a permutation  $ \eta \in \operatorname{Perm}(G) $ on an element
$ \eta \in \operatorname{Perm}(G) $ on an element  $ g \in G $.
$ g \in G $.
In general, a G-stable regular subgroup N of  $ \operatorname{Perm}(G) $ need not be normalized by the image of the right regular representation
$ \operatorname{Perm}(G) $ need not be normalized by the image of the right regular representation  $ \rho : G \hookrightarrow \operatorname{Perm}(G) $. This motivates the following (see also [Reference Koch, Kohl, Truman and Underwood17, Example 2.7]).
$ \rho : G \hookrightarrow \operatorname{Perm}(G) $. This motivates the following (see also [Reference Koch, Kohl, Truman and Underwood17, Example 2.7]).
Proposition 2.1. Let N be a G-stable regular subgroup of  $ \operatorname{Perm}(G) $ and let
$ \operatorname{Perm}(G) $ and let  $ g \in G $. Then
$ g \in G $. Then  $ N_{g} := \rho(g)N\rho(g)^{-1} $ is a G-stable regular subgroup of
$ N_{g} := \rho(g)N\rho(g)^{-1} $ is a G-stable regular subgroup of  $ \operatorname{Perm}(G) $.
$ \operatorname{Perm}(G) $.
Proof. Clearly, Ng is a subgroup of  $ \operatorname{Perm}(G) $ that is isomorphic to N. Since N acts transitively on G, we have
$ \operatorname{Perm}(G) $ that is isomorphic to N. Since N acts transitively on G, we have
 \begin{equation*}N_{g} [g] = (N [e_{G}])g^{-1} = G g^{-1} = G, \end{equation*}
\begin{equation*}N_{g} [g] = (N [e_{G}])g^{-1} = G g^{-1} = G, \end{equation*}so Ng acts transitively on G. NgTherefore, Ng is a regular subgroup of  $ \operatorname{Perm}(G) $. To show G-stability, we note that
$ \operatorname{Perm}(G) $. To show G-stability, we note that  $ \lambda(G) $ and
$ \lambda(G) $ and  $ \rho(G) $ centralize one another inside
$ \rho(G) $ centralize one another inside  $ \operatorname{Perm}(G) $; hence, for
$ \operatorname{Perm}(G) $; hence, for  $ h \in G $ and
$ h \in G $ and  $ \eta \in N $, we have
$ \eta \in N $, we have
 \begin{equation*}\begin{array}{rlrlrlrlrlrl}h\ast\rho(g)\eta\rho(g)^{-1}&=\lambda(h)\rho(g)\eta\rho(g)^{-1}\lambda(h)^{-1}\\&=\rho(g)\lambda(h)\eta\lambda(h)^{-1}\rho(g)^{-1}\\&=\rho(g)(h\ast\eta)\rho(g)^{-1}.\end{array}\end{equation*}
\begin{equation*}\begin{array}{rlrlrlrlrlrl}h\ast\rho(g)\eta\rho(g)^{-1}&=\lambda(h)\rho(g)\eta\rho(g)^{-1}\lambda(h)^{-1}\\&=\rho(g)\lambda(h)\eta\lambda(h)^{-1}\rho(g)^{-1}\\&=\rho(g)(h\ast\eta)\rho(g)^{-1}.\end{array}\end{equation*} We have  $h\ast\eta\in N$ because N is G-stable; hence,
$h\ast\eta\in N$ because N is G-stable; hence,  $\rho(g)(h\ast\eta)\rho(g)^{-1}\in N_g$, and so Ng is G-stable.
$\rho(g)(h\ast\eta)\rho(g)^{-1}\in N_g$, and so Ng is G-stable.
Definition 2.2. We shall call two G-stable regular subgroups  $ N,N^\prime $ of
$ N,N^\prime $ of  $\textstyle\mathrm{Perm}(G)\;\rho$-conjugate to mean that
$\textstyle\mathrm{Perm}(G)\;\rho$-conjugate to mean that  $ N^\prime = N_{g} $ for some
$ N^\prime = N_{g} $ for some  $ g \in G $. In this case, we shall also say that the Hopf–Galois structures corresponding to
$ g \in G $. In this case, we shall also say that the Hopf–Galois structures corresponding to  $ N, N^\prime $ are ρ-conjugate to one another.
$ N, N^\prime $ are ρ-conjugate to one another.
It is clear that ρ-conjugation is an equivalence relation on the set of G-stable regular subgroups of  $ \operatorname{Perm}(G) $, and so yields an equivalence relation on the set of Hopf–Galois structures admitted by
$ \operatorname{Perm}(G) $, and so yields an equivalence relation on the set of Hopf–Galois structures admitted by  $ L/K $. Given a G-stable regular subgroup N, we have
$ L/K $. Given a G-stable regular subgroup N, we have  $ N_{g} = N_{h} $ if and only if
$ N_{g} = N_{h} $ if and only if  $ \rho(g^{-1}h) \in \mathrm{Norm}_{\scriptsize{\operatorname{Perm}(G)}}(N) $. In particular, if G is abelian then
$ \rho(g^{-1}h) \in \mathrm{Norm}_{\scriptsize{\operatorname{Perm}(G)}}(N) $. In particular, if G is abelian then  $ \rho(G) = \lambda(G) $, and since N is G-stable we have
$ \rho(G) = \lambda(G) $, and since N is G-stable we have  $ N_{g}=N $ for all
$ N_{g}=N $ for all  $ g \in G $. We shall return to the question of determining the number of distinct ρ-conjugates of a given G-stable regular subgroup in
$ g \in G $. We shall return to the question of determining the number of distinct ρ-conjugates of a given G-stable regular subgroup in ![]() $\S$ 4.
$\S$ 4.
Example 2.3. Let  $ p,q $ be prime numbers with
$ p,q $ be prime numbers with  $ p \equiv 1 \pmod{q} $, and let
$ p \equiv 1 \pmod{q} $, and let  $ L/K $ be a Galois extension whose Galois group G is isomorphic to the metacyclic group of order pq:
$ L/K $ be a Galois extension whose Galois group G is isomorphic to the metacyclic group of order pq:
 \begin{equation*} G = \big\langle s, t \mid s^{p}=t^{q}=e, \; t s t^{-1} = s^{d} \big\rangle, \end{equation*}
\begin{equation*} G = \big\langle s, t \mid s^{p}=t^{q}=e, \; t s t^{-1} = s^{d} \big\rangle, \end{equation*}where d is a positive integer of multiplicative order q modulo p. Let  $ \eta = \lambda(s) \rho(t) \in \operatorname{Perm}(G) $ and
$ \eta = \lambda(s) \rho(t) \in \operatorname{Perm}(G) $ and  $ N = \langle \eta \rangle $. It is routine to verify that N is G-stable and regular. We find that
$ N = \langle \eta \rangle $. It is routine to verify that N is G-stable and regular. We find that  $ N_{t} = N $ and that
$ N_{t} = N $ and that
 \begin{equation*} N_{s^{i}} = \langle \lambda(s) \rho(s^{i(1-d)}t) \rangle \end{equation*}
\begin{equation*} N_{s^{i}} = \langle \lambda(s) \rho(s^{i(1-d)}t) \rangle \end{equation*}for each  $ i=0, \ldots ,p-1 $. These subgroups are all distinct, and so we obtain a family of p mutually ρ-conjugate Hopf–Galois structures on
$ i=0, \ldots ,p-1 $. These subgroups are all distinct, and so we obtain a family of p mutually ρ-conjugate Hopf–Galois structures on  $ L/K $.
$ L/K $.
Example 2.4. Let n be an even natural number and let  $ L/K $ be a Galois extension whose Galois group G is isomorphic to the dihedral group of order 2n:
$ L/K $ be a Galois extension whose Galois group G is isomorphic to the dihedral group of order 2n:
 \begin{equation*} G = \big\langle r,s \mid r^{n}=s^{2}=e, \; srs^{-1} = r^{-1} \big\rangle. \end{equation*}
\begin{equation*} G = \big\langle r,s \mid r^{n}=s^{2}=e, \; srs^{-1} = r^{-1} \big\rangle. \end{equation*} Let  $ N = \langle \lambda(r) \rho(s), \lambda(s) \rangle $. Then N is G stable, regular and isomorphic to
$ N = \langle \lambda(r) \rho(s), \lambda(s) \rangle $. Then N is G stable, regular and isomorphic to  $ D_{2n} $. We find that
$ D_{2n} $. We find that  $ N_{s} = N $ and that
$ N_{s} = N $ and that
 \begin{equation*} N_{r^{k}} = \langle \lambda(r) \rho(r^{2k}s), \lambda(s) \rangle \end{equation*}
\begin{equation*} N_{r^{k}} = \langle \lambda(r) \rho(r^{2k}s), \lambda(s) \rangle \end{equation*}for each  $ k=0, \ldots ,n/2-1 $. These subgroups are all distinct, and so we obtain a family of
$ k=0, \ldots ,n/2-1 $. These subgroups are all distinct, and so we obtain a family of  $ n/2 $ mutually ρ-conjugate Hopf–Galois structures on
$ n/2 $ mutually ρ-conjugate Hopf–Galois structures on  $ L/K $.
$ L/K $.
Byott [Reference Byott4] enumerates the Hopf–Galois structures admitted by a Galois extension of degree pq. In particular, [Reference Byott4, Theorem 6.2] implies that the Hopf–Galois structures corresponding to the G-stable regular subgroups constructed in Example 2.3 are all of those for which the corresponding subgroup of  $ \operatorname{Perm}(G) $ is cyclic (i.e., those of cyclic type). On the other hand, Kohl [Reference Kohl19] enumerates the Hopf–Galois structures of dihedral type admitted by a Galois extension whose Galois group is isomorphic to the dihedral group of order 2n; the precise formula depends upon the congruence class of n modulo 8, but if n is even there are at least
$ \operatorname{Perm}(G) $ is cyclic (i.e., those of cyclic type). On the other hand, Kohl [Reference Kohl19] enumerates the Hopf–Galois structures of dihedral type admitted by a Galois extension whose Galois group is isomorphic to the dihedral group of order 2n; the precise formula depends upon the congruence class of n modulo 8, but if n is even there are at least  $ (n/2+1)|\Upsilon_{n}| $, where
$ (n/2+1)|\Upsilon_{n}| $, where  $ |\Upsilon_{n}| $ is the number of square roots of 1 modulo n. The Hopf–Galois structures corresponding to the G-stable regular subgroups constructed in Example 2.4 account for less than half of these. Similarly, it is known that a Galois extension with Galois group isomorphic to the quaternion group of order 8 admits 22 Hopf–Galois structures [Reference Smoktunowicz and Vendramin20, Table A.1]. Using the descriptions of the corresponding G-stable regular subgroups found in [Reference Taylor and Truman22], we find that the six cyclic subgroups fall into threee pairs under ρ-conjugation, whereas each of the remaining 16 subgroups is normalized by
$ |\Upsilon_{n}| $ is the number of square roots of 1 modulo n. The Hopf–Galois structures corresponding to the G-stable regular subgroups constructed in Example 2.4 account for less than half of these. Similarly, it is known that a Galois extension with Galois group isomorphic to the quaternion group of order 8 admits 22 Hopf–Galois structures [Reference Smoktunowicz and Vendramin20, Table A.1]. Using the descriptions of the corresponding G-stable regular subgroups found in [Reference Taylor and Truman22], we find that the six cyclic subgroups fall into threee pairs under ρ-conjugation, whereas each of the remaining 16 subgroups is normalized by  $ \rho(G) $. We expect the situation of Example 2.3, in which all of the G-stable regular subgroups of a given type are mutually ρ-conjugate, to be rare.
$ \rho(G) $. We expect the situation of Example 2.3, in which all of the G-stable regular subgroups of a given type are mutually ρ-conjugate, to be rare.
We shall shed more light on these examples, and construct others, in ![]() $\S$ 6.
$\S$ 6.
In Greither and Pareigis’s original paper classifying Hopf–Galois structures on separable extensions [Reference Greither and Pareigis12] they show that if N is a G-stable regular subgroup of  $ \operatorname{Perm}(G) $ then so too is
$ \operatorname{Perm}(G) $ then so too is
 \begin{equation*} N^{\rm opp} = \mathrm{Cent}_{{\tiny \operatorname{Perm}(G)}}(N). \end{equation*}
\begin{equation*} N^{\rm opp} = \mathrm{Cent}_{{\tiny \operatorname{Perm}(G)}}(N). \end{equation*} In [Reference Koch and Truman15], the Hopf–Galois structure corresponding to N opp is called the opposite of the one corresponding to N, and [Reference Koch and Truman16, Corollary 7.2] shows that for each  $ g \in G $ we have
$ g \in G $ we have  $ (N_{g})^{\rm opp} = (N^{\rm opp})_{g} $. We shall see fruitful applications of this interaction in
$ (N_{g})^{\rm opp} = (N^{\rm opp})_{g} $. We shall see fruitful applications of this interaction in ![]() $\S$ 5.
$\S$ 5.
Example 2.5. Recall the hypotheses and notation of Example 2.4 above. The opposite of the subgroup N constructed in that example is the subgroup generated by  $ \rho(s) $ and the permutation µ 0 defined by
$ \rho(s) $ and the permutation µ 0 defined by
 \begin{equation*} \mu_{0}[r^{i}s^{j}] = r^{i+(-1)^{i+j}} s^{j}. \end{equation*}
\begin{equation*} \mu_{0}[r^{i}s^{j}] = r^{i+(-1)^{i+j}} s^{j}. \end{equation*} Exploiting the interaction between opposite subgroups and ρ-conjugation, we find that for each  $ k=0, \ldots ,n/2-1 $ the opposite of the subgroup
$ k=0, \ldots ,n/2-1 $ the opposite of the subgroup  $ N_{r^{k}} $ is generated by
$ N_{r^{k}} $ is generated by  $ \rho(s) $ and the permutation µ k defined by
$ \rho(s) $ and the permutation µ k defined by
 \begin{equation*} \mu_{k}[r^{i}s^{j}] = r^{i+(-1)^{i+j+k}} s^{j}. \end{equation*}
\begin{equation*} \mu_{k}[r^{i}s^{j}] = r^{i+(-1)^{i+j+k}} s^{j}. \end{equation*} The Hopf algebra giving the Hopf–Galois structure corresponding to a G-stable regular subgroup N is the fixed ring  $ L[N]^{G} $, where G acts on L via the usual Galois action and on N via
$ L[N]^{G} $, where G acts on L via the usual Galois action and on N via  $g\ast\eta=\lambda(g)\eta\lambda(g)^{-1}$. It is possible for two distinct Hopf–Galois structures on
$g\ast\eta=\lambda(g)\eta\lambda(g)^{-1}$. It is possible for two distinct Hopf–Galois structures on  $ L/K $ to involve isomorphic Hopf algebras, and it is well known (see [Reference Koch, Kohl, Truman and Underwood17, Corollary 2.3], for example) that we have
$ L/K $ to involve isomorphic Hopf algebras, and it is well known (see [Reference Koch, Kohl, Truman and Underwood17, Corollary 2.3], for example) that we have  $ L[N]^{G} \cong L[N^\prime]^{G} $ if and only if there is a group isomorphism
$ L[N]^{G} \cong L[N^\prime]^{G} $ if and only if there is a group isomorphism  $ \phi : N \xrightarrow{\sim} N^\prime $ such that
$ \phi : N \xrightarrow{\sim} N^\prime $ such that  $h\ast\phi(\eta)=\phi(h\ast\eta)$ for all
$h\ast\phi(\eta)=\phi(h\ast\eta)$ for all  $ \eta \in N $ and all
$ \eta \in N $ and all  $ h \in G $. More explicitly, ϕ induces an isomorphism of L-Hopf algebras
$ h \in G $. More explicitly, ϕ induces an isomorphism of L-Hopf algebras  $ \phi : L[N] \xrightarrow{\sim} L[N^\prime] $, which descends to an isomorphism of K-Hopf algebras
$ \phi : L[N] \xrightarrow{\sim} L[N^\prime] $, which descends to an isomorphism of K-Hopf algebras  $ \phi : L[N]^{G} \xrightarrow{\sim} L[N^\prime]^{G} $.
$ \phi : L[N]^{G} \xrightarrow{\sim} L[N^\prime]^{G} $.
Turning to ρ-conjugate subgroups, we have (see also [Reference Koch, Kohl, Truman and Underwood17, Example 2.7])
Proposition 2.6. For each  $ g \in G $, we have
$ g \in G $, we have  $ L[N]^{G} \cong L[N_{g}]^{G} $ as K-Hopf algebras.
$ L[N]^{G} \cong L[N_{g}]^{G} $ as K-Hopf algebras.
Proof. Consider the isomorphism  $ \phi : N \rightarrow N_{g} $ defined by
$ \phi : N \rightarrow N_{g} $ defined by  $ \phi(\eta) = \rho(g)\eta\rho(g)^{-1} $ for all
$ \phi(\eta) = \rho(g)\eta\rho(g)^{-1} $ for all  $ \eta \in N $. By the proof of Proposition 2.1, we have
$ \eta \in N $. By the proof of Proposition 2.1, we have  $h\ast\phi(\eta)=\phi(h\ast\eta)$ for all
$h\ast\phi(\eta)=\phi(h\ast\eta)$ for all  $ \eta \in N $ and all
$ \eta \in N $ and all  $ h \in G $, so ϕ yields an isomorphism of K-Hopf algebras
$ h \in G $, so ϕ yields an isomorphism of K-Hopf algebras  $ \phi: L[N]^{G} \xrightarrow{\sim} L[N_{g}]^{G} $. Explicitly, we have
$ \phi: L[N]^{G} \xrightarrow{\sim} L[N_{g}]^{G} $. Explicitly, we have
 \begin{equation}
\phi \left( \sum_{\eta \in N} c_{\eta} \eta \right) = \sum_{\eta \in N} c_{\eta} \rho(g) \eta \rho(g)^{-1}.
\end{equation}
\begin{equation}
\phi \left( \sum_{\eta \in N} c_{\eta} \eta \right) = \sum_{\eta \in N} c_{\eta} \rho(g) \eta \rho(g)^{-1}.
\end{equation}3. The Hopf–Galois correspondence
If a Hopf algebra H gives a Hopf–Galois structure on a finite extension of fields  $ L/K $ then each Hopf subalgebra Hʹ of H has a corresponding fixed field
$ L/K $ then each Hopf subalgebra Hʹ of H has a corresponding fixed field
 \begin{equation*} L^{H^\prime} = \{x \in L \mid z \cdot x = \varepsilon(z)x\quad \mbox{for all } z \in H^{\prime}\}, \end{equation*}
\begin{equation*} L^{H^\prime} = \{x \in L \mid z \cdot x = \varepsilon(z)x\quad \mbox{for all } z \in H^{\prime}\}, \end{equation*}where  $ \varepsilon: H \rightarrow K $ denotes the counit map of H. In analogy with the classical Galois correspondence, we have
$ \varepsilon: H \rightarrow K $ denotes the counit map of H. In analogy with the classical Galois correspondence, we have  $ [L:L^{H^\prime}] = \dim_{K}(H^{\prime})$. The correspondence from Hopf subalgebras of H to intermediate fields of the extension
$ [L:L^{H^\prime}] = \dim_{K}(H^{\prime})$. The correspondence from Hopf subalgebras of H to intermediate fields of the extension  $ L/K $ is inclusion reversing and injective, but not surjective in general; we say that an intermediate field M is realizable with respect to H to mean that there is a Hopf subalgebra Hʹ of H such that
$ L/K $ is inclusion reversing and injective, but not surjective in general; we say that an intermediate field M is realizable with respect to H to mean that there is a Hopf subalgebra Hʹ of H such that  $ L^{H^\prime} = M $. The proportion of the intermediate fields of the extension
$ L^{H^\prime} = M $. The proportion of the intermediate fields of the extension  $ L/K $ that are realizable with respect to H has been called the Galois correspondence ratio for H and has been studied by several authors in recent years, with particular interest in situations for which it is equal to 1 (see [Reference Childs8], [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9, Chapter 7] or [Reference Stefanello and Trappeniers21], for example).
$ L/K $ that are realizable with respect to H has been called the Galois correspondence ratio for H and has been studied by several authors in recent years, with particular interest in situations for which it is equal to 1 (see [Reference Childs8], [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9, Chapter 7] or [Reference Stefanello and Trappeniers21], for example).
Specializing to the case in which  $ L/K $ is a Galois extension, we have seen that each Hopf algebra giving a Hopf–Galois structure on
$ L/K $ is a Galois extension, we have seen that each Hopf algebra giving a Hopf–Galois structure on  $ L/K $ has the form
$ L/K $ has the form  $ L[N]^{G} $ with N some G-stable regular subgroup of
$ L[N]^{G} $ with N some G-stable regular subgroup of  $ \operatorname{Perm}(G) $; in this case, the Hopf subalgebras of
$ \operatorname{Perm}(G) $; in this case, the Hopf subalgebras of  $ L[N]^{G} $ are precisely the sets
$ L[N]^{G} $ are precisely the sets  $ L[P]^{G} $ with P a subgroup of N that is itself G-stable (see [Reference Crespo, Rio and Vela11, Theorem 2.3] or [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9, Theorem 7.2]). Abusing notation, we write L P in place of
$ L[P]^{G} $ with P a subgroup of N that is itself G-stable (see [Reference Crespo, Rio and Vela11, Theorem 2.3] or [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9, Theorem 7.2]). Abusing notation, we write L P in place of  $ L^{L[P]^{G}} $; since
$ L^{L[P]^{G}} $; since  $ \dim_{K}(L[P]^{G}) = |P| $, we then have
$ \dim_{K}(L[P]^{G}) = |P| $, we then have  $ [L:L^{P}]=|P| $.
$ [L:L^{P}]=|P| $.
Turning to ρ-conjugate subgroups, we have
Proposition 3.1. For each  $ g \in G $, the lattices of intermediate fields of
$ g \in G $, the lattices of intermediate fields of  $ L/K $ realizable with respect to the Hopf–Galois structures given by
$ L/K $ realizable with respect to the Hopf–Galois structures given by  $ L[N]^{G} $ and
$ L[N]^{G} $ and  $ L[N_{g}]^{G} $ are isomorphic.
$ L[N_{g}]^{G} $ are isomorphic.
Proof. By virtue of the Hopf algebra isomorphism  $ \phi : L[N]^{G} \xrightarrow{\sim} L[N_{g}]^{G} $ constructed in Proposition 2.6, the lattices of Hopf subalgebras of
$ \phi : L[N]^{G} \xrightarrow{\sim} L[N_{g}]^{G} $ constructed in Proposition 2.6, the lattices of Hopf subalgebras of  $ L[N]^{G} $ and
$ L[N]^{G} $ and  $ L[N_{g}]^{G} $ are isomorphic. The result then follows from the discussion above.
$ L[N_{g}]^{G} $ are isomorphic. The result then follows from the discussion above.
As a consequence of this, the Galois correspondence ratios for the Hopf–Galois structures given by  $ L[N]^{G} $ and
$ L[N]^{G} $ and  $ L[N_{g}]^{G} $ are the same for all
$ L[N_{g}]^{G} $ are the same for all  $ g \in G $. In fact, we can identify precisely how the intermediate fields realizable with respect to
$ g \in G $. In fact, we can identify precisely how the intermediate fields realizable with respect to  $ L[N]^{G} $ and
$ L[N]^{G} $ and  $ L[N_{g}]^{G} $ are related to one another. As a first step, we study the actions of these Hopf algebras on the field L.
$ L[N_{g}]^{G} $ are related to one another. As a first step, we study the actions of these Hopf algebras on the field L.
It follows from the theorem of Greither and Pareigis that the action of an element  $ \sum_{\eta \in N} c_{\eta} \eta \in L[N]^{G} $ on L is given by
$ \sum_{\eta \in N} c_{\eta} \eta \in L[N]^{G} $ on L is given by
 \begin{equation}
\left( \sum_{\eta \in N} c_{\eta} \eta \right) \cdot x = \sum_{\eta \in N} c_{\eta} \eta^{-1}[e_{G}](x)\quad \mbox{for all } x \in L
\end{equation}
\begin{equation}
\left( \sum_{\eta \in N} c_{\eta} \eta \right) \cdot x = \sum_{\eta \in N} c_{\eta} \eta^{-1}[e_{G}](x)\quad \mbox{for all } x \in L
\end{equation} (see [Reference Childs, Greither, Keating, Koch, Kohl, Truman and Underwood9, Proposition 4.14], for example). The action of  $ L[N_{g}]^{G} $ on L is given by an analogous formula. We describe how the isomorphism ϕ interacts with these actions.
$ L[N_{g}]^{G} $ on L is given by an analogous formula. We describe how the isomorphism ϕ interacts with these actions.
Proposition 3.2. Let  $ x \in L $. Then for all
$ x \in L $. Then for all  $ z \in L[N]^{G} $, we have
$ z \in L[N]^{G} $, we have
 \begin{equation*} \phi(z) \cdot x = g ( z \cdot g^{-1}(x) ). \end{equation*}
\begin{equation*} \phi(z) \cdot x = g ( z \cdot g^{-1}(x) ). \end{equation*}Proof. Write  $ z = \sum_{\eta \in N} c_{\eta} \eta $ with
$ z = \sum_{\eta \in N} c_{\eta} \eta $ with  $ c_{\eta} \in L $. Since
$ c_{\eta} \in L $. Since  $ z \in L[N]^{G} $, we have
$ z \in L[N]^{G} $, we have  $z=h\ast z$ for all
$z=h\ast z$ for all  $ h \in G $. In particular,
$ h \in G $. In particular,
 \begin{equation}z=g\ast z=\sum_{\eta\in N}g(c_\eta)\lambda(g)\eta\lambda(g)^{-1},\end{equation}
\begin{equation}z=g\ast z=\sum_{\eta\in N}g(c_\eta)\lambda(g)\eta\lambda(g)^{-1},\end{equation}and so
 \begin{align*}
\phi(z) & = \sum_{\eta \in N} g(c_{\eta}) \rho(g) \lambda(g) \eta \lambda(g)^{-1} \rho(g)^{-1} \\
& = \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta \rho(g)^{-1}\lambda(g)^{-1}
\end{align*}
\begin{align*}
\phi(z) & = \sum_{\eta \in N} g(c_{\eta}) \rho(g) \lambda(g) \eta \lambda(g)^{-1} \rho(g)^{-1} \\
& = \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta \rho(g)^{-1}\lambda(g)^{-1}
\end{align*}since  $ \lambda(G) $ and
$ \lambda(G) $ and  $ \rho(G) $ centralize each other inside
$ \rho(G) $ centralize each other inside  $ \operatorname{Perm}(G) $. Therefore,
$ \operatorname{Perm}(G) $. Therefore,
 \begin{align*}
\phi(z) \cdot x & = \left( \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta \rho(g)^{-1}\lambda(g)^{-1} \right) \cdot x \\
& = \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta^{-1} \rho(g)^{-1}\lambda(g)^{-1}[e_{G}](x) \\
& = \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta^{-1} [g^{-1}g] (x) \\
& = \sum_{\eta \in N} g(c_{\eta}) g \eta^{-1} [e_{G}] g^{-1} (x) \\
& = g \left( \sum_{\eta \in N} c_{\eta} \eta^{-1} [e_{G}] g^{-1} (x) \right) \\
& = g \left( \sum_{\eta \in N} c_{\eta} \eta \right) \cdot g^{-1} (x) \\
& = g \left( z \cdot g^{-1} (x)\right),
\end{align*}
\begin{align*}
\phi(z) \cdot x & = \left( \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta \rho(g)^{-1}\lambda(g)^{-1} \right) \cdot x \\
& = \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta^{-1} \rho(g)^{-1}\lambda(g)^{-1}[e_{G}](x) \\
& = \sum_{\eta \in N} g(c_{\eta}) \lambda(g)\rho(g) \eta^{-1} [g^{-1}g] (x) \\
& = \sum_{\eta \in N} g(c_{\eta}) g \eta^{-1} [e_{G}] g^{-1} (x) \\
& = g \left( \sum_{\eta \in N} c_{\eta} \eta^{-1} [e_{G}] g^{-1} (x) \right) \\
& = g \left( \sum_{\eta \in N} c_{\eta} \eta \right) \cdot g^{-1} (x) \\
& = g \left( z \cdot g^{-1} (x)\right),
\end{align*}as claimed.
Now we have the following:
Theorem 3.3. An intermediate field M of  $ L/K $ is realizable with respect to
$ L/K $ is realizable with respect to  $ L[N]^{G} $ if and only if the intermediate field g(M) is realizable with respect to
$ L[N]^{G} $ if and only if the intermediate field g(M) is realizable with respect to  $ L[N_{g}]^{G} $.
$ L[N_{g}]^{G} $.
Proof. Suppose that M is realizable with respect to  $ L[N]^{G} $. Then
$ L[N]^{G} $. Then  $ M = L^{P} $ for some G-stable subgroup P of N. We have
$ M = L^{P} $ for some G-stable subgroup P of N. We have  $ \phi(L[P]^{G}) = L[P_{g}]^{G} $, a Hopf subalgebra of
$ \phi(L[P]^{G}) = L[P_{g}]^{G} $, a Hopf subalgebra of  $ L[N_{g}]^{G} $, and for
$ L[N_{g}]^{G} $, and for  $ x \in L $ we have
$ x \in L $ we have
 \begin{equation*}\begin{array}{rcl}x\in L^{P_g}&\Leftrightarrow&\phi(z) \cdot x =\varepsilon(z)x \hspace{1em}{\textstyle\text{for all }}z\in L\lbrack P\rbrack^G\\&\Leftrightarrow&g(z \cdot g^{-1}(x))=\varepsilon(z)x\hspace{1em}{\textstyle\text{for all }}z\in L\lbrack P\rbrack^G,{\textstyle\text{by Proposition 3.2}}\\&\Leftrightarrow&z \cdot g^{-1}(x)=\varepsilon(z)g^{-1}(x)\hspace{1em}{\textstyle\text{for all }}z\in L\lbrack P\rbrack^G\\&\Leftrightarrow&g^{-1}(x)\in L^P=M\\&\Leftrightarrow&x\in g(M).\end{array}\end{equation*}
\begin{equation*}\begin{array}{rcl}x\in L^{P_g}&\Leftrightarrow&\phi(z) \cdot x =\varepsilon(z)x \hspace{1em}{\textstyle\text{for all }}z\in L\lbrack P\rbrack^G\\&\Leftrightarrow&g(z \cdot g^{-1}(x))=\varepsilon(z)x\hspace{1em}{\textstyle\text{for all }}z\in L\lbrack P\rbrack^G,{\textstyle\text{by Proposition 3.2}}\\&\Leftrightarrow&z \cdot g^{-1}(x)=\varepsilon(z)g^{-1}(x)\hspace{1em}{\textstyle\text{for all }}z\in L\lbrack P\rbrack^G\\&\Leftrightarrow&g^{-1}(x)\in L^P=M\\&\Leftrightarrow&x\in g(M).\end{array}\end{equation*} Hence, g(M) is realizable with respect to  $ L[N_{g}]^{G} $. For the converse statement, we replace N by Ng and g by g −1 in the argument above.
$ L[N_{g}]^{G} $. For the converse statement, we replace N by Ng and g by g −1 in the argument above.
Example 3.4. Recall the hypotheses and notation of Example 2.3 above. Each of the subgroups  $N_{s^{i}}$ is cyclic of order pq and therefore has a unique subgroup Pi of order p and a unique subgroup Q i of order q; by uniqueness, each of these is G-stable. Since
$N_{s^{i}}$ is cyclic of order pq and therefore has a unique subgroup Pi of order p and a unique subgroup Q i of order q; by uniqueness, each of these is G-stable. Since  $ N_{s^{i}} $ is generated by
$ N_{s^{i}} $ is generated by  $ \lambda(s) \rho(s^{i(1-d)}t) $, we see that
$ \lambda(s) \rho(s^{i(1-d)}t) $, we see that  $ P_{i} = \langle \lambda(s) \rangle $ regardless of i, and that
$ P_{i} = \langle \lambda(s) \rangle $ regardless of i, and that  $ Q_{i} = \langle \rho(s^{i(1-d)}t) \rangle $. Therefore,
$ Q_{i} = \langle \rho(s^{i(1-d)}t) \rangle $. Therefore,  $ L^{P_{i}} = L^{\langle s \rangle} $ (the unique intermediate field of degree p over K) and
$ L^{P_{i}} = L^{\langle s \rangle} $ (the unique intermediate field of degree p over K) and  $ L^{Q} = L^{\langle s^{i(1-d)}t \rangle} $. Thus, each of this family of ρ-conjugate Hopf–Galois structures realizes the intermediate fields
$ L^{Q} = L^{\langle s^{i(1-d)}t \rangle} $. Thus, each of this family of ρ-conjugate Hopf–Galois structures realizes the intermediate fields  $ L, K, L^{\langle s \rangle} $, and a unique intermediate field of degree q over K, and each intermediate field of degree q over K is realized by exactly one Hopf–Galois structure in this family.
$ L, K, L^{\langle s \rangle} $, and a unique intermediate field of degree q over K, and each intermediate field of degree q over K is realized by exactly one Hopf–Galois structure in this family.
4. Skew braces
A (left) skew brace is a triple  $ (B,\star,\circ) $, where B is a set and
$ (B,\star,\circ) $, where B is a set and  $\star,\circ $ are binary operations on B, each making B into a group, such that
$\star,\circ $ are binary operations on B, each making B into a group, such that
 \begin{equation}
x \circ (y \star z) = (x \circ y) \star x^{-1} \star (x \circ z)\quad \mbox{for all } x,y,z \in B.
\end{equation}
\begin{equation}
x \circ (y \star z) = (x \circ y) \star x^{-1} \star (x \circ z)\quad \mbox{for all } x,y,z \in B.
\end{equation} Here x −1 denotes the inverse of x with respect to ![]() $ \star $; we denote the inverse of x with respect to
$ \star $; we denote the inverse of x with respect to ![]() $ \circ $ by
$ \circ $ by ![]() $ \overline{x} $. We call Equation 3 the left skew brace relation; the right skew brace relation is analogous. We call a skew brace two-sided if both the left and right skew brace relations hold for all triples of elements of B. Skew braces with underlying set B yield solutions of the set theoretic Yang–Baxter equation on B; we can then obtain solutions of the Yang–Baxter equation over any field by using B as the basis for a vector space (see [Reference Koch, Stordy and Truman18, § 2], for example).
$ \overline{x} $. We call Equation 3 the left skew brace relation; the right skew brace relation is analogous. We call a skew brace two-sided if both the left and right skew brace relations hold for all triples of elements of B. Skew braces with underlying set B yield solutions of the set theoretic Yang–Baxter equation on B; we can then obtain solutions of the Yang–Baxter equation over any field by using B as the basis for a vector space (see [Reference Koch, Stordy and Truman18, § 2], for example).
The connection between skew braces and Hopf–Galois structures on Galois extensions follows from an observation by Bachiller [Reference Bachiller1, Remark 2.6]. One formulation is as follows: a finite skew brace  $ (B,\star,\circ) $ yields two left regular representation maps
$ (B,\star,\circ) $ yields two left regular representation maps  $ \lambda_{\star}, \lambda_{\circ} : B \hookrightarrow \operatorname{Perm}(B) $; we find that
$ \lambda_{\star}, \lambda_{\circ} : B \hookrightarrow \operatorname{Perm}(B) $; we find that  $ \lambda_{\star}(B) $ is a
$ \lambda_{\star}(B) $ is a  $ (B,\circ) $-stable regular subgroup of
$ (B,\circ) $-stable regular subgroup of  $ \operatorname{Perm}(B) $ and so corresponds to a Hopf–Galois structure on a Galois extension with Galois group
$ \operatorname{Perm}(B) $ and so corresponds to a Hopf–Galois structure on a Galois extension with Galois group  $ (B,\circ) $. Conversely, if
$ (B,\circ) $. Conversely, if  $ G = (G,\circ) $ is a finite group and N is a G-stable regular subgroup of
$ G = (G,\circ) $ is a finite group and N is a G-stable regular subgroup of  $ \operatorname{Perm}(G) $ then the map
$ \operatorname{Perm}(G) $ then the map  $ \eta \mapsto \eta[e_{G}] $ is a bijection from N to G; we use this to define a second binary operation
$ \eta \mapsto \eta[e_{G}] $ is a bijection from N to G; we use this to define a second binary operation ![]() $ \star $ on G by
$ \star $ on G by
 \begin{equation*} \eta[e_{G}] \star \mu[e_{G}] = \eta\mu[e_{G}]. \end{equation*}
\begin{equation*} \eta[e_{G}] \star \mu[e_{G}] = \eta\mu[e_{G}]. \end{equation*} Then  $ (G,\star) \cong N $, and the fact that N is G-stable implies that
$ (G,\star) \cong N $, and the fact that N is G-stable implies that  $ (G, \star, \circ) $ is a skew brace. As noted in [Reference Zenouz25, Proposition 2.1] and [Reference Koch and Truman16, Proposition 3.1], two G-stable regular subgroups
$ (G, \star, \circ) $ is a skew brace. As noted in [Reference Zenouz25, Proposition 2.1] and [Reference Koch and Truman16, Proposition 3.1], two G-stable regular subgroups  $ N, N^\prime \leq \operatorname{Perm}(G) $ yield isomorphic skew braces if and only if
$ N, N^\prime \leq \operatorname{Perm}(G) $ yield isomorphic skew braces if and only if  $ N^\prime = \varphi^{-1} N \varphi $ for some
$ N^\prime = \varphi^{-1} N \varphi $ for some  $ \varphi \in \operatorname{Aut}(G,\circ) $ and yield the same skew brace if and only if
$ \varphi \in \operatorname{Aut}(G,\circ) $ and yield the same skew brace if and only if  $ N^\prime = \varphi^{-1} N \varphi $ for some
$ N^\prime = \varphi^{-1} N \varphi $ for some  $ \varphi \in \operatorname{Aut}(G, \star, \circ) $ (the group of automorphisms of
$ \varphi \in \operatorname{Aut}(G, \star, \circ) $ (the group of automorphisms of  $ (G,\circ) $ that also respect
$ (G,\circ) $ that also respect ![]() $ \star $). Since these results involve conjugation by automorphisms of
$ \star $). Since these results involve conjugation by automorphisms of  $ (G,\circ) $, the following alternative formulation of ρ-conjugate subgroups is useful when studying connections with skew braces (see also [Reference Koch and Truman16, Proposition 4.1]). We note, however, that this formulation is less suitable for studying results such as Proposition 2.6 and Proposition 3.2, whose proofs depend heavily on the fact that
$ (G,\circ) $, the following alternative formulation of ρ-conjugate subgroups is useful when studying connections with skew braces (see also [Reference Koch and Truman16, Proposition 4.1]). We note, however, that this formulation is less suitable for studying results such as Proposition 2.6 and Proposition 3.2, whose proofs depend heavily on the fact that  $ \lambda(G) $ and
$ \lambda(G) $ and  $ \rho(G) $ centralize each other inside
$ \rho(G) $ centralize each other inside  $ \operatorname{Perm}(G) $.
$ \operatorname{Perm}(G) $.
Proposition 4.1. Let N be a G-stable regular subgroup of  $ \operatorname{Perm}(G) $, and let
$ \operatorname{Perm}(G) $, and let  $ g \in G $. Then
$ g \in G $. Then  $ N_{g} = \varphi_{g} N \varphi_{g}^{-1} $, where φ g is the inner automorphism of G corresponding to g.
$ N_{g} = \varphi_{g} N \varphi_{g}^{-1} $, where φ g is the inner automorphism of G corresponding to g.
Proof. We may write  $ \varphi_{g} = \rho(g) \lambda(g) $, and we then have
$ \varphi_{g} = \rho(g) \lambda(g) $, and we then have
 \begin{align*}
\varphi_{g} N \varphi_{g}^{-1} & = \rho(g) \lambda(g) N \lambda(g)^{-1} \rho(g)^{-1} \\
& =\rho(g) N \rho(g)^{-1}\ \mathrm{since}\ N\ \mathrm{is}\ \mathrm{G}{\rm-stable } \\
& = N_{g}.
\end{align*}
\begin{align*}
\varphi_{g} N \varphi_{g}^{-1} & = \rho(g) \lambda(g) N \lambda(g)^{-1} \rho(g)^{-1} \\
& =\rho(g) N \rho(g)^{-1}\ \mathrm{since}\ N\ \mathrm{is}\ \mathrm{G}{\rm-stable } \\
& = N_{g}.
\end{align*}This observation immediately implies that ρ-conjugate Hopf–Galois structures yield isomorphic skew braces under the correspondence described above ([Reference Koch and Truman16, Corollary 4.1]).
The number of distinct ρ-conjugates of a given G-stable regular subgroup N of  $ \operatorname{Perm}(G) $ can be studied via the corresponding skew brace.
$ \operatorname{Perm}(G) $ can be studied via the corresponding skew brace.
Theorem 4.2. Let N be a G-stable regular subgroup of  $ \operatorname{Perm}(G) $, let
$ \operatorname{Perm}(G) $, let  $ (G,\star,\circ) $ be the corresponding skew brace and let
$ (G,\star,\circ) $ be the corresponding skew brace and let  $ g \in G $. The following are equivalent:
$ g \in G $. The following are equivalent:
(i) The G-stable regular subgroup N is normalized by
 $ \rho(g) $.
$ \rho(g) $.(ii) The inner automorphism φ g of
 $ \operatorname{Aut}(G,\circ) $ is an element of
$ \operatorname{Aut}(G,\circ) $ is an element of  $ \operatorname{Aut}(G,\star,\circ) $.
$ \operatorname{Aut}(G,\star,\circ) $.(iii) We have
 $ (y \star z) \circ g = (y \circ g ) \star g^{-1} \star (z \circ g) $ for all
$ (y \star z) \circ g = (y \circ g ) \star g^{-1} \star (z \circ g) $ for all  $ y,z \in G $.
$ y,z \in G $.
Corollary 4.3. Let  $ G^\prime = \{g \in G \mid \varphi_{g} \in \operatorname{Aut}(G,\star,\circ) \} $. Then the G-stable regular subgroup N has
$ G^\prime = \{g \in G \mid \varphi_{g} \in \operatorname{Aut}(G,\star,\circ) \} $. Then the G-stable regular subgroup N has  $ |G|/|G^\prime| $ distinct ρ-conjugates.
$ |G|/|G^\prime| $ distinct ρ-conjugates.
Corollary 4.4. The G-stable regular subgroup N is normalized by  $ \rho(G) $ if and only if
$ \rho(G) $ if and only if  $ (G,\star,\circ) $ is a two-sided skew brace.
$ (G,\star,\circ) $ is a two-sided skew brace.
Proof of Theorem 4.2
By Proposition 4.1, we have  $ N_{g} = \varphi_{g}N\varphi_{g}^{-1} $, and the discussion above then implies that
$ N_{g} = \varphi_{g}N\varphi_{g}^{-1} $, and the discussion above then implies that  $ N_{g} = N $ if and only if
$ N_{g} = N $ if and only if  $ \varphi_{g} \in \operatorname{Aut}(G,\star,\circ) $. Hence, (i) and (ii) are equivalent.
$ \varphi_{g} \in \operatorname{Aut}(G,\star,\circ) $. Hence, (i) and (ii) are equivalent.
Now suppose that (ii) holds. By the left skew brace relation (3), for all  $ y,z \in G $, we have
$ y,z \in G $, we have
 \begin{equation*} g \circ (y \star z) = (g \circ y) \star g^{-1} \star (g \circ z). \end{equation*}
\begin{equation*} g \circ (y \star z) = (g \circ y) \star g^{-1} \star (g \circ z). \end{equation*} Since  $ \varphi_{g} \in \operatorname{Aut}(G,\star,\circ) $, so is
$ \varphi_{g} \in \operatorname{Aut}(G,\star,\circ) $, so is  $ \varphi_{g}^{-1} = \varphi_{\overline{g}} $. Applying this to the equation above yields
$ \varphi_{g}^{-1} = \varphi_{\overline{g}} $. Applying this to the equation above yields
 \begin{eqnarray*}
&& \varphi_{\overline{g}}(g \circ (y \star z)) = \varphi_{\overline{g}}((g \circ y) \star g^{-1} \star (g \circ z)) \\
& \Rightarrow & \varphi_{\overline{g}}(g \circ (y \star z)) = \varphi_{\overline{g}}(g \circ y) \star \varphi_{\overline{g}}(g^{-1}) \star \varphi_{\overline{g}}(g \circ z) \\
& \Rightarrow & \overline{g} \circ g \circ (y \star z) \circ g = (\overline{g} \circ g \circ y \circ g) \star \varphi_{\overline{g}}(g)^{-1} \star (\overline{g} \circ g \circ z \circ g) \\
& \Rightarrow & (y \star z) \circ g = (y \circ g) \star g^{-1} \star (z \circ g).
\end{eqnarray*}
\begin{eqnarray*}
&& \varphi_{\overline{g}}(g \circ (y \star z)) = \varphi_{\overline{g}}((g \circ y) \star g^{-1} \star (g \circ z)) \\
& \Rightarrow & \varphi_{\overline{g}}(g \circ (y \star z)) = \varphi_{\overline{g}}(g \circ y) \star \varphi_{\overline{g}}(g^{-1}) \star \varphi_{\overline{g}}(g \circ z) \\
& \Rightarrow & \overline{g} \circ g \circ (y \star z) \circ g = (\overline{g} \circ g \circ y \circ g) \star \varphi_{\overline{g}}(g)^{-1} \star (\overline{g} \circ g \circ z \circ g) \\
& \Rightarrow & (y \star z) \circ g = (y \circ g) \star g^{-1} \star (z \circ g).
\end{eqnarray*}Hence, (iii) holds.
Finally, suppose that (iii) holds. Then for all  $ y,z \in G $, we have
$ y,z \in G $, we have
 \begin{align*}
\varphi_{\overline{g}}(y \star z) & = \overline{g} \circ (y \star z) \circ g \\
& = \overline{g} \circ ( (y \circ g) \star g^{-1} \star (z \circ g))\quad \mbox{by (iii) } \\
& = (\overline{g} \circ y \circ g) \star \overline{g}^{-1} \star (\overline{g} \circ g^{-1}) \star \overline{g}^{-1} \star (\overline{g} \circ z \circ g) \quad\mbox{by Equation 3. }
\end{align*}
\begin{align*}
\varphi_{\overline{g}}(y \star z) & = \overline{g} \circ (y \star z) \circ g \\
& = \overline{g} \circ ( (y \circ g) \star g^{-1} \star (z \circ g))\quad \mbox{by (iii) } \\
& = (\overline{g} \circ y \circ g) \star \overline{g}^{-1} \star (\overline{g} \circ g^{-1}) \star \overline{g}^{-1} \star (\overline{g} \circ z \circ g) \quad\mbox{by Equation 3. }
\end{align*}Now by a well-known skew brace identity (see [Reference Guarneri and Vendramin13, Lemma 1.7, part (2)], for example), we have
 \begin{equation*} \overline{g}^{-1} \star (\overline{g} \circ g^{-1}) \star \overline{g}^{-1} = (\overline{g} \circ g)^{-1} = e_{G}; \end{equation*}
\begin{equation*} \overline{g}^{-1} \star (\overline{g} \circ g^{-1}) \star \overline{g}^{-1} = (\overline{g} \circ g)^{-1} = e_{G}; \end{equation*}applying this to the final line above yields
 \begin{equation*} \varphi_{\overline{g}}(y \star z) = (\overline{g} \circ y \circ g) \star (\overline{g} \circ z \circ g) = \varphi_{\overline{g}}(y) \star \varphi_{\overline{g}}(z), \end{equation*}
\begin{equation*} \varphi_{\overline{g}}(y \star z) = (\overline{g} \circ y \circ g) \star (\overline{g} \circ z \circ g) = \varphi_{\overline{g}}(y) \star \varphi_{\overline{g}}(z), \end{equation*}and so  $ \varphi_{\overline{g}} \in \operatorname{Aut}(G,\star,\circ) $. Therefore,
$ \varphi_{\overline{g}} \in \operatorname{Aut}(G,\star,\circ) $. Therefore,  $ \varphi_{g} \in \operatorname{Aut}(G,\star,\circ)) $, so (ii) holds.
$ \varphi_{g} \in \operatorname{Aut}(G,\star,\circ)) $, so (ii) holds.
5. Integral module structure
We now suppose that  $ L/K $ is a Galois extension of local or global fields (in any characteristic) with Galois group G. As discussed briefly in
$ L/K $ is a Galois extension of local or global fields (in any characteristic) with Galois group G. As discussed briefly in ![]() $\S$ 1, Hopf–Galois structures can be used in this context to study the structure of fractional ideals
$\S$ 1, Hopf–Galois structures can be used in this context to study the structure of fractional ideals  $ \mathfrak B $ of L. More precisely, if H is a Hopf algebra giving a Hopf–Galois structure on the extension, then L is a free H-module of rank 1 (this is a Hopf–Galois analogue of the classical normal basis theorem), and we seek integral analogues of this result. Each fractional ideal
$ \mathfrak B $ of L. More precisely, if H is a Hopf algebra giving a Hopf–Galois structure on the extension, then L is a free H-module of rank 1 (this is a Hopf–Galois analogue of the classical normal basis theorem), and we seek integral analogues of this result. Each fractional ideal  $ \mathfrak B $ of L has an associated order in H:
$ \mathfrak B $ of L has an associated order in H:
 \begin{equation*} {\mathfrak A}_{H}(\mathfrak B) = \{h \in H \mid h \cdot x \in \mathfrak B \quad \mbox{for all } x \in \mathfrak B \}, \end{equation*}
\begin{equation*} {\mathfrak A}_{H}(\mathfrak B) = \{h \in H \mid h \cdot x \in \mathfrak B \quad \mbox{for all } x \in \mathfrak B \}, \end{equation*}and we may study the structure of  $ \mathfrak B $ as an
$ \mathfrak B $ as an  $ {\mathfrak A}_{H}(\mathfrak B) $-module, with particular emphasis on determining criteria for it to be free (necessarily of rank 1). By construction,
$ {\mathfrak A}_{H}(\mathfrak B) $-module, with particular emphasis on determining criteria for it to be free (necessarily of rank 1). By construction,  $ {\mathfrak A}_{H}(\mathfrak B) $ is the largest subring of H for which
$ {\mathfrak A}_{H}(\mathfrak B) $ is the largest subring of H for which  $ \mathfrak B $ is a module, and it is the only order in H over which
$ \mathfrak B $ is a module, and it is the only order in H over which  $ \mathfrak B $ can possibly be free.
$ \mathfrak B $ can possibly be free.
The most famous results in this area are due to Byott [Reference Byott3], who exhibits Galois extensions  $ L/K $ of p-adic fields for which the valuation ring
$ L/K $ of p-adic fields for which the valuation ring  $ {\mathfrak O}_{L} $ is not free over
$ {\mathfrak O}_{L} $ is not free over  $ {\mathfrak A}_{K[G]}({\mathfrak O}_{L}) $ but is free over
$ {\mathfrak A}_{K[G]}({\mathfrak O}_{L}) $ but is free over  $ {\mathfrak A}_{H}({\mathfrak O}_{L}) $ for some other Hopf algebra H giving a Hopf–Galois structure on the extension. On the other hand, in [Reference Truman23] and [Reference Truman24], we show that a fractional ideal
$ {\mathfrak A}_{H}({\mathfrak O}_{L}) $ for some other Hopf algebra H giving a Hopf–Galois structure on the extension. On the other hand, in [Reference Truman23] and [Reference Truman24], we show that a fractional ideal  $ \mathfrak B $ of L is free over its associated order in a particular Hopf–Galois structure if and only if it is free over its associated order in the opposite Hopf–Galois structure. The main result of this section is in a similar vein. Recall that an ambiguous ideal of L is a fractional ideal of L that is invariant under the action of G. (In particular, the ring of algebraic integers
$ \mathfrak B $ of L is free over its associated order in a particular Hopf–Galois structure if and only if it is free over its associated order in the opposite Hopf–Galois structure. The main result of this section is in a similar vein. Recall that an ambiguous ideal of L is a fractional ideal of L that is invariant under the action of G. (In particular, the ring of algebraic integers  $ {\mathfrak O}_{L} $ is an ambiguous ideal of L, and if
$ {\mathfrak O}_{L} $ is an ambiguous ideal of L, and if  $ L/K $ is an extension of local fields, then every fractional ideal of L is an ambiguous ideal.) We will prove the following:
$ L/K $ is an extension of local fields, then every fractional ideal of L is an ambiguous ideal.) We will prove the following:
Theorem 5.1. Let  $ L[N]^{G} $ give a Hopf–Galois structure on
$ L[N]^{G} $ give a Hopf–Galois structure on  $ L/K $, and let
$ L/K $, and let  $ g \in G $. Then an ambiguous ideal
$ g \in G $. Then an ambiguous ideal  $ \mathfrak B $ of L is free over its associated order in
$ \mathfrak B $ of L is free over its associated order in  $ L[N]^{G} $ if and only if it is free over its associated order in
$ L[N]^{G} $ if and only if it is free over its associated order in  $ L[N_{g}]^{G} $.
$ L[N_{g}]^{G} $.
Corollary 5.2. An ambiguous ideal of L is free over its associated order in  $ L[N]^{G} $ if and only if it is free over its associated order in
$ L[N]^{G} $ if and only if it is free over its associated order in  $ L[N_{g}]^{G} $ for all
$ L[N_{g}]^{G} $ for all  $ g \in G $.
$ g \in G $.
The proof of Theorem 5.1 does not depend upon the fact that L is a field, so if  $ L/K $ is an extension of global fields and
$ L/K $ is an extension of global fields and ![]() $ \mathfrak{p} $ is a prime ideal of
$ \mathfrak{p} $ is a prime ideal of  $ {\mathfrak O}_{K} $, then we may apply the theorem to the Galois algebra
$ {\mathfrak O}_{K} $, then we may apply the theorem to the Galois algebra  $ L_{\mathfrak{p}} = K_{\mathfrak{p}} \otimes_{K} L $. This yields the following:
$ L_{\mathfrak{p}} = K_{\mathfrak{p}} \otimes_{K} L $. This yields the following:
Corollary 5.3. If  $ L/K $ is an extension of global fields then an ambiguous ideal of L is locally free over its associated order in
$ L/K $ is an extension of global fields then an ambiguous ideal of L is locally free over its associated order in  $ L[N]^{G} $ if and only if it is locally free over its associated order in
$ L[N]^{G} $ if and only if it is locally free over its associated order in  $ L[N_{g}]^{G} $ for all
$ L[N_{g}]^{G} $ for all  $ g \in G $.
$ g \in G $.
Proof of Theorem 5.1
Let  $ {\mathfrak A} $ denote the associated order of
$ {\mathfrak A} $ denote the associated order of  $ \mathfrak B $ in
$ \mathfrak B $ in  $ L[N]^{G} $, and let
$ L[N]^{G} $, and let  $ {\mathfrak A}_{g} $ denote the associated order of
$ {\mathfrak A}_{g} $ denote the associated order of  $ \mathfrak B $ in
$ \mathfrak B $ in  $ L[N_{g}]^{G} $. Suppose that
$ L[N_{g}]^{G} $. Suppose that  $ \mathfrak B $ is a free
$ \mathfrak B $ is a free  $ {\mathfrak A} $-module, with generator
$ {\mathfrak A} $-module, with generator  $ x \in \mathfrak B $. We shall show that
$ x \in \mathfrak B $. We shall show that  $ {\mathfrak A}_{g} = \phi({\mathfrak A}) $ and that
$ {\mathfrak A}_{g} = \phi({\mathfrak A}) $ and that  $ \mathfrak B $ is a free
$ \mathfrak B $ is a free  $ {\mathfrak A}_{g} $-module with generator g(x) (note that
$ {\mathfrak A}_{g} $-module with generator g(x) (note that  $ g(x) \in \mathfrak B $ because
$ g(x) \in \mathfrak B $ because  $ \mathfrak B $ is an ambiguous ideal of L).
$ \mathfrak B $ is an ambiguous ideal of L).
Since ϕ is an isomorphism of K-Hopf algebras, it is immediate that  $ \phi({\mathfrak A}) $ is an order in
$ \phi({\mathfrak A}) $ is an order in  $ L[N_{g}]^{G} $. Now let
$ L[N_{g}]^{G} $. Now let  $ y \in \mathfrak B $. Since
$ y \in \mathfrak B $. Since  $ \mathfrak B $ is an ambiguous ideal of L, we have
$ \mathfrak B $ is an ambiguous ideal of L, we have  $ g^{-1}(y) \in \mathfrak B $, and since
$ g^{-1}(y) \in \mathfrak B $, and since  $ \mathfrak B $ is a free
$ \mathfrak B $ is a free  $ {\mathfrak A} $-module with generator x, there exists a unique element
$ {\mathfrak A} $-module with generator x, there exists a unique element  $ z \in {\mathfrak A} $ such that
$ z \in {\mathfrak A} $ such that  $ z \cdot x = g^{-1}(y) $. Now consider the action of the element
$ z \cdot x = g^{-1}(y) $. Now consider the action of the element  $ \phi(z) \in \phi({\mathfrak A}) $ on the element
$ \phi(z) \in \phi({\mathfrak A}) $ on the element  $ g(x) \in \mathfrak B $. We have
$ g(x) \in \mathfrak B $. We have
 \begin{align*}
\phi(z) \cdot g(x) & = g ( z \cdot g^{-1}( g(x)) )\quad \mbox{by Proposition 3.2 }\\
& = g ( g^{-1}(y)) \\
& = y.
\end{align*}
\begin{align*}
\phi(z) \cdot g(x) & = g ( z \cdot g^{-1}( g(x)) )\quad \mbox{by Proposition 3.2 }\\
& = g ( g^{-1}(y)) \\
& = y.
\end{align*} Hence, given  $ y \in \mathfrak B $, there exists a unique element
$ y \in \mathfrak B $, there exists a unique element  $ \phi(z) $ of the order
$ \phi(z) $ of the order  $ \phi({\mathfrak A}) $ in
$ \phi({\mathfrak A}) $ in  $ L[N_{g}]^{G} $ such that
$ L[N_{g}]^{G} $ such that  $ \phi(z) \cdot g(x) = y $, and so
$ \phi(z) \cdot g(x) = y $, and so  $ \mathfrak B $ is a free
$ \mathfrak B $ is a free  $ \phi({\mathfrak A}) $-module with generator g(x). Since
$ \phi({\mathfrak A}) $-module with generator g(x). Since  $ {\mathfrak A}_{g} $ is the only order in
$ {\mathfrak A}_{g} $ is the only order in  $ L[N_{g}]^{G} $ over which
$ L[N_{g}]^{G} $ over which  $ \mathfrak B $ can be free, we conclude that
$ \mathfrak B $ can be free, we conclude that  $ \phi({\mathfrak A}) = {\mathfrak A}_{g} $.
$ \phi({\mathfrak A}) = {\mathfrak A}_{g} $.
For the converse statement, we replace N by Ng and g by g −1 in the argument above.
The interaction between Theorem 5.1 and the previously mentioned results concerning integral module structure with respect to opposite Hopf–Galois structures can cause one instance of freeness to propagate to a whole family.
Theorem 5.4. Consider the Hopf–Galois structures given by  $ L[N_{g}]^{G} $ and
$ L[N_{g}]^{G} $ and  $ L[N_{g}^{\rm opp}]^{G} $ as g runs through the elements of G. An ambiguous ideal
$ L[N_{g}^{\rm opp}]^{G} $ as g runs through the elements of G. An ambiguous ideal  $ \mathfrak B $ of L is either free over its associated orders in all of these Hopf–Galois structures or in none of them.
$ \mathfrak B $ of L is either free over its associated orders in all of these Hopf–Galois structures or in none of them.
Proof. By Corollary 5.2, the ambiguous ideal  $ \mathfrak B $ is free over its associated order in
$ \mathfrak B $ is free over its associated order in  $ L[N]^{G} $ if and only if it is free over its associated order in
$ L[N]^{G} $ if and only if it is free over its associated order in  $ L[N_{g}]^{G} $ for each
$ L[N_{g}]^{G} $ for each  $ g \in G $. Now applying [Reference Truman24, Theorem 1.2] to each
$ g \in G $. Now applying [Reference Truman24, Theorem 1.2] to each  $ L[N_{g}]^{G} $, we find that
$ L[N_{g}]^{G} $, we find that  $ \mathfrak B $ is free over its associated order in
$ \mathfrak B $ is free over its associated order in  $ L[N_{g}]^{G} $ if and only if it is free over its associated order in
$ L[N_{g}]^{G} $ if and only if it is free over its associated order in  $ L[N_{g}^{\rm opp}]^{G} $.
$ L[N_{g}^{\rm opp}]^{G} $.
Example 5.5. Let  $ p,q $ be odd primes with
$ p,q $ be odd primes with  $ p \equiv 1 \pmod{q} $ and let
$ p \equiv 1 \pmod{q} $ and let  $ L/K $ be a Galois extension of local or global fields whose Galois group G is isomorphic to the metacyclic group of order pq
$ L/K $ be a Galois extension of local or global fields whose Galois group G is isomorphic to the metacyclic group of order pq
 \begin{equation*} G = \langle s, t \mid s^{p}=t^{q}=e, \; t s t^{-1} = s^{d} \rangle \end{equation*}
\begin{equation*} G = \langle s, t \mid s^{p}=t^{q}=e, \; t s t^{-1} = s^{d} \rangle \end{equation*}as in Example 2.3. For  $ k=0, \ldots , p-1 $, let
$ k=0, \ldots , p-1 $, let
 \begin{equation*} N_{k} = \langle \lambda(s), \lambda(t) \rho(s^{k}t) \rangle. \end{equation*}
\begin{equation*} N_{k} = \langle \lambda(s), \lambda(t) \rho(s^{k}t) \rangle. \end{equation*} It is routine to verify that each N k is distinct, G-stable, regular and isomorphic to G. We find that  $ \rho(t) $ normalizes each N k and that
$ \rho(t) $ normalizes each N k and that  $ \rho(s)N_{k}\rho(s^{-1}) = N_{k+1-d} $. Hence, the subgroups N k are mutually ρ-conjugate, and by [Reference Koch and Truman16, Corollary 7.2], the same is true for their opposites
$ \rho(s)N_{k}\rho(s^{-1}) = N_{k+1-d} $. Hence, the subgroups N k are mutually ρ-conjugate, and by [Reference Koch and Truman16, Corollary 7.2], the same is true for their opposites  $ N_{k}^{\rm opp} $. Thus, we obtain a family of 2p Hopf–Galois structures on
$ N_{k}^{\rm opp} $. Thus, we obtain a family of 2p Hopf–Galois structures on  $ L/K $. By Theorem 5.4, an ambiguous ideal
$ L/K $. By Theorem 5.4, an ambiguous ideal  $ \mathfrak B $ of L is either free over its associated orders in all of these 2p Hopf–Galois structures or in none of them.
$ \mathfrak B $ of L is either free over its associated orders in all of these 2p Hopf–Galois structures or in none of them.
6. Interactions with existing constructions
In this section, we study the interactions between ρ-conjugation and various results concerning the construction and enumeration of Hopf–Galois structures on a Galois extension  $ L/K $. (We no longer assume that
$ L/K $. (We no longer assume that  $ L/K $ is an extension of local or global fields.)
$ L/K $ is an extension of local or global fields.)
6.1. Byott’s translation theorem and fixed-point free pairs of homomorphisms
Many results concerning the construction and enumeration of Hopf–Galois structures on separable field extensions employ Byott’s translation theorem [Reference Byott2] to address some of the combinatorial difficulties inherent to Greither–Pareigis theory. Specializing to the Galois case, Byott’s theorem may be summarized as follows: let M be an abstract group of the same order as G and consider regular embeddings  $ \alpha : M \rightarrow \operatorname{Perm}(G) $ (i.e., embeddings with regular image). Such an embedding yields a bijection
$ \alpha : M \rightarrow \operatorname{Perm}(G) $ (i.e., embeddings with regular image). Such an embedding yields a bijection  $ a : M \rightarrow G $ defined by
$ a : M \rightarrow G $ defined by  $ a(\mu) = \alpha(\mu)[e_{G}] $ for all
$ a(\mu) = \alpha(\mu)[e_{G}] $ for all  $ \mu \in M $, and thence a regular embedding
$ \mu \in M $, and thence a regular embedding  $ \beta : G \rightarrow \operatorname{Perm}(M) $ defined by
$ \beta : G \rightarrow \operatorname{Perm}(M) $ defined by  $ \beta(g) = a^{-1} \lambda(g) a $ for all
$ \beta(g) = a^{-1} \lambda(g) a $ for all  $ g \in G $. Byott’s theorem asserts that this construction yields a bijection between regular embeddings of M into
$ g \in G $. Byott’s theorem asserts that this construction yields a bijection between regular embeddings of M into  $ \operatorname{Perm}(G) $ and regular embeddings of G into
$ \operatorname{Perm}(G) $ and regular embeddings of G into  $ \operatorname{Perm}(M) $. Furthermore,
$ \operatorname{Perm}(M) $. Furthermore,  $ \alpha(M) $ is G-stable if and only if
$ \alpha(M) $ is G-stable if and only if  $ \beta(G) \subseteq \operatorname{Hol}(M) = \lambda(M) \operatorname{Aut}(M) \subseteq \operatorname{Perm}(M) $, and
$ \beta(G) \subseteq \operatorname{Hol}(M) = \lambda(M) \operatorname{Aut}(M) \subseteq \operatorname{Perm}(M) $, and  $ \alpha(M)=\alpha^\prime(M) $ if and only if there exists
$ \alpha(M)=\alpha^\prime(M) $ if and only if there exists  $ \theta \in \operatorname{Aut}(M) $ such that
$ \theta \in \operatorname{Aut}(M) $ such that  $ \beta^\prime(g) = \theta \beta(g) \theta^{-1} $ for all
$ \beta^\prime(g) = \theta \beta(g) \theta^{-1} $ for all  $ g \in G $.
$ g \in G $.
We can study ρ-conjugate Hopf–Galois structures from this perspective using the reformulation expressed in Proposition 4.1. Given a regular embedding  $ \alpha : M \rightarrow \operatorname{Perm}(G) $ and an element
$ \alpha : M \rightarrow \operatorname{Perm}(G) $ and an element  $ g \in G $, the map
$ g \in G $, the map  $ \alpha_{g} : M \rightarrow \operatorname{Perm}(G) $ defined by
$ \alpha_{g} : M \rightarrow \operatorname{Perm}(G) $ defined by  $ \alpha_{g}(\mu) = \varphi_{g} \alpha(\mu) \varphi_{g}^{-1} $ for all
$ \alpha_{g}(\mu) = \varphi_{g} \alpha(\mu) \varphi_{g}^{-1} $ for all  $ \mu \in M $ is a regular embedding with image
$ \mu \in M $ is a regular embedding with image  $ \alpha(M)_{g} $. We then find the following:
$ \alpha(M)_{g} $. We then find the following:
Lemma 6.1. Let  $ \beta : G \rightarrow \operatorname{Hol}(M) $ be the embedding corresponding to α. Then the embedding
$ \beta : G \rightarrow \operatorname{Hol}(M) $ be the embedding corresponding to α. Then the embedding  $ \beta_{g} : G \rightarrow \operatorname{Hol}(M) $ corresponding to αg is given by
$ \beta_{g} : G \rightarrow \operatorname{Hol}(M) $ corresponding to αg is given by  $ \beta\!_{g} = \beta \varphi\!_{g^{-1}} $.
$ \beta\!_{g} = \beta \varphi\!_{g^{-1}} $.
Proof. The bijection  $ a_{g} : M \rightarrow G $ corresponding to αg is given by
$ a_{g} : M \rightarrow G $ corresponding to αg is given by
 \begin{equation*} a_{g}(\mu) = \varphi_{g} \alpha(\mu) \varphi_{g^{-1}}[e_{G}] = \varphi_{g} \alpha(\mu)[e_{G}] = \varphi_{g} a(\mu). \end{equation*}
\begin{equation*} a_{g}(\mu) = \varphi_{g} \alpha(\mu) \varphi_{g^{-1}}[e_{G}] = \varphi_{g} \alpha(\mu)[e_{G}] = \varphi_{g} a(\mu). \end{equation*} Hence, for  $ h \in G $ and
$ h \in G $ and  $ \mu \in M $ we have
$ \mu \in M $ we have
 \begin{align*}
\beta_{g}(h)[\mu] & = a^{-1} \varphi_{g}^{-1} \lambda_{G}(h) \varphi_{g} a [\mu] \\
& = a^{-1} \varphi_{g}^{-1} (h g a [\mu] g^{-1}) \\
& = a^{-1}( g^{-1} h g a [\mu] g^{-1} g )\\
& = a^{-1} \lambda(\varphi_{g^{-1}}(h)) a [\mu] \\
& = \beta(\varphi_{g^{-1}}(h))[\mu].
\end{align*}
\begin{align*}
\beta_{g}(h)[\mu] & = a^{-1} \varphi_{g}^{-1} \lambda_{G}(h) \varphi_{g} a [\mu] \\
& = a^{-1} \varphi_{g}^{-1} (h g a [\mu] g^{-1}) \\
& = a^{-1}( g^{-1} h g a [\mu] g^{-1} g )\\
& = a^{-1} \lambda(\varphi_{g^{-1}}(h)) a [\mu] \\
& = \beta(\varphi_{g^{-1}}(h))[\mu].
\end{align*} Hence  $ \beta\!_{g} = \beta \varphi\!_{g^{-1}} $, as claimed.
$ \beta\!_{g} = \beta \varphi\!_{g^{-1}} $, as claimed.
As an application of Lemma 6.1, we study the interaction between ρ-conjugation and Hopf–Galois structures on Galois extensions arising from fixed point free pairs of homomorphisms, due to Byott and Childs [Reference Byott and Childs5]. Given finite groups  $ G,M $ of the same order, a pair of homomorphisms
$ G,M $ of the same order, a pair of homomorphisms  $ f_{1}, f_{2} : G \rightarrow M $ is called fixed point free if the only element
$ f_{1}, f_{2} : G \rightarrow M $ is called fixed point free if the only element  $ h \in G $ for which
$ h \in G $ for which  $ f_{1}(h)=f_{2}(h) $ is
$ f_{1}(h)=f_{2}(h) $ is  $ h = e_{G} $. Given such a pair of homomorphisms, the map
$ h = e_{G} $. Given such a pair of homomorphisms, the map  $ \beta_{f_{1},f_{2}} : G \rightarrow \operatorname{Hol}(M) $ defined by
$ \beta_{f_{1},f_{2}} : G \rightarrow \operatorname{Hol}(M) $ defined by
 \begin{equation*} \beta_{f_{1},f_{2}}(h) = \lambda(f_{1}(h)) \rho(f_{2}(h))\quad \mbox{for all } h \in G \end{equation*}
\begin{equation*} \beta_{f_{1},f_{2}}(h) = \lambda(f_{1}(h)) \rho(f_{2}(h))\quad \mbox{for all } h \in G \end{equation*}is a regular embedding of G into  $ \operatorname{Hol}(M) $ (where
$ \operatorname{Hol}(M) $ (where  $ \lambda, \rho $ denote the left and right regular representations of M) and therefore yields a Hopf–Galois structure of type M on a Galois extension with Galois group G. Applying Lemma 6.1, we obtain immediately the following:
$ \lambda, \rho $ denote the left and right regular representations of M) and therefore yields a Hopf–Galois structure of type M on a Galois extension with Galois group G. Applying Lemma 6.1, we obtain immediately the following:
Proposition 6.2. Let  $ f_{1},f_{2} : G \rightarrow \operatorname{Hol}(M) $ be a fixed point free pair of homomorphisms, with corresponding regular embedding
$ f_{1},f_{2} : G \rightarrow \operatorname{Hol}(M) $ be a fixed point free pair of homomorphisms, with corresponding regular embedding  $ \beta : G \rightarrow \operatorname{Hol}(M) $, and let
$ \beta : G \rightarrow \operatorname{Hol}(M) $, and let  $ g \in G $. For
$ g \in G $. For  $ i=1,2 $ let
$ i=1,2 $ let  $ f_{i,g} = f_{i} \varphi_{g} $. Then
$ f_{i,g} = f_{i} \varphi_{g} $. Then  $ f_{1,g}, f_{2,g} $ is a fixed point free pair of homomorphisms, and the corresponding embedding of G into
$ f_{1,g}, f_{2,g} $ is a fixed point free pair of homomorphisms, and the corresponding embedding of G into  $ \operatorname{Hol}(M) $ is β g.
$ \operatorname{Hol}(M) $ is β g.
Example 6.3. Let n be an even natural number and let  $ G = \langle r,s \rangle $ and
$ G = \langle r,s \rangle $ and  $ M = \langle \mu, \pi \rangle $ both be isomorphic to the dihedral group of order 2n (here
$ M = \langle \mu, \pi \rangle $ both be isomorphic to the dihedral group of order 2n (here  $ r, \mu $ have order n and
$ r, \mu $ have order n and  $ s, \pi $ have order 2). Fix
$ s, \pi $ have order 2). Fix  $ 0 \leq k \leq n/2-1 $ and define
$ 0 \leq k \leq n/2-1 $ and define  $ f_{1} : G \rightarrow M $ by
$ f_{1} : G \rightarrow M $ by  $ f_{1}(r^{i}s^{j}) = \mu^{i}\pi^{j} $ and
$ f_{1}(r^{i}s^{j}) = \mu^{i}\pi^{j} $ and  $ f_{2}: G \rightarrow M $ by
$ f_{2}: G \rightarrow M $ by  $ f_{2}(r^{i}s^{j}) = (\mu^{2k}\pi)^{i} $. Then
$ f_{2}(r^{i}s^{j}) = (\mu^{2k}\pi)^{i} $. Then  $ f_{1}, f_{2} $ form a fixed point free pair of homomorphisms, and the corresponding embedding
$ f_{1}, f_{2} $ form a fixed point free pair of homomorphisms, and the corresponding embedding  $ \beta : G \hookrightarrow \operatorname{Hol}(M) $ is given by
$ \beta : G \hookrightarrow \operatorname{Hol}(M) $ is given by
 \begin{equation*} \beta(r) = \lambda(\mu) \rho(\mu^{2k}\pi), \quad \beta(s) = \lambda(\pi). \end{equation*}
\begin{equation*} \beta(r) = \lambda(\mu) \rho(\mu^{2k}\pi), \quad \beta(s) = \lambda(\pi). \end{equation*} (Here  $ \lambda, \rho $ denote the left and right regular representations of M.) We find that composing β with φ s results in an equivalent embedding, whereas composing with each
$ \lambda, \rho $ denote the left and right regular representations of M.) We find that composing β with φ s results in an equivalent embedding, whereas composing with each  $ \varphi_{r^{\ell}} $ for
$ \varphi_{r^{\ell}} $ for  $ 0 \leq \ell \leq n/2-1 $ gives an inequivalent embedding. Thus, we obtain
$ 0 \leq \ell \leq n/2-1 $ gives an inequivalent embedding. Thus, we obtain  $ n/2 $ mutually ρ-conjugate Hopf–Galois structures of dihedral type on a Galois extension with Galois group isomorphic to G. In fact, these are the Hopf–Galois structures described in Example 2.4.
$ n/2 $ mutually ρ-conjugate Hopf–Galois structures of dihedral type on a Galois extension with Galois group isomorphic to G. In fact, these are the Hopf–Galois structures described in Example 2.4.
6.2. Hopf–Galois structures arising from abelian maps
An abelian map is a group endomorphism with abelian image. In [Reference Childs7], Childs shows that fixed point free abelian maps on a finite group G yield a family of Hopf–Galois structures on a Galois extension  $ L/K $ with Galois group G. This approach is generalized by Koch [Reference Koch14], as follows: let
$ L/K $ with Galois group G. This approach is generalized by Koch [Reference Koch14], as follows: let  $ \psi : G \rightarrow G $ be an abelian map, and for each
$ \psi : G \rightarrow G $ be an abelian map, and for each  $ h \in G, $ define
$ h \in G, $ define  $ \eta_{\psi}(h) \in \operatorname{Perm}(G) $ by
$ \eta_{\psi}(h) \in \operatorname{Perm}(G) $ by
 \begin{equation*} \eta_{\psi}(h) = \lambda(h \psi(h)^{-1}) \rho(\psi(h)^{-1}). \end{equation*}
\begin{equation*} \eta_{\psi}(h) = \lambda(h \psi(h)^{-1}) \rho(\psi(h)^{-1}). \end{equation*} Then  $ N_{\psi} = \{\eta_{\psi}(h) \mid h \in G \} $ is a G-stable regular subgroup of
$ N_{\psi} = \{\eta_{\psi}(h) \mid h \in G \} $ is a G-stable regular subgroup of  $ \operatorname{Perm}(G) $ [Reference Koch14, Theorem 3.1], which therefore corresponds to a Hopf–Galois structure on
$ \operatorname{Perm}(G) $ [Reference Koch14, Theorem 3.1], which therefore corresponds to a Hopf–Galois structure on  $ L/K $.
$ L/K $.
Proposition 6.4. Let ψ be an abelian map on G and let N ψ be the corresponding G-stable regular subgroup of  $ \operatorname{Perm}(G) $. Then for each
$ \operatorname{Perm}(G) $. Then for each  $ g \in G $ the subgroup
$ g \in G $ the subgroup  $ N_{\psi,g} $ arises from an abelian map on G.
$ N_{\psi,g} $ arises from an abelian map on G.
Proof. By Proposition 4.1, we have  $ N_{\psi,g} = \varphi_{g} N_{\psi} \varphi_{g}^{-1} $. It is shown in [Reference Koch and Truman16, Proposition 5.1] that if ψ is an abelian map on G and
$ N_{\psi,g} = \varphi_{g} N_{\psi} \varphi_{g}^{-1} $. It is shown in [Reference Koch and Truman16, Proposition 5.1] that if ψ is an abelian map on G and  $ \varphi \in \operatorname{Aut}(G) $, then the map
$ \varphi \in \operatorname{Aut}(G) $, then the map  $ \varphi \psi \varphi^{-1} $ is also an abelian map on G and
$ \varphi \psi \varphi^{-1} $ is also an abelian map on G and  $ N_{\varphi \psi \varphi^{-1}} = \varphi N_{\psi} \varphi^{-1} $. Thus,
$ N_{\varphi \psi \varphi^{-1}} = \varphi N_{\psi} \varphi^{-1} $. Thus,  $ N_{\psi,g} $ arises from the abelian map
$ N_{\psi,g} $ arises from the abelian map  $ \psi^\prime = \varphi_{g} \psi \varphi_{g}^{-1} $ on G.
$ \psi^\prime = \varphi_{g} \psi \varphi_{g}^{-1} $ on G.
Example 6.5. Let  $ n \geq 5 $ and suppose that G is isomorphic to the symmetric group S n. In [Reference Koch14, Example 3.7], Koch shows that the abelian maps on G correspond bijectively with elements
$ n \geq 5 $ and suppose that G is isomorphic to the symmetric group S n. In [Reference Koch14, Example 3.7], Koch shows that the abelian maps on G correspond bijectively with elements  $ x \in S_{n} $ of order at most 2, as follows:
$ x \in S_{n} $ of order at most 2, as follows:
 \begin{equation*} \psi_{x}(t) = \left\{\begin{array}{ll} e & \mbox{if } t \in A_{n} \\ x & \mbox{if } t \not \in A_{n} \end{array} \right. \end{equation*}
\begin{equation*} \psi_{x}(t) = \left\{\begin{array}{ll} e & \mbox{if } t \in A_{n} \\ x & \mbox{if } t \not \in A_{n} \end{array} \right. \end{equation*} Let Nx be the G-stable regular subgroup arising from the abelian map ψ x. Applying Proposition 6.4, we see that for each  $ g \in G $, the subgroup
$ g \in G $, the subgroup  $ N_{\psi,g} $ arises from the abelian map
$ N_{\psi,g} $ arises from the abelian map  $ \varphi_{g} \psi_{x} \varphi_{g}^{-1} $, which behaves as follows
$ \varphi_{g} \psi_{x} \varphi_{g}^{-1} $, which behaves as follows
 \begin{equation*} \varphi_{g} \psi_{x} \varphi_{g}^{-1}(t) = \left\{\begin{array}{ll} e & \mbox{if } t \in A_{n} \\ gxg^{-1} & \mbox{if } t \not \in A_{n} \end{array} \right. \end{equation*}
\begin{equation*} \varphi_{g} \psi_{x} \varphi_{g}^{-1}(t) = \left\{\begin{array}{ll} e & \mbox{if } t \in A_{n} \\ gxg^{-1} & \mbox{if } t \not \in A_{n} \end{array} \right. \end{equation*} Thus,  $ \varphi_{g} \psi_{x} \varphi_{g}^{-1} = \psi_{g x g^{-1}} $, and so the ρ-conjugate subgroups correspond to conjugacy classes of elements of order at most 2 in G. In particular, the subgroups arising from transpositions are mutually ρ-conjugate.
$ \varphi_{g} \psi_{x} \varphi_{g}^{-1} = \psi_{g x g^{-1}} $, and so the ρ-conjugate subgroups correspond to conjugacy classes of elements of order at most 2 in G. In particular, the subgroups arising from transpositions are mutually ρ-conjugate.
6.3. Induced Hopf–Galois structures
The method of induced Hopf–Galois structures is due to Crespo, Rio and Vela [Reference Crespo, Rio and Vela10]: if T is a subgroup of G having a normal complement S in G, then given a Hopf–Galois structure on  $ L/L^{T} $ and a Hopf–Galois structure on
$ L/L^{T} $ and a Hopf–Galois structure on  $ L^{T}/K $, we can induce a Hopf–Galois structure on
$ L^{T}/K $, we can induce a Hopf–Galois structure on  $ L/K $. Note that T need not be a normal subgroup of G, and so the extension
$ L/K $. Note that T need not be a normal subgroup of G, and so the extension  $ L^{T}/K $ need not be a Galois extension. In order to study Hopf–Galois structures on this extension, we need the full strength of the Greither–Pareigis classification. In this context, it implies that the Hopf–Galois structures on
$ L^{T}/K $ need not be a Galois extension. In order to study Hopf–Galois structures on this extension, we need the full strength of the Greither–Pareigis classification. In this context, it implies that the Hopf–Galois structures on  $ L^{T}/K $ correspond bijectively with regular subgroups of
$ L^{T}/K $ correspond bijectively with regular subgroups of  $ \operatorname{Perm}(G/T) $ (the permutation group of the left coset space) that are G-stable in the sense that they are normalized by the image of G under the left translation map
$ \operatorname{Perm}(G/T) $ (the permutation group of the left coset space) that are G-stable in the sense that they are normalized by the image of G under the left translation map  $ \lambda : G \rightarrow \operatorname{Perm}(G/T) $ defined by
$ \lambda : G \rightarrow \operatorname{Perm}(G/T) $ defined by  $ \lambda(h)[xT] = hxT $ for all
$ \lambda(h)[xT] = hxT $ for all  $ h \in G $ and
$ h \in G $ and  $ xT \in G/T $.
$ xT \in G/T $.
Given a G-stable regular subgroup  $ A \subset \operatorname{Perm}(G/T) $ and a T-stable regular subgroup
$ A \subset \operatorname{Perm}(G/T) $ and a T-stable regular subgroup  $ B \subset \operatorname{Perm}(T) $, we use the fact that G is the semidirect product of S and T to identify A with a regular subgroup of
$ B \subset \operatorname{Perm}(T) $, we use the fact that G is the semidirect product of S and T to identify A with a regular subgroup of  $ \operatorname{Perm}(S) $ and then to define a map
$ \operatorname{Perm}(S) $ and then to define a map  $ \eta : A \times B \rightarrow \operatorname{Perm}(G) $ by
$ \eta : A \times B \rightarrow \operatorname{Perm}(G) $ by
 \begin{equation*} \eta(a,b)[s t] = a[s] b[t] \quad \mbox{for all } s \in S\ \mbox{and } t \in T. \end{equation*}
\begin{equation*} \eta(a,b)[s t] = a[s] b[t] \quad \mbox{for all } s \in S\ \mbox{and } t \in T. \end{equation*} It is easy to see that η is an embedding whose image N is a regular subgroup of  $ \operatorname{Perm}(G) $ isomorphic to A × B; [Reference Crespo, Rio and Vela10, Theorem 3] shows that N is G-stable and hence corresponds to a Hopf–Galois structure on
$ \operatorname{Perm}(G) $ isomorphic to A × B; [Reference Crespo, Rio and Vela10, Theorem 3] shows that N is G-stable and hence corresponds to a Hopf–Galois structure on  $ L/K $. We say that the subgroup N is induced from the subgroups A and B and that the Hopf–Galois structure on
$ L/K $. We say that the subgroup N is induced from the subgroups A and B and that the Hopf–Galois structure on  $ L/K $ corresponding to N is induced from those corresponding to A on
$ L/K $ corresponding to N is induced from those corresponding to A on  $ L^{T}/K $ and B on
$ L^{T}/K $ and B on  $ L/L^{T} $.
$ L/L^{T} $.
Now let  $ g \in G $ and let
$ g \in G $ and let  $ \varphi = \varphi_{g} $ be the inner automorphism corresponding to g. Since S is normal in G, we have
$ \varphi = \varphi_{g} $ be the inner automorphism corresponding to g. Since S is normal in G, we have  $ \varphi(S) = S $, and it is easily shown that G is the semidirect product of S and
$ \varphi(S) = S $, and it is easily shown that G is the semidirect product of S and  $ \varphi(T) $. We shall show that the Hopf–Galois structure corresponding to Ng is induced from certain Hopf–Galois structures on
$ \varphi(T) $. We shall show that the Hopf–Galois structure corresponding to Ng is induced from certain Hopf–Galois structures on  $ L^{\varphi(T)}/K $ and
$ L^{\varphi(T)}/K $ and  $ L/L^{\varphi(T)} $.
$ L/L^{\varphi(T)} $.
Lemma 6.6. Let  $ g \in G $ and
$ g \in G $ and  $ \varphi = \varphi_{g} $. Then,
$ \varphi = \varphi_{g} $. Then,
i)
 $ \varphi A \varphi^{-1} $ identifies naturally with a G-stable regular subgroup of
$ \varphi A \varphi^{-1} $ identifies naturally with a G-stable regular subgroup of  $ \operatorname{Perm}(G / \varphi(T)) $;
$ \operatorname{Perm}(G / \varphi(T)) $;ii)
 $ \varphi B \varphi^{-1} $ identifies naturally with a
$ \varphi B \varphi^{-1} $ identifies naturally with a  $ \varphi(T) $-stable regular subgroup of
$ \varphi(T) $-stable regular subgroup of  $ \operatorname{Perm}(\varphi(T)) $.
$ \operatorname{Perm}(\varphi(T)) $.
Proof. To show (i), let  $ a \in A $ and
$ a \in A $ and  $ s\varphi(T) \in G / \varphi(T) $ (with
$ s\varphi(T) \in G / \varphi(T) $ (with  $ s \in S $). Then
$ s \in S $). Then
 \begin{equation*} \varphi a \varphi^{-1} [s\varphi(T)] = \varphi a [ \varphi^{-1}(s) T ] = s^\prime \varphi(T) \end{equation*}
\begin{equation*} \varphi a \varphi^{-1} [s\varphi(T)] = \varphi a [ \varphi^{-1}(s) T ] = s^\prime \varphi(T) \end{equation*}for some element  $ s^\prime \in S $. Hence,
$ s^\prime \in S $. Hence,  $ \varphi A \varphi^{-1} $ permutes
$ \varphi A \varphi^{-1} $ permutes  $ G / \varphi(T) $, and this action is regular because A acts regularly on
$ G / \varphi(T) $, and this action is regular because A acts regularly on  $ G/T $. To show G-stability, let
$ G/T $. To show G-stability, let  $ h \in G $ and consider
$ h \in G $ and consider
 \begin{align*}
\lambda(h) \varphi a \varphi^{-1} \lambda(h^{-1}) [s \varphi(T)] & = h \varphi a [ \varphi^{-1}(h^{-1}) \varphi^{-1}(s) T ] \\
& = \varphi( \varphi^{-1}(h) a [ \varphi^{-1}(h^{-1}) \varphi^{-1}(s) T ] \\
& = \varphi( \varphi^{-1}(h) a \varphi^{-1}(h^{-1}) \varphi^{-1} [s \varphi(T) ] \\
& = \varphi a^\prime \varphi^{-1} [ s \varphi(T) ]
\end{align*}
\begin{align*}
\lambda(h) \varphi a \varphi^{-1} \lambda(h^{-1}) [s \varphi(T)] & = h \varphi a [ \varphi^{-1}(h^{-1}) \varphi^{-1}(s) T ] \\
& = \varphi( \varphi^{-1}(h) a [ \varphi^{-1}(h^{-1}) \varphi^{-1}(s) T ] \\
& = \varphi( \varphi^{-1}(h) a \varphi^{-1}(h^{-1}) \varphi^{-1} [s \varphi(T) ] \\
& = \varphi a^\prime \varphi^{-1} [ s \varphi(T) ]
\end{align*}for some  $ a^\prime \in A $, since A is G-stable. Hence,
$ a^\prime \in A $, since A is G-stable. Hence,  $ \varphi A \varphi^{-1} $ is G-stable. The proof of (ii) is similar.
$ \varphi A \varphi^{-1} $ is G-stable. The proof of (ii) is similar.
Note that if  $ g \in T $, then the regular subgroup
$ g \in T $, then the regular subgroup  $ \varphi B \varphi^{-1} $ of
$ \varphi B \varphi^{-1} $ of  $ \operatorname{Perm}(T) $ is ρ-conjugate to B.
$ \operatorname{Perm}(T) $ is ρ-conjugate to B.
Proposition 6.7. For A, B, N and g as above, the subgroup  $ N_{g} \leq \operatorname{Perm}(G) $ is induced from
$ N_{g} \leq \operatorname{Perm}(G) $ is induced from  $ \varphi A \varphi^{-1} \leq \operatorname{Perm}(G/\varphi(T)) $ and
$ \varphi A \varphi^{-1} \leq \operatorname{Perm}(G/\varphi(T)) $ and  $ \varphi B \varphi^{-1} \leq \operatorname{Perm}(\varphi(T)) $.
$ \varphi B \varphi^{-1} \leq \operatorname{Perm}(\varphi(T)) $.
Proof. Let  $ h \in G $, and write
$ h \in G $, and write  $ h = s\varphi(t) $ with
$ h = s\varphi(t) $ with  $ s \in S $ and
$ s \in S $ and  $ t \in T $. Now let
$ t \in T $. Now let  $ \eta = \eta(a,b) \in N $ and consider
$ \eta = \eta(a,b) \in N $ and consider
 \begin{align*}
\varphi \eta \varphi^{-1} [s\varphi(t)] & = \varphi \eta [\varphi^{-1}(s) t] \\
& = \varphi (a[\varphi^{-1}(s)] b[t])\quad (\mathrm{note that}\ \varphi^{-1}(s) \in S) \\
& = (\varphi a[\varphi^{-1}(s)]) (\varphi b[t]) \\
& = (\varphi a[\varphi^{-1}(s)]) (\varphi b[\varphi^{-1}( \varphi(t))]) \\
& = \eta( \varphi a \varphi^{-1}, \varphi b \varphi^{-1}) [s \varphi(t)].
\end{align*}
\begin{align*}
\varphi \eta \varphi^{-1} [s\varphi(t)] & = \varphi \eta [\varphi^{-1}(s) t] \\
& = \varphi (a[\varphi^{-1}(s)] b[t])\quad (\mathrm{note that}\ \varphi^{-1}(s) \in S) \\
& = (\varphi a[\varphi^{-1}(s)]) (\varphi b[t]) \\
& = (\varphi a[\varphi^{-1}(s)]) (\varphi b[\varphi^{-1}( \varphi(t))]) \\
& = \eta( \varphi a \varphi^{-1}, \varphi b \varphi^{-1}) [s \varphi(t)].
\end{align*} Hence,  $ N_{g} \leq \operatorname{Perm}(G) $ is induced from
$ N_{g} \leq \operatorname{Perm}(G) $ is induced from  $ \varphi A \varphi^{-1} \leq \operatorname{Perm}(G/\varphi(T)) $ and
$ \varphi A \varphi^{-1} \leq \operatorname{Perm}(G/\varphi(T)) $ and  $ \varphi B \varphi^{-1} \leq \operatorname{Perm}(\varphi(T)) $.
$ \varphi B \varphi^{-1} \leq \operatorname{Perm}(\varphi(T)) $.
We remark that the argument of Proposition 6.7 remains valid if φ is any automorphism of G that preserves S.
Example 6.8. Let  $ p,q $ be odd primes with
$ p,q $ be odd primes with  $ p \equiv 1 \pmod{q} $ and let
$ p \equiv 1 \pmod{q} $ and let  $ L/K $ be a Galois extension of fields whose Galois group G is isomorphic to the metacyclic group of order pq
$ L/K $ be a Galois extension of fields whose Galois group G is isomorphic to the metacyclic group of order pq
 \begin{equation*} G = \langle s, t \mid s^{p}=t^{q}=e, \; t s t^{-1} = s^{d} \rangle \end{equation*}
\begin{equation*} G = \langle s, t \mid s^{p}=t^{q}=e, \; t s t^{-1} = s^{d} \rangle \end{equation*}as in Example 2.3. Let  $ T = \langle t \rangle $ and
$ T = \langle t \rangle $ and  $ S = \langle s \rangle $. Then the conjugates of T are precisely the subgroups
$ S = \langle s \rangle $. Then the conjugates of T are precisely the subgroups  $ T_{i} = s^{i} T s^{-i} $, and S is a normal complement to each of these subgroups in G. For each i, the extensions
$ T_{i} = s^{i} T s^{-i} $, and S is a normal complement to each of these subgroups in G. For each i, the extensions  $ L/L^{T_{i}} $ and
$ L/L^{T_{i}} $ and  $ L^{T_{i}}/K $ have prime degree and each admits a unique Hopf–Galois structure (note that each
$ L^{T_{i}}/K $ have prime degree and each admits a unique Hopf–Galois structure (note that each  $ L^{T_{i}}/K $ is non-normal). In each case, we can induce a Hopf–Galois structure on
$ L^{T_{i}}/K $ is non-normal). In each case, we can induce a Hopf–Galois structure on  $ L/K $ from these, and by Proposition 6.7, we obtain a family of p mutually ρ-conjugate Hopf–Galois structures on
$ L/K $ from these, and by Proposition 6.7, we obtain a family of p mutually ρ-conjugate Hopf–Galois structures on  $ L/K $. Referring to Example 2.3, we see that these are all of the Hopf–Galois structures of cyclic type admitted by
$ L/K $. Referring to Example 2.3, we see that these are all of the Hopf–Galois structures of cyclic type admitted by  $ L/K $.
$ L/K $.
Acknowledgements
I am grateful to Prof. Anna Rio for the suggestion to study the interaction between ρ-conjugate Hopf–Galois structures and induced Hopf–Galois structures, to Prof. Alan Koch for comments on an early draft of this paper and to the anonymous referee for numerous improvements to the exposition and interpretation of the results.
Competing Interests
The author declares that there are no competing interests.