Article contents
On a Boolean algebra of projections constructed by Dieudonné
Published online by Cambridge University Press: 20 January 2009
Extract
Dieudonné (4) has constructed an example of a Banach space X and a complete Boolean algebra 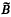 of projections on X such that
of projections on X such that 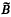 has uniform multiplicity two, but for no choice of x1, x2 in X and non-zero E in
has uniform multiplicity two, but for no choice of x1, x2 in X and non-zero E in 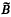 is EX the direct sum of the cyclic subspaces clm {Ex1:E∈
is EX the direct sum of the cyclic subspaces clm {Ex1:E∈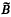 } and clm {Ex2:E∈
} and clm {Ex2:E∈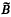 }. Tzafriri observed that it could be deduced from Corollary 4 (9, p. 221) that the commutant
}. Tzafriri observed that it could be deduced from Corollary 4 (9, p. 221) that the commutant 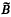 ′ of
′ of 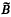 is equal to A(
is equal to A(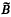 ), the algebra of operators generated by
), the algebra of operators generated by 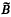 in the uniform operator topology. A study of (3) suggested the direct proof of the second property given in this note. From this there follows a simple proof that
in the uniform operator topology. A study of (3) suggested the direct proof of the second property given in this note. From this there follows a simple proof that 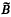 has the first property.
has the first property.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1969
References
REFERENCES
- 1
- Cited by


