Hostname: page-component-78c5997874-dh8gc Total loading time: 0 Render date: 2024-11-08T14:30:59.041Z Has data issue: false hasContentIssue false
  • English
  • Français

Notes on an orthocentric triangle

Published online by Cambridge University Press:  20 January 2009

R. Tucker
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

1. In the accompanying figure DEF is the pedal triangle of ABC and P, Q, R are the orthocentres of AFE, BDF, CED.

AP, BQ, CR evidently meet in the circumcentre, O, of ABC, which is the orthocentre of PQR,

2. Now the circumradius of AFE(ρa) = RcosA,

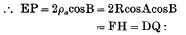
hence the sides of PQR are equal and parallel to the sides of DEF, i.e., the triangles are congruent, and their centre of perspective, L, bisects DP, EQ, FR.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 12 , February 1893 , pp. 118 - 119
Copyright
Copyright © Edinburgh Mathematical Society 1893

References

* See Proccedings, London Mathematical Society, Vol. xv., Appendix.)