Note on the theory of the rolling of one rigid surface on another

Extract

Take first the case of a surface S rolling on a plane, the instantaneous axis of rotation being a line in the tangent plane at the point of contact. Take that line as x-axis, and the normal as z-axis, and let the equation to the surface be

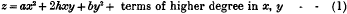
Then if p is small, the equation of the section of the surface S by the plane z = p is

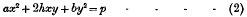
Now for rolling of this sort it is clear that the successive axes of rotation during a short time will be generators parallel to OX of a cylindrical surface which will touch S in the neighbourhood of O.