Article contents
Multiparameter spectral theory and Taylor's joint spectrum in Hilbert space
Published online by Cambridge University Press: 20 January 2009
Extract
Let n≧1 be an integer and suppose that for each i= 1,…,n, we have a Hilbert space Hi and a set of bounded linear operators Ti, Vij:Hi→Hi, j=1,…,n. We define the system of operators
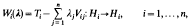
where λ=(λ1,…,λn)∈ℂn. Coupled systems of the form (1.1) are called multiparameter systems and the spectral theory of such systems has been studied in many recent papers. Most of the literature on multiparameter theory deals with the case where the operators Ti and Vij are self-adjoint (see [14]). The non self-adjoint case, which has received relatively little attention, is discussed in [12] and [13].
- Type
- Research Article
- Information
- Proceedings of the Edinburgh Mathematical Society , Volume 31 , Issue 1 , February 1988 , pp. 127 - 144
- Copyright
- Copyright © Edinburgh Mathematical Society 1988
References
REFERENCES
- 4
- Cited by


