No CrossRef data available.
Article contents
Invariants of two quaternary quadrics
Published online by Cambridge University Press: 20 January 2009
Extract
Following the papers by H. W. Turnbull and J. Williamson, I have verified that the 122 forms, of the system of two quaternary quadrics 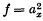 and
and  are actually irreducible. The original 1917 system contained 125 forms, which Williamson reduced by three. The present verification shews that no further reduction is possible. The proof was carried out as follows. I first constructed the whole system in canonical form with
are actually irreducible. The original 1917 system contained 125 forms, which Williamson reduced by three. The present verification shews that no further reduction is possible. The proof was carried out as follows. I first constructed the whole system in canonical form with  and
and  for the two quadrics, and then listed the degrees in the coefficients and variables u, p, x of these concomitants. I next made Diophantine equations between these degrees for testing the supposed reducibility and found them to be impossible, except for Williamson's reduced forms.
for the two quadrics, and then listed the degrees in the coefficients and variables u, p, x of these concomitants. I next made Diophantine equations between these degrees for testing the supposed reducibility and found them to be impossible, except for Williamson's reduced forms.
- Type
- Research Article
- Information
- Copyright
- Copyright © Edinburgh Mathematical Society 1936
References
page 41 note 1 Proc. London Math. Soc. (2) 18 (1917) 69–94.Google Scholar
page 41 note 2 Journal London Math. Soc. 4 (1929) 182–183.Google Scholar


