Hostname: page-component-f554764f5-wjqwx Total loading time: 0 Render date: 2025-04-10T21:24:40.398Z Has data issue: false hasContentIssue false
  • English
  • Français

Integrals involving Bessel and Legendre functions

Published online by Cambridge University Press:  20 January 2009

J. S. Lowndes
J. S. Lowndes
Affiliation:
Department of Mathematics, Uiversity of Strathclyde, Glasgow
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Felsen (1) has shown that when a plane wave is incident along the axis of a rigid cone of narrow apex angle an approximate expression for the scattered wave involves an integral of the form

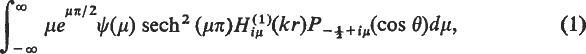
where 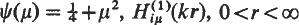is a Bessel function of the third kind, k a constant and 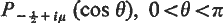, is the Legendre (conical) function of the first kind.

Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 14 , Issue 4 , December 1965 , pp. 269 - 272
Copyright
Copyright © Edinburgh Mathematical Society 1965

References

REFERENCES

(1) Felsen, L. B.Trans. IRE-PGAP, 5 (1957), 121–129.Google Scholar
(2) Erdélyi, A. et al. , Higher Transcendental Functions, (a) Vol. 1, (b) Vol. 2 (McGraw-Hill, 1953).Google Scholar
(3) Lowndes, J. S.SIAM Review, 3, 2 (1961), 162–164.Google Scholar